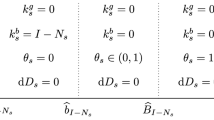Dynamic Pricing of Credit Cards and the Effects of Regulation

Abstract

We construct a two-period model of revolving credit with asymmetric information and adverse selection. In the second period, lenders exploit an informational advantage with respect to their own customers. Those rents stimulate competition for customers in the first period. The informational advantage the current lender enjoys relative to its competitors determines interest rates, credit supply, and switching behavior. We evaluate the consequences of limiting the repricing of existing balances as implemented by recent legislation. Such restrictions increase deadweight losses and reduce ex ante consumer surplus. The model suggests novel approaches to identify empirically the effects of this law. We find the pattern of changes to interest rates and balance transfer activity before and after the CARD Act are consistent with the testable implications of the model.

Appendices

Appendix A: Proofs of Lemmas and Propositions

1.1 A.1 Proof of Lemma 1

The first-order condition of (4.3) with respect to b₁, assuming that second-period rates for new borrowing do not depend on b₁, is⁵⁷

$$\begin{array}{@{}rcl@{}} &&u^{\prime }\left(b_{1}\right) -(1+r_{1}) \bar{p}-\delta\left(1-\bar{p}\right) (1+r_{1}) \left[\left(\mu p_{H}\left(1+R^{o}_{j2}\left(H\right) \right)+ (1-\mu)p_{L}\left(1+R^{o}_{j2}\left(L\right) \right)\right)\right]\\ &-&\delta(1-\overline{p})b_{1}(1+r_{1})\left(\mu p_{H}\frac{\partial R^{o}_{j2}(H)}{\partial b_{1}}+(1-\mu)p_{L}\frac{\partial R^{o}_{j2}(L)}{\partial b_{1}}\right)=0\text{.} \end{array}$$

(A.1)

Let $b_{1}^{\ast }\left (r_{1}\right )$ denote the size of the first-period loan. The constrained utility maximization problem can be stated as follows:

$$\begin{array}{@{}rcl@{}} &&\max\limits_{\{r^{n}_{j2},r^{o}_{j2}\}} U_{2}=u\left(b^{*}_{2}\right)-\left(1+r^{n}_{j2}\left(i\right) \right)b^{*}_{2}p_{i}-\left(1+r^{o}_{j2}\left(i\right) \right)\left(b^{*}_{1}\left(1+r_{1} \right) \right) p_{i}\\ &&\text{subject to:}\\ &&\left((r^{n}_{j2}(i)-\overline{r})p_{i}-(1-p_{i})(1+\overline{r})\right)b_{2}^{\ast}+\left((r^{o}_{j2}(i)-\overline{r})p_{i}- (1-p_{i})(1+\overline{r})\right)b^{*}_{1}(1+r_{1})=k. \end{array}$$

The (absolute) marginal rate of substitution between old and new interest rates, $dr^{o}_{j2}/dr^{n}_{j2}$, using the envelope theorem, holding expected utility constant (indifference curve) is

$$\frac{b^{*}_{2}}{b_{1}(1+r_{1})},$$

while holding the expected profits constant (iso-profit curve) is

$$\frac{p_{i}b_{2}^{*}+((r^{n}_{j2}(i)-\overline{r})p_{i}-(1-p_{i})(1+\overline{r}))\frac{\partial b_{2}^{*}}{\partial r_{j2}^{n}}}{p_{i}b^{*}_{1}(1+r_{1})}.$$

It can be easily calculated, using (4.2), that $\partial b^{*}_{2}/\partial r^{n}_{j2}<0$. The two marginal rates of substitution become equal at $r^{n}_{j2}(i)=(1+\overline {r}-p_{i})/p_{i}$. This is the one-period break-even interest rate. The unique tangency is optimal because when $r^{n}_{j2}(i)<(1+\overline {r}-p_{i})/p_{i}$ the iso-profit curve has a steeper slope than the indifference curve and when $r^{n}_{j2}(i)>(1+\overline {r}-p_{i})/p_{i}$ the slope of the iso-profit is flatter. These imply that any movement away from the tangency, on the iso-profit, will only lower the borrower’s expected utility. The optimal rate on old debt, $r_{j2}^{o}(i)$, is derived from the iso-profit constraint. Therefore, the solution to the previous constrained maximization problem is

$$r^{n}_{j2}(i)=\frac{1+\overline{r}-p_{i}}{p_{i}}\text{ and } r^{o}_{j2}(i)=\frac{b^{*}_{1}(1+r_{1})(1+\overline{r}-p_{i})+k}{p_{i}b^{*}_{1}(1+r_{1})}.$$

1.2 A.2 Derivation of probabilities

Using Bayes’ rule, with the understanding that μ is a function of whether d = 0 or d≠ 0, as given by (3.1), but in the expressions below such a dependence is suppressed, we derive the following conditional probabilities

$$\Pr(H|h)=\frac{(1+\phi)\mu}{(1+2\mu\phi-\phi)} \text{ and } \Pr(L|\ell)=\frac{(1+\phi)(1-\mu)}{(1-2\mu\phi+\phi)}.$$

(A.2)

The expected repayment probability, attached by an outsider who has received signal s = h, is

$$p(h)\equiv p_{H}\Pr(H|h)+p_{L}\Pr(L|h)=p_{H}\frac{(1+\phi)\mu}{(1+2\mu\phi-\phi)}+p_{L}\frac{(1-\phi)(1-\mu)}{(1+2\mu\phi-\phi)},$$

(A.3)

while if the signal the outsider has received is ℓ the expected probability is

$$p(\ell)\equiv p_{H}\Pr(H|\ell)+p_{L}\Pr(L|\ell)=p_{H}\frac{(1-\phi)\mu}{(1-2\mu\phi+\phi)}+p_{L}\frac{(1+\phi)(1-\mu)}{(1-2\mu\phi+\phi)}.$$

(A.4)

1.3 A.3 Proof of Proposition 1

We assume that the inside lender moves first and commits to the rate policy. Outsiders observe the insider’s policy but not the actual offer. The competitive fringe moves second, as in Sharpe (1990). In what follows, we suppress the dependence of the interest rates on d = 0 or d≠ 0, but it should be clear from the context which one is the case. Recall that the belief about the fraction of high types in the second period μ depends on whether the borrower carries debt or not. This affects the repayment probabilities p(s) and the interest rates, whenever those depend on the outsiders’ signal.

Borrower carries debt. :

All lenders offer the efficient rate for new debt as given in Lemma 1. The rate on old debt simply transfers surplus. The insider offers $r^{n}_{I2}(i,s)=\hat {r}_{I2}(i)$ for new debt, i = L,H and s = ℓ,h. When i = L, the insider also offers $r_{I2}^{o}(L,s)=\hat {r}_{I2}(L)$ for existing debt and for any s, making zero expected profits. The outsiders also offer $r_{O2}^{n}(s)=r_{O2}^{o}(s)=\hat {r}_{I2}(L)$ for old and new debt, regardless of the signal they receive. When i = H, the insider’s offer for existing debt is the one that yields utility to the borrower equal to the utility the borrower would receive had the outsiders offered their break-even rates $\hat {r}_{O2}(s)$, s = ℓ,h, for new and old debt, that is

$$\begin{array}{@{}rcl@{}} r_{I2}^{o}(H,s)&=&\frac{1+\overline{r}-p(s)}{p(s)}\\ &&+\frac{(u(b^{*}_{2}(r_{I2}^{n}(H,s)))-u(b^{*}_{2}(\hat{r}_{O2}(s))))p(s)- (1+\overline{r})(b^{*}_{2}(r_{I2}^{n}(H,s))p(s)-b^{*}_{2}(\hat{r}_{O2}(s))p_{H})}{p(s)p_{H}b_{1}^{*}(r_{1})(1+r_{1})}, \end{array}$$

where $b^{*}_{2}(r_{j2}^{n})$ is the amount the lender borrows in period 2 (new debt) from lender j = I,O.

Since the rate of the insider on new debt is lower than the rate the outsiders offer, the second-period expected utility of the high-type borrower, from new borrowing only, is higher when he borrows from the insider than the outsider

$$u(b^{*}_{2}(r_{I2}^{n}(H,s)))-(1+\overline{r})b^{*}_{2}(r_{I2}^{n}(H,s))\geq u(b^{*}_{2}(\hat{r}_{O2}(s)))-(1+\overline{r})\frac{p_{H}b^{*}_{2}(\hat{r}_{O2}(s))}{p(s)}.$$

The above inequality implies that the second term in the RHS of (4.10) is positive. Hence, $r_{I2}^{o}(H,s)\geq \hat {r}_{O2}(s)$, s = ℓ,h. Given the strategy of the insider, the outsider has no incentive to deviate. To steal the high-type borrower from the insider, it must offer rates lower than $\hat {r}_{O2}(s)$. This strategy will attract both types of borrowers, but the outsider’s expected profits are negative. If the outsider’s rates are higher than $\hat {r}_{O2}(s)$, it makes a loan to the borrower only in the event the borrower is of low quality. But in this case, expected profit is also negative. Given the response of the competitive fringe, the insider’s strategy is optimal. Profits will not increase if the rate offered to the low type changes; if it goes down, profits become negative, and if it goes up, the competitive fringe can make a better and profitable offer. Moreover, if the rates offered to the high type decrease, then profits will decrease (the competitive fringe will not match the offers since expected profits for them conditional on the signal are negative), while if the rates increase the competitive fringe will profitably undercut them.

Borrower carries no debt. :

The insider offers $r_{I2}(L,s)=\hat {r}_{I2}(L)$, when i = L and regardless of the outsiders’ signal. The outsiders also offer $r_{O2}(s)=\hat {r}_{I2}(L)$, no matter what signal they receive. When i = H, and assuming that the monopoly interest rate for the inside lender when it lends to the high type is higher than $\hat {r}_{O2}(\ell )$, the insider offers $r_{I2}(H,s)=\hat {r}_{O2}(s)$, s = ℓ,h. Following a similar logic as in the previous case, no player has an incentive to deviate.

1.4 A.4 Proof of Lemma 2

We totally differentiate (A.1) with respect to b₁ and r₁, by noting that equilibrium interest rates in period 2 for the low type are independent of b₁ and r₁, while for the high type the rate for old debt is given by (4.10). We need to compute the effect of b₁ on the second-period rate for the old debt the high type will pay. Using (4.10) we obtain

$$\frac{dr^{o}_{I2}(H,s)}{db_{1}}=-\frac{(u(b^{*}_{2}(r_{I2}^{n}(H,s)))-u(b^{*}_{2}(\hat{r}_{O2}(s))))p(s)- (1+\overline{r})(b^{*}_{2}(r_{I2}^{n}(H,s))p(s)-b^{*}_{2}(\hat{r}_{O2}(s))p_{H})}{p(s)p_{H}(b_{1}^{*}(r_{1}))^{2}(1+r_{1})}.$$

(A.5)

From the proof of Proposition 1, the above term is negative. Let

$$A(s)\equiv (u(b^{*}_{2}(r_{I2}^{n}(H,s)))-u(b^{*}_{2}(\hat{r}_{O2}(s))))p(s)- (1+\overline{r})(b^{*}_{2}(r_{I2}^{n}(H,s))p(s)-b^{*}_{2}(\hat{r}_{O2}(s))p_{H}).$$

We substitute (A.5), (4.10) and the equilibrium second-period rate for existing debt for the L types into the borrower’s first-order condition for b₁, (A.1), also noting that the secondperiod rate on old debt for the low type is not a function of b₁. This yields

$$\begin{array}{@{}rcl@{}} &&u^{\prime }\left(b_{1}\right) -(1+r_{1}) \bar{p}\\ &-&\delta\left(1-\bar{p}\right) (1+r_{1})\Big(\mu p_{H}\Big\{\frac{1+\phi}{2}\left(1+\frac{1+\overline{r}-p(h)}{p(h)}+\frac{A(h)}{p(h)p_{H}b_{1}^{*}(r_{1})(1+r_{1})}\right)+\\ &&\frac{1-\phi}{2}\left(1+\frac{1+\overline{r}-p(\ell)}{p(\ell)}+\frac{A(\ell)}{p(\ell)p_{H}b_{1}^{*}(r_{1})(1+r_{1})}\right)\Big\}+(1-\mu)p_{L}\left(1+\frac{1+\bar{r}-p_{L}}{p_{L}} \right)\Big)\\ &+&\delta(1-\overline{p})b_{1}(1+r_{1})\left(\mu p_{H}\left\{\frac{1+\phi}{2}\frac{A(h)}{p(h)p_{H}(b_{1}^{*}(r_{1}))^{2}(1+r_{1})}+\frac{1-\phi}{2}\frac{A(\ell)}{p(\ell)p_{H}(b_{1}^{*}(r_{1}))^{2}(1+r_{1})}\right\}\right)=0\Rightarrow\\ &&u^{\prime }\left(b_{1}\right) -(1+r_{1}) \bar{p}-\delta\left(1-\bar{p}\right)(1+r_{1})(1+\bar{r})\left[\mu\underbrace{p_{H}\left\{\frac{1+\phi}{2p(h)}+\frac{1-\phi}{2p(\ell)}\right\}}_{X(\phi)}+(1-\mu)\right]=0. \end{array}$$

(A.6)

As r₁ affects the first-order condition negatively and the utility function u(⋅) is concave, it follows easily that $db^{*}_{1}/dr_{1}<0$.

The X(ϕ) term in (A.6) captures the inside lender’s informational advantage, and it ranges monotonically from 1 when ϕ = 1 (no informational advantage) to $p_{H}/\bar {p}>1$ when ϕ = 0 (maximum informational advantage). Finally, it is easy to show that the term in the brackets in (A.6) is inverse U-shaped in μ, suggesting that the highest uncertainty, and hence market power for the inside lenders, is for intermediate μ’s.

1.5 A.5 Proof of Proposition 2

First, we account for the outsider signal uncertainty. Using the second-period equilibrium interest rates (see Proposition 1), the expected profits of the insider in the second period, if the borrower is of high type, using (4.4) and (4.5), are

$$E\pi_{I2}(H;d\neq 0)=\pi_{I2}(d\neq 0,h)\frac{1+\phi}{2}+\pi_{I2}(d\neq 0,\ell)\frac{1-\phi}{2},$$

where, using the rates from Proposition 1

$$\begin{array}{@{}rcl@{}} &&\pi_{I2}(d\neq 0,s)=\frac{(p_{H}-p(s))(1+\overline{r})b^{*}_{1}(r_{1})(1+r_{1})}{p(s)}\\ &+&\frac{(u(b^{*}_{2}(r_{I2}^{n}(H,s)))-u(b^{*}_{2}(\hat{r}_{O2}(s))))p(s)-(1+\overline{r})(b^{*}_{2}(r_{I2}^{n}(H,s))p(s)-b^{*}_{2}(\hat{r}_{O2}(s))p_{H})}{p(s)}\geq 0 \end{array}$$

(A.7)

and⁵⁸

$$\begin{array}{@{}rcl@{}} E\pi_{I2}(H;d=0)&=&\pi_{I2}(d=0,h)\frac{1+\phi}{2}+\pi_{I2}(d=0,\ell)\frac{1-\phi}{2}\\ &=&\frac{1+\phi}{2}\left(\left(\hat{r}_{O2}(h)-\bar{r}\right) p_{H}-\left(1-p_{H}\right)\left(1+\bar{r}\right)\right)b^{*}_{2}(\hat{r}_{O2}(h))\\ &&+\frac{1-\phi}{2}\left(\left(\hat{r}_{O2}(l)-\bar{r}\right) p_{H}-\left(1-p_{H}\right)\left(1+\bar{r}\right)\right)b^{*}_{2}(\hat{r}_{O2}(l))\\ &=&\frac{(1-\mu)(p_{H}-p_{L})(1+\overline{r})(1-\phi^{2})b^{*}_{2}(\hat{r}_{O2}(h))}{2\left(p_{H}\mu(1+\phi)+p_{L}(1-\mu)(1-\phi)\right)}\\ &&+\frac{(1-\mu)(p_{H}-p_{L})(1+\overline{r})(1-\phi^{2})b^{*}_{2}(\hat{r}_{O2}(\ell))}{2\left(p_{H}\mu(1-\phi)+p_{L}(1-\mu)(1+\phi)\right)}\geq 0. \end{array}$$

(A.8)

When the borrower is of the low type (high risk), profits are Eπ_I2(L) = 0, regardless of the level of debt.

Second, we account for the uncertainty over the true probability p_i and the level of debt d₁. Therefore, second-period expected profits are given by

$$E\pi_{I2}=\mu\left[p_{H} E\pi_{I2}(H;d=0)+(1-p_{H})E\pi_{I2}(H;d\neq 0)\right]\geq 0.$$

(A.9)

The representative lender’s expected profits at the beginning of period 1 are

$$E\pi=\left((r_{1}-\overline{r})\overline{p}-(1-\overline{p})(1+\overline{r})\right)b^{\ast}_{1}+\delta E\pi_{I2}.$$

Because borrowers are ex-ante identical and lenders are homogeneous, competition in the first period will drive expected profits down to zero. The first period equilibrium interest rate $r_{1}^{\ast }$ must satisfy Eπ = 0, which yields

$$r^{*}_{1}=\frac{1+\overline{r}-\overline{p}}{\overline{p}}-\frac{\delta E\pi_{I2}}{\overline{p}b^{*}_{1}(r^{*}_{1})}.$$

(A.10)

Note that: i) Eπ_I2(H; d = 0) does not depend on r₁, ii) Eπ_I2(H; d≠ 0) is increasing in r₁ and iii) $db^{*}_{1}(r_{1})/dr_{1}<0$ (see Lemma 2). Therefore, the term $\delta E\pi _{I2}/(\overline {p}b^{*}_{1}(r_{1}))$ is increasing in r₁. So, the right-hand side of (A.10) is decreasing in r₁. This implies that there exists a unique $r^{*}_{1}$ (not necessarily positive) that makes Eπ zero.

Next, we examine the effect of ϕ on $r^{*}_{1}$. It is clear that as ϕ increases, the informational advantage of the insider over the outsiders diminishes and second-period expected profits decrease. Also, from (A.6), it follows that b₁ increases as ϕ increases, holding r₁ fixed. This implies that as ϕ increases, the RHS of (A.10) increases as well and hence $r_{1}^{*}$ increases.

1.6 A.6 Proof of Proposition 4

Second-period expected profits for the inside lender will be positive. Therefore, first-period rate $r^{reg}_{1}$ must be less that $(1+\overline {r}-\overline {p})/\overline {p}$; otherwise first-period profits are also positive, which contradicts the fact that overall expected profits must be zero in equilibrium. Hence, the following ranking holds

$$r^{reg}_{1}<\frac{1+\overline{r}-\overline{p}}{\overline{p}}<\frac{1+\overline{r}-p(\ell)}{p(\ell)}<\frac{1+\overline{r}-p_{L}}{p_{L}}.$$

(A.11)

We use $\tilde {r}$ to denote the second-period rates under regulation. In what follows, we assume that the borrower carries debt into the second period. Otherwise, the regulation does not affect the equilibrium described in Propositions 1 and 2.

Low type. :

From (A.11), the break-even rate for the low type, $\hat {r}_{I2}(L)\equiv (1+\overline {r}-p_{L})/p_{L}$, is higher than the first-period rate, $r^{reg}_{1}$. The inside lender is constrained by the regulation not to raise the second-period rate for existing debt from $r^{reg}_{1}$. Thus, in equilibrium, the insider offers $\tilde {r}^{o}_{I2}(L,s)=r_{1}^{reg}$ for existing debt and

$$\tilde{r}^{n}_{I2}(L,s)=\frac{1+\overline{r}-p_{L}}{p_{L}}+\frac{b_{1}(1+r_{1}^{reg})\left(1+\overline{r}-p_{L}(1+r_{1}^{reg})\right)}{p_{L}b_{2}},$$

(A.12)

s = ℓ,h, for new debt so that expected profits are zero.⁵⁹ Denote the utility to the borrower by U₂(L). The outside lenders have an advantage over the insider and can offer to the borrower strictly higher utility than U₂(L), while their expected profits are zero. This follows from Lemma 1, which demonstrates that the rate for new debt that maximizes the surplus in the lender-borrower relationship is $\hat {r}_{I2}(L)\equiv (1+\overline {r}-p_{L})/p_{L}$. The rate on existing debt simply transfers surplus between the two parties. Due to regulation, the insider offers a rate on new debt that is higher than the efficient rate, $\tilde {r}^{n}_{I2}(L,s)>\hat {r}_{I2}(L)$. In equilibrium, the outsiders offer $\tilde {r}^{n}_{O2}(s)=\tilde {r}^{o}_{O2}(s)=\hat {r}_{I2}(L)$, s = ℓ,h, which provides to the borrower strictly higher utility than the insider⁶⁰ (but the same as in the unregulated case) while, due to Bertrand-type competition, make zero expected profits.

In sum, regulation has no effect on the second-period equilibrium rates offered to the low type (same as in Proposition 1). Also, all lenders make zero expected profits, as in the unregulated case. The only effect regulation has is that it induces the low type to switch lenders. The low type, however, is as well off as he was prior to regulation (assuming switching is costless). This is true as long as no high type switches lenders, so the outsiders are certain that they are lending to the low types.

High type. :

The rate for existing debt prior to regulation is given by (4.10). It follows from (A.11) that regulation is certainly binding for the insider when i = H and s = ℓ, that is, $r_{1}^{reg}$ is below $r^{o}_{I2}(H,\ell )$, the rate the insider offers under no regulation to the H type when the marker’s signal is ℓ. It may or may not be below $r^{o}_{I2}(H,h)$, depending on the informational asymmetry ϕ.⁶¹

We assume that $r_{1}^{reg}>\hat {r}_{I2}(H)\equiv (1+\bar {r}-p_{H})/p_{H}$, which guarantees strictly positive expected profits of the inside lenders when they lend to the high types. Hence, no high-type borrower switches lenders. This assumption is confirmed by the numerical example of Section B and is certainly true for high ϕ. In what follows, we assume that $r_{1}^{reg}<r^{o}_{I2}(H,h)<r^{o}_{I2}(H,\ell )$, but the arguments do not change if $r_{1}^{reg}>r^{o}_{I2}(H,h)$.

In equilibrium, the insider makes interest rate offers to the borrower for existing and new debt so that, if the outsiders were to match the borrower’s utility, their profits would be zero (the same as when they lend to the low type exclusively). Since the outsiders face no constraints, a deviator’s offer for new debt would be equal to $\hat {r}_{O2}(s)$, s = ℓ,h and for old debt greater than or equal to $\hat {r}_{O2}(s)$, s = ℓ,h. To ensure that there does not exist a profitable deviation on part of the outsiders, when regulation is binding, the rate the insider offers for new debt should match the expected utility the borrower obtains if he borrows from an outsider instead at the zero-profit-for-an-outsider deviation rates $\hat {r}_{O2}(s)$, s = ℓ,h, and it is given (implicitly) by⁶²

$$\begin{array}{@{}rcl@{}} \tilde{r}_{I2}^{n}(H,s)&=&-1+\left(\frac{(1+\hat{r}_{O2}(s))(1+r_{1}^{reg})}{b^{*}_{2}(\tilde{r}_{I2}^{n}(H,s))}-\frac{(1+r_{1}^{reg})^{2}}{b^{*}_{2}(\tilde{r}_{I2}^{n}(H,s))}\right)b^{*}_{1}\\ &&+\frac{u(b^{*}_{2}(\tilde{r}_{I2}^{n}(H,s)))- u(b^{*}_{2}(\hat{r}_{O2}(s)))}{b^{*}_{2}(\tilde{r}_{I2}^{n}(H,s))p_{H}}+\frac{b^{*}_{2}(\hat{r}_{O2}(s))(1+\hat{r}_{O2})}{b^{*}_{2}(\tilde{r}_{I2}^{n}(H,s))p(H)}. \end{array}$$

(A.13)

Due to the insider’s inability to raise the rate on old debt from $r_{1}^{reg}$, the rate on new debt can be higher than the efficient rate.⁶³ Given that the inside lender can no longer offer the efficient rate for new debt and the borrower’s utility stays the same between the two regimes (determined by the outsiders’ potential offers), it follows that the inside lender is worse off under the regulation in the second period, that is, $E\pi _{I2}^{reg}(H;d\neq 0)\leq E\pi _{I2}(H;d\neq 0)$, where Eπ_I2(H; d≠ 0) was derived when we analyzed the unregulated equilibrium.

Combining the case of positive second-period debt we just examined with that of no debt, the expected profits in the second period for the inside lender are

$$E\pi_{I2}^{reg}=\mu\left[p_{H} E\pi_{I2}(H;d=0)+(1-p_{H})E\pi_{I2}^{reg}(H;d\neq 0)\right]>0.$$

Analogous to the unregulated case, see (A.10), the first-period equilibrium rate must satisfy

$$r^{reg}_{1}=\frac{1+\overline{r}-\overline{p}}{\overline{p}}-\frac{\delta E\pi_{I2}^{reg}}{\overline{p}b^{*}_{1}(r^{reg}_{1})}.$$

(A.14)

The $\delta E\pi _{I2}^{reg}/(\overline {p}b_{1}^{\ast }(r^{reg}_{1}))$ term decreases as $r_{1}^{reg}$ decreases. As we argued previously, the numerator decreases, while the denominator increases, $db^{*}_{1}(r_{1}^{reg})/dr_{1}^{reg}<0$, as we show next. First, we differentiate (4.3) with respect to b₁ taking into account that b₁ affects the rates for new borrowing in period 2, as it is demonstrated by (A.13). This yields

$$\begin{array}{@{}rcl@{}} &&u^{\prime }\left(b_{1}\right) -(1+r^{reg}_{1}) \bar{p}-\delta\left(1-\bar{p}\right) (1+r^{reg}_{1})\left[\left(\mu p_{H}\left(1+R^{o}_{j2}\left(H\right) \right)+ (1-\mu)p_{L}\left(1+R^{o}_{j2}\left(L\right) \right)\right)\right]\\ &-&\delta(1-\overline{p})\left(\mu p_{H}\frac{\partial R^{n}_{j2}(H)}{\partial b_{1}}b^{*}_{2}(p_{H})\right)=0. \end{array}$$

Using the equilibrium rates and how (A.13) responds to changes in b₁, we obtain

$$\begin{array}{@{}rcl@{}} &&u^{\prime }\left(b_{1}\right) -(1+r^{reg}_{1}) \bar{p}-\delta\left(1-\bar{p}\right) \left[\left(\mu p_{H}(1+r_{1}^{reg})^{2}\right)+ (1-\mu)(1+\overline{r})(1+r_{1}^{reg})\right]\\ &-&\delta(1-\overline{p})\mu p_{H}\left(\frac{1+\phi}{2}\left(\frac{(1+\overline{r})(1+r_{1}^{reg})}{b^{*}_{2}(r_{1}^{reg})p(h)}-\frac{(1+r_{1}^{reg})^{2}}{b^{*}_{2}(r_{1}^{reg})}\right)b^{*}_{2}(r_{1}^{reg})\right)\\ &-&\delta(1-\overline{p})\mu p_{H}\left(\frac{1-\phi}{2} \left(\frac{(1+\overline{r})(1+r_{1}^{reg})}{b^{*}_{2}(r_{1}^{reg})p(\ell)}-\frac{(1+r_{1}^{reg})^{2}}{b^{*}_{2}(r_{1}^{reg})}\right)b^{*}_{2}(r_{1}^{reg})\right)=0\Rightarrow\\ &&u^{\prime }\left(b_{1}\right) -(1+r^{reg}_{1}) \bar{p}\\ &-&\delta\left(1-\bar{p}\right)(1+\overline{r})(1+r_{1}^{reg})\left(\frac{1+\phi}{2}\frac{\mu p_{H}}{p(h)}+\frac{1-\phi}{2}\frac{\mu p_{H}}{p(\ell)}+ (1-\mu)\right)=0. \end{array}$$

(A.15)

Using (A.15), we can derive $db^{*}_{1}/dr_{1}^{reg}$

$$\frac{db^{*}_{1}}{dr^{reg}_{1}}=\frac{\bar{p}+\delta\left(1-\bar{p}\right)(1+\overline{r})\left(\frac{1+\phi}{2}\frac{\mu p_{H}}{p(h)}+\frac{1-\phi}{2}\frac{\mu p_{H}}{p(\ell)}\right)}{u^{\prime\prime}(b_{1})}<0.$$

Thus, the RHS of (A.14) is increasing as $r_{1}^{reg}$ decreases. Therefore, there exists a unique $r^{reg}_{1}$ that satisfies the equilibrium condition (A.14).

How does the regulated equilibrium first-period rate compare with that in the unregulated equilibrium? Because $E\pi _{I2}\geq E\pi _{I2}^{reg}$, the equilibrium interest rate under regulation is higher than the first-period rate under no regulation.

1.7 A.7 Proof of Proposition 5

The first-order condition of (C.1) with respect to b₂ is

$$\begin{array}{@{}rcl@{}} &&u^{\prime}(b_{2})-p_{i}C^{nd}f(C^{nd})\frac{dC^{nd}}{db_{2}}+p_{i}C^{nd}f(C^{nd})\frac{dC^{nd}}{db_{2}}-p_{i}\frac{dC^{nd}}{db_{2}}(1-F(C^{nd}))=0\\ &&\Rightarrow u^{\prime}(b_{2})-p_{i}(1+r^{n/nd})(1-F(C^{nd}))=0. \end{array}$$

(A.16)

Let $b_{2}^{nd}(r^{n/nd})$ denote the solution to the borrower’s optimization problem. The effect of the interest rate on the amount borrowed is (where the superscript of r is suppressed)

$$\frac{db^{nd}_{2}}{dr}=\frac{p_{i}\left((1-F(C^{nd}))-(1+r)f(C^{nd})b_{2}\right)}{u^{\prime\prime}(b_{2})+p_{i}(1+r)^{2}f(C^{nd})}.$$

The denominator must be negative (from the second order condition). The numerator is positive if and only if

$$b_{2}<\frac{1-F(C^{nd})}{(1+r)f(C^{nd})}.$$

(A.17)

If we assume that F(⋅) satisfies the monotone hazard rate (MHR) property, then the RHS of (A.17) is decreasing in b₂, because C^nd is increasing in b₂. When b₂ = 0, the above inequality is satisfied, but for some b₂, it may not. At that point, demand for credit will become upward sloping. So there may exist a b₂, denoted by $\overline {b}$ that satisfies the above expression with equality, such that beyond this level db₂/dr > 0. We want to rule out this possibility. This is what we do next.

From the first-order condition of the borrower’s problem, see (A.16), the following expression must be satisfied

$$p_{i}=\frac{u^{\prime}(b_{2})}{(1+r)(1-F(C^{nd}))}.$$

(A.18)

We substitute the above into the borrower’s second-order condition

$$\begin{array}{@{}rcl@{}} &&u^{\prime\prime}(b_{2})+\frac{u^{\prime}(b_{2})}{(1+r)(1-F(C^{nd}))}(1+r)^{2}f(C^{nd})<0\Rightarrow u^{\prime\prime}(b_{2})+\frac{u^{\prime}(b_{2})(1+r)f(C^{nd})}{(1-F(C^{nd}))}<0\\ &&\Rightarrow-\frac{u^{\prime\prime}(b_{2})}{u^{\prime}(b_{2})}>\frac{(1+r)f(C^{nd})}{1-F(C^{nd})}. \end{array}$$

(A.19)

If the above condition is satisfied, then the borrower’s utility function is concave at the point where the first-order condition is satisfied. This suggests that for every interest rate there is a unique (interior) level of borrowing that maximizes utility. The LHS of (A.19) is the Arrow-Pratt coefficient of absolute risk aversion, which – given our assumption – is decreasing in b₂. On the other hand, the RHS is increasing in b₂ given that F(⋅) satisfies the MHR property. Furthermore, we assume that the inequality (A.19) is strictly satisfied at b₂ = 0.⁶⁴ It follows that there must exist a unique b₂ > 0 that satisfies (A.19) with equality. For b₂’s higher than this threshold, whenever the first-order condition is satisfied, the borrower’s utility function is convex. Moreover, from that point on, the expected utility of the borrower is monotonically increasing in b₂. The probability of default is very high and the borrower chooses to borrow a lot knowing that default is almost certain. In sum, the borrower’s expected utility may exhibit a sideways “S”-shape with a unique interior maximum.⁶⁵

Let $\overline {B}$ be the level of borrowing that attains the same expected utility as the interior maximum, see Fig. 10. The lender, to prevent the borrower from borrowing too much and then defaulting, will impose a credit limit somewhere to the right of the point that satisfies the first-order condition for the interior maximum and to the left of $\overline {B}$. If ${\Gamma }\leq (1+r)\overline {B}$, then the probability of default is 1 when borrowing exceeds $\overline {B}$. Hence, a lender will never find it optimal to set a credit limit that exceeds $\overline {B}$, as this will induce a certain default. Therefore, the first-order condition as given by (A.16) characterizes the borrower’s decision. When the probability of default is exogenously given, as we have assumed up to this section, a credit limit is not needed, since the borrower’s utility assumes a unique interior maximum.

Further, we assume that the Arrow-Pratt degree of relative risk aversion for u(⋅) does not exceed one

$$-\frac{u^{\prime\prime}(b_{2})b_{2}}{u^{\prime}(b_{2})}\leq 1.$$

This, together with (A.19), imply

$$\frac{1}{b_{2}}\geq-\frac{u^{\prime\prime}(b_{2})}{u^{\prime}(b_{2})}>\frac{(1+r)f(C^{nd})}{1-F(C^{nd})}.$$

Therefore, (A.17) is satisfied. Demand for credit is downward sloping, when the solution to a borrower’s utility maximization problem is interior (i.e., credit limit is not binding). Moreover, given our assumption ${\Gamma }\leq (1+r)\overline {B}$, the credit limit is not binding.

Now suppose the borrower carries debt from the first period. The first-order condition of (C.2) with respect to b₂ is

$$\begin{array}{@{}rcl@{}} &&u^{\prime}(b_{2})-p_{i}C^{d}f(C^{d})\frac{dC^{d}}{db_{2}}+p_{i}C^{d}f(C^{nd})\frac{dC^{d}}{db_{2}}-p_{i}\frac{dC^{d}}{db_{2}}(1-F(C^{d}))=0\\ &&\Rightarrow u^{\prime}(b_{2})-p_{i}(1+r^{n/d})(1-F(C^{d}))=0. \end{array}$$

(A.20)

Let ${b_{2}^{d}}(r^{n/d},r^{o})$ denote the solution to the borrower’s optimization problem. It follows easily, by comparing (A.16) with (A.20), that ${b_{2}^{d}}\geq b_{2}^{nd}$ if the interest rates on new debt are the same. This is because default is more likely when the consumer carries debt, which makes borrowing in the second period cheaper. The effect of interest rates on the amount borrowed is

$$\begin{array}{@{}rcl@{}} \frac{d{b_{2}^{d}}}{dr^{n/d}}&=&\frac{p_{i}\left((1-F(C^{d}))-(1+r^{n/d})f(C^{d})b_{2}\right)}{u^{\prime\prime}(b_{2})+p_{i}(1+r^{n/d})^{2}f(C^{d})}\\ \frac{d{b_{2}^{d}}}{dr^{o}}&=&\frac{p_{i}\left((1-F(C^{d}))-(1+r^{n/d})f(C^{d})b_{1}(1+r_{1})\right)}{u^{\prime\prime}(b_{2})+p_{i}(1+r^{n})^{2}f(C^{d})}. \end{array}$$

(A.21)

Both r^n/d and r^o affect second-period borrowing. The rate on old debt affects new borrowing because it affects the default probability. As in the case of no debt, db₂/dr^n/d is negative provided that the borrower’s utility has an Arrow-Pratt degree of relative risk aversion is not greater than one. For db₂/dr^o to be negative, it must be that b₁(1 + r₁) is low enough.

1.8 A.8 Proof of Lemma 3

The break-even rates must (implicitly) satisfy⁶⁶

$$\hat{r}^{n/d}=\frac{1+\overline{r}-p_{i}(1-F(C^{d}))}{p_{i}(1-F(C^{d}))} \text{ and } \hat{r}^{o}=\frac{1+\overline{r}-p_{i}(1-F(C^{d}))}{p_{i}(1-F(C^{d}))}.$$

(A.22)

These rates resemble the break-even interest rates from the case of exogenous default (see (4.6)) but are modified to account for the probability of no default (1 − F(⋅)).

The first-order condition with respect to r^n/d is

$$\begin{array}{@{}rcl@{}} &&\frac{d{\pi^{d}_{2}}}{dr^{n/d}}=\\ &&p_{i}(1-F(C^{d}))b_{2}-p_{i}f(C^{d})\left((1+r^{n/d}){b_{2}^{d}}+(1+r^{o})(1+r_{1})b_{1}\right)\left(b_{2}+(1+r^{n/d})\frac{db_{2}}{dr^{n/d}}\right)\\ &+&p_{i}(1-F(C^{d}))(1+r^{n/d})\frac{db_{2}}{dr^{n/d}}-(1+\overline{r})\frac{db_{2}}{dr^{n/d}}=0. \end{array}$$

The first-order condition with respect to r^o is

$$\begin{array}{@{}rcl@{}} &&\frac{d\pi^{d}_2}{dr^{o}}=\\ &&p_i(1-F(C^d))(1+r_1)b_1-p_if(C^d)\left((1+r^{n/d})b_2^d+(1+r^o)(1+r_1)b_1\right)\left(b_1(1+r_1)+(1+r^{n/d})\frac{db_2}{dr^o}\right)\\ &+&p_i(1-F(C^d))(1+r^{n/d})\frac{db_2}{dr^o}-(1+\overline{r})\frac{db_2}{dr^o}=0. \end{array}$$

Let’s now turn to the borrower’s utility. Using (C.2), we can derive the (absolute) marginal rate of substitution between old and new interest rates, dr^o/drⁿ, using the envelope theorem, and holding expected utility constant (indifference curve). This yields

$$\frac{dr^{o}}{dr^{n/d}}=\frac{b^{*}_{2}}{b_{1}(1+r_{1})}.$$

The iso-profit of the lender has slope (using C^d ≡ (1 + r^n/d)b₂ + (1 + r^o)b₁(1 + r₁))

$$\begin{array}{@{}rcl@{}} &&\frac{dr^{o}}{dr^{n}}=\frac{d\pi/dr^{n/d}}{d\pi/dr^{o}}=\\ &&\frac{(p_{i}(1-F(C^{d}))-p_{i}f(C^{d})C^{d})b_{2}+\left(p_{i}(1-F(C^{d}))(1+r^{n/d})-(1+\overline{r})-p_{i}f(C^{d})C^{d}\right)\frac{db_{2}}{dr^{n/d}}}{(p_{i}(1-F(C^{d}))-p_{i}f(C^{d})C^{d})b_{1}(1+r_{1})+\left(p_{i}(1-F(C^{d}))(1+r^{/d}n)-(1+\overline{r})-p_{i}f(C^{d})C^{d}\right)\frac{db_{2}}{dr^{o}}}. \end{array}$$

If r^n/d satisfies

$$r^{n/d}=\frac{1+\overline{r}-p_{i}(1-F(C^{d}))}{p_{i}(1-F(C^{d}))}+\frac{f(C^{d})C^{d}}{1-F(C^{d})},$$

(A.23)

then the slope of the iso-profit equals the slope of the indifference curve. The interest rates associated with this tangency maximize borrower utility subject to a constant lender profit constraint. The idea is similar to the one used in the proof of Lemma 1 and uses the fact we state in Proposition 5 that demand for credit is downward sloping.

Observe that the rate implied by (A.23) is higher than the break-even rate for new borrowing implied by (A.22). Therefore, for the lender to break even, the rate on existing debt must decrease, suggesting that the efficient break-even rates must satisfy

$$\tilde{r}^{n/d}=\frac{1+\overline{r}-p_{i}(1-F(C^{d}))}{p_{i}(1-F(C^{d}))}+\frac{f(C^{d})C^{d}}{1-F(C^{d})} \text{ and } \tilde{r}^{o}<\frac{1+\overline{r}-p_{i}(1-F(C^{d}))}{p_{i}(1-F(C^{d}))}.$$

Appendix B: A Numerical Example

We assume a CRRA utility function $u(b_{t})=\frac {b_{t}^{1-\gamma }}{1-\gamma }$, t = 1, 2. We choose the parameter values so that the equilibrium interest rates are reasonably close to what is observed in real data. We set γ = 1/2, μ = 0.3, p_H = 0.95, p_L = 0.85, $\overline {r}=0.02$, and δ = 0.8.

Figure 5 depicts the unregulated equilibrium second- and first-period rates, see Propositions 1 and 2.

Fig. 5

Equilibrium second- and first-period interest rates as a function of the outsiders’ signal accuracy ϕ, the borrower’s type i = H,L, the outsiders’ signal s = h,ℓ, and the level of debt, d = 0 or d≠ 0

Figure 6 depicts the first- and second-period equilibrium and first-best borrowing. First-period equilibrium borrowing can be above or below what a social planner prefers, depending on the extent of the informational asymmetry. In the second period, while the social planner prefers smoothing of borrowing across types the market equilibrium does not deliver it. In addition, there is underborrowing relative to the first best. When ϕ = 1, and hence the inside lenders have no market power, the equilibrium borrowing in the second period is at the first-best levels,⁶⁷ but there is still inefficiency in period 1 borrowing. As we discuss in Section 4.3, this is because the social planner solution may imply negative profit for the lenders.

Fig. 6

Equilibrium (*) versus the first-best (fb) borrowing (b) in periods 1 and 2 as a function of the outsiders’ signal accuracy ϕ, the borrower’s type i = H,L, the outsiders’ signal s = h,ℓ and the level of debt, d = 0 or d≠ 0

Figure 7 plots the regulated first-period rate, $r_{1}^{reg}$, together with the equilibrium, $r_{1}^{\ast }$, and the first-best, $r_{1}^{fb}$, first-period rates, for all values of ϕ.

Fig. 7

The first-period rates: $r_{1}^{\ast }$ under no regulation, $r_{1}^{reg}$ under regulation and $r_{1}^{fb}=\frac {1+\overline {r}-\overline {p}}{\overline {p}}$, the first-best first-period rate. For ϕ less than (approximately) 0.81, the regulation is binding for the H type regardless of the outsiders’ signal, i.e., $r_{1}^{reg}<r_{I2}^{o}(H,h,d\neq 0)<r_{I2}^{o}(H,\ell ,d\neq 0)$. For ϕ > 0.81, $r_{1}^{reg}$ is higher than $r^{o}_{I2}(H,h,d\neq 0)$ and therefore the regulation does not bind for the H type when s = h. In this case, the inside lender switches to the unregulated equilibrium rates

Borrowers pay a higher first-period rate but a lower rate on existing borrowing carried over in period 2 under regulation than in the unregulated equilibrium. As Fig. 8 illustrates, the overall effect of regulation on first-period borrowing is negative. Less first-period borrowing can be social welfare enhancing or damaging depending on the value of ϕ. Second-period borrowing for the H types who carry debt under regulation falls below the first best, since the rate on new debt under regulation is higher than the efficient rate. Moreover, these rates, as it can be seen from (A.13), depend on s and therefore regulation also introduces variability in the borrowing of the H types in period 2. Finally, as we know, regulation increases the deadweight loss, see Fig. 9.

Fig. 8

Borrowers borrow less in the first period under regulation than no regulation

Fig. 9

Total welfare comparisons: Regulation exacerbates the inefficiency relative to the unregulated equilibrium. When there is no asymmetric information between the inside and the outside lenders, ϕ = 1, then total welfare is the same between the regulated and the unregulated equilibrium

Appendix C: Endogenous default / moral hazard and Switching costs

The purpose of this section is to demonstrate that our main results do not change qualitatively when we allow the borrower to decide whether to default or not, when his income is high, or when the borrowers incur a cost to switch lenders.

1.1 C.1 Endogenous default / moral hazard

In the presence of potential moral hazard, lenders impose non-binding credit limits and the equilibrium interest rate in the second period on new borrowing can be lower than the rate on existing debt. Given the complexity of the problem, we focus on the second period, taking first-period interest rate and amount borrowed as given.

1.1.1 C.2 Borrower’s problem

Borrower i in period 2, who borrows from lender j, chooses b₂ to maximize his second-period expected utility. After he chooses b₂ the cost of bankruptcy c is a random draw from a distribution F(c) on [0,Γ] with mean $\overline {c}$. We assume that F(⋅) satisfies the monotone hazard rate (MHR) property.⁶⁸ The borrower, after observing the cost of default and given that his income is not zero, decides whether to default or not. The second-period utility of the borrower is given as follows

$$\begin{array}{@{}rcl@{}} U_{2}=\left\{ \begin{array}{llll} u\left(b_{2}\right)-\left(1+r^{n/d} \right)b_{2}-\left(1+r^{o} \right)b_{1}\left(1+r_{1} \right)\text{,} & \text{ if } d=b_{1}(1+r_{1}) \text{ and no default} \\ u\left(b_{2}\right)-c\text{,} & \text{ if } d=b_{1}(1+r_{1}) \text{ and default} \\ u\left(b_{2}\right) -\left(1+r^{n/nd} \right) b_{2}\text{ ,} & \text{ if } d=0 \text{ and no default}\\ u\left(b_{2}\right) -c\text{ ,} & \text{ if } d=0 \text{ and default,} \end{array} \right. \end{array}$$

where n/d in the superscript of r stands for new (borrowing)/debt, while n/nd stands for new (borrowing)/ no debt. We assume that u(b₂) exhibits a (weakly) decreasing Arrow-Pratt degree of absolute risk aversion and has a degree of relative risk aversion that is less than one.⁶⁹

If the borrower carries debt from the first period, and assuming that his income realization is high, he chooses to default if and only if the cost of default is low, that is, c ≤ (1 + r^n/d)b₂ + (1 + r^o)b₁(1 + r₁), where r^o is the interest rate for old debt. Let C^d ≡ (1 + r^n/d)b₂ + (1 + r^o)b₁(1 + r₁). So, the probability of default is $F\left (C^{d}\right )$. If the borrower does not carry debt from the first period, he chooses to default if and only if c ≤ (1 + r^n/nd)b₂. Let C^nd ≡ (1 + r^n/nd)b₂. The probability of default is F(C^nd).

The borrower’s expected utility if he carries no debt from the first period, where now we also account for the high income realization probability p_i, is⁷⁰

$$U^{nd}_{2}=u(b_{2})-p_{i}{\int}_{0}^{C^{nd}}cf(c)dc-p_{i}C^{nd}{\int}_{C^{nd}}^{\infty}f(c)dc-(1-p_{i})\overline{c},$$

(C.1)

while if the borrower carries debt from the first period his expected utility is

$${U^{d}_{2}}=u(b_{2})-p_{i}{\int}_{0}^{C^{d}}cf(c)dc-p_{i}C^{d}{\int}_{C^{d}}^{\infty}f(c)dc-(1-p_{i})\overline{c}.$$

(C.2)

Finally, we assume that the upper bound of the support of the cost of default distribution Γ is below a threshold, that is defined in the proof of Proposition 5.⁷¹

The following proposition summarizes the results from the borrower’s utility maximization problem.

Proposition 5

The borrower’s expected utility exhibits a sideways “S” shape, see Fig. 10. The lender will set a nonbinding credit limit to the left of $\overline {B}$ to prevent the borrower from borrowing a lot and then defaulting with certainty. There exists a unique interior level of borrowing that maximizes expected utility, and the demand for credit is downward sloping with respect to the interest rate.

Fig. 10

Second-period expected utility as a function of the amount borrowed. If the credit limit is set below $\overline {B}$, then it is not binding. The borrower in this case will opt for the interior maximum

1.1.2 C.3 Lenders’ problem

We now turn to the lenders’ profit maximization problem. We begin by assuming that the borrower carries debt into the second period. The expected profit function of the lender in period 2 is

$$\begin{array}{@{}rcl@{}} {\pi_{2}^{d}}&=&p_{i}(1-F(C^{d}))\left((1+r^{n/d}){b_{2}^{d}}+(1+r^{o})(1+r_{1})b_{1}\right)-(1+\overline{r})({b_{2}^{d}}+(1+r_{1})b_{1})\\ &=&(p_{i}(1-F(C^{d}))(1+r^{n/d})-(1+\overline{r}))b_{2}+(p_{i}(1-F(C^{d}))(1+r^{o})-(1+\overline{r}))(b_{1}(1+r_{1})). \end{array}$$

The following Lemma, which is the extension of Lemma 1 to the endogenous default case, summarizes the results from the lender’s second-period problem.

Lemma 3

If the borrower carries debt into the second period, the second-period interest rates for old (o) and new (n) debt that maximize the borrower’s expected utility subject to lender j making zero expected profit are:

$$\tilde{r}^{n/d}=\frac{1+\overline{r}-p_{i}(1-F(C^{d}))}{p_{i}(1-F(C^{d}))}+\frac{f(C^{d})C^{d}}{1-F(C^{d})} \text{ and } \tilde{r}^{o}<\frac{1+\overline{r}-p_{i}(1-F(C^{d}))}{p_{i}(1-F(C^{d}))}.$$

(C.3)

The rate on new borrowing is higher than the rate on existing debt.

The rate on new borrowing is the efficient rate and balances optimally the following effects (assuming the rate decreases): A higher b₂ increases the cost according to the cost of funds $\overline {r}$, it increases the profit to the lender in case of repayment (which happens with probability p_i(1 − F(⋅))), and increases the probability of default according to the hazard rate f(⋅)/(1 − F(⋅)). From (C.3), it is clear that the rate on new debt must be higher than the rate on existing debt. The difference with the efficient rate in the case of exogenous default, see Lemma Eq. 1, is that when default is endogenous the efficient rate increases by an amount equal to the hazard rate to optimally induce the borrower to borrow less.

In sum, when we allow for moral hazard and endogenous default, we can obtain, in equilibrium, a reversal in the ranking of the interest rates between old and new debt, relative to when default is exogenous.⁷² In addition, a nonbinding credit limit arises in equilibrium.⁷³ However, the main results and insights we derived with exogenous default will hold here qualitatively, at least for the second period, (i.e., holding r₁ and b₁ fixed). All one has to do is to redefine all the key break-even interest rates from the main analysis, see (4.9), by adding to them the term that accounts for the hazard rate, f(⋅)/(1 − F(⋅)), and define the probability of repayment as p_i(1 − F(⋅)) instead of p_i. The regulation then will force the inside lender to increase the rate on new borrowing, as it would in the case of exogenous default. In other words, the intuition from Fig. 1, and the switching behavior of borrowers in particular, can be readily extended to the case of endogenous default.

1.2 C.4 Switching costs

We lay out an intuition about how switching fixed costs, borrowers incur when in the second period switch from an inside lender to an outsider, should affect the regulated and the unregulated equilibrium. Our purpose with this extension is to argue that it is straightforward to add this kind of friction to our benchmark model.

Suppose borrowers incur a common fixed cost τ > 0 of switching lenders in the second period. Let’s start with the unregulated equilibrium. The offers of the outsiders in the second period are still determined by the Bertrand-type competition among them and so they will not be affected. Moreover, all the rate offers of the inside lender, as given in Proposition 1, will increase by τ, so the inside lender now earns rents from the low types as well. This suggests that second period profits are higher than when τ = 0 and so the first period rate will be lower. Thus, the regulation is more binding for the inside lender than in the absence of switching costs.

Since all rates the inside lender offers in equilibrium increase uniformly by τ, the inside lender still earns higher rents from the high types than the low types and so has more room to maneuver post regulation. If the switching cost is low enough, then only the low types will switch, as in the main analysis. Borrowers incur the cost of switching but the switching cost is already included in the rate offers of the insider, so switching caused by the regulation does not create any additional deadweight loss. But the deadweight loss due to regulation, identified in the main analysis with zero switching costs, remains. In sum, switching costs represent a transfer from the borrowers to the inside lenders in the second period that is competed away in the first period. Regulation has the effects described in Proposition 4.