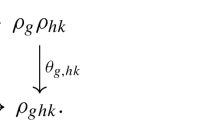Indecomposability of derived categories for arbitrary schemes

Abstract

We extend the criterion of Kawatani and Okawa for indecomposability of the derived category of a smooth projective variety to arbitrary schemes. For relative schemes, we also give a criterion for the nonexistence of semiorthogonal decompositions that are linear over the base. These criteria are based on the base loci of the global or relative dualizing complexes.

Appendix A: Indecomposability of semistable curves (by Shinnosuke Okawa)

Let X be a semistable curve over an algebraically closed field ${\textbf {k}}$; namely, a connected projective nodal curve such that the relative dualizing sheaf $\omega _{ X / {\textbf {k}}}$ of X over ${\textbf {k}}$ is nef, in the sense that $\deg \omega _{ X / {\textbf {k}}} \vert _{ C } \ge 0$ for any irreducible component $C \hookrightarrow X$. It is shown in Corollary 4.4 that ${{\text{Perf }}}(X)$ admits no semiorthogonal decomposition provided that $\omega _{ X /{\textbf {k}}}$ is ample and there is no LCRT. The aim of this appendix is to answer the question by Alexander Kuznetsov whether Corollary 4.4 generalizes to all semistable curves. The author is indebted to him for asking the question and for his useful comments. The author was partially supported by JSPS Grants-in-Aid for Scientific Research (18H01120, 19KK0348, 20H01797, 20H01794, 21H04994, 23H01074).

Proposition 5.1

Let X be a nodal connected projective curve over an algebraically closed field ${\textbf {k}}$. Then ${{\text{Perf }}}(X)$ is semiorthogonally indecomposable if and only if X is semistable.

For the sake of completeness, we first show the following easy lemma. Below ${{\,\mathrm{{Pic}}\,}}^{ 0 } ( X )$ denotes the identity component of the Picard scheme.

Lemma 5.2

Let X be a reduced and connected projective curve over ${\textbf {k}}$ and $L \in {{\,\mathrm{{Pic}}\,}}^{ 0 } ( X )$ such that $L \not \simeq \mathcal{O}_{ X }$. Then $H ^{ 0 } \left( X, L \right) = 0$.

Proof

Suppose $L \in {{\,\mathrm{{Pic}}\,}}^{ 0 } \left( X \right)$ admits a global section $0 \ne s \in H ^{ 0 } \left( X, L \right)$.

If $s \vert _{ C } \ne 0$ for any irreducible component $C \hookrightarrow X$, then obviously s must be nowhere vanishing. This would imply $L \simeq \mathcal{O}_{ X }$.

Suppose that $s \vert _{ C } = 0$ for some irreducible component C. We can assume without loss of generality that there is another component $D \hookrightarrow X$ which intersects C and $s \vert _{ D } \ne 0$. Since $L \vert _{ D } \in {{\,\mathrm{{Pic}}\,}}^{ 0 } \left( D \right)$, it follows that $s \vert _{ D }$ is a nowhere vanishing global section of $L \vert _{ D }$. This contradicts $C \cap D \ne \emptyset$ and $s \vert _{ C } = 0$. $\square$

Lemma 5.3

Let X be a nodal projective curve over ${\textbf {k}}$ such that $\dim _{ {\textbf {k}}} H ^{ 1 } \left( X, \mathcal{O}_{ X } \right) > 0$. Then there exists an invertible sheaf $L \not \simeq \mathcal{O}_{ X }$ such that $L \in {{\,\mathrm{{Pic}}\,}}^{ 0 } ( X )$.

Proof

Since $\dim X = 1$, it follows that $H ^{ 2 } \left( X, \mathcal{O}_{ X } \right) = 0$. The deformation theory of coherent sheaves implies that ${{\,\mathrm{{Pic}}\,}}^{ 0 } \left( X \right)$ is a smooth connected group scheme of dimension $\dim _{ {\textbf {k}}} H ^{ 1 } \left( X, \mathcal{O}_{ X } \right) > 0$. Hence we can take L to be the line bundle corresponding to any point of ${{\,\mathrm{{Pic}}\,}}^{ 0 } \left( X \right) {\setminus } \left\{ \mathcal{O}_{ X } \right\}$. $\square$

Proof of Proposition 5.1

Let us first assume X is semistable, and show that ${{\text{Perf }}}(X)$ is indecomposable. As in Theorem 1.4 in [15], let us consider the paracanonical base locus:

$$\begin{aligned} \textsf {PBs}( X ) := \bigcap _{ L \in {{\,\mathrm{{Pic}}\,}}^{ 0 } ( X ) } \textsf {Bs}( L \otimes \omega _{ X / {\textbf {k}}} ) \left( \hookrightarrow \textsf {Bs}\omega _{ X / {\textbf {k}}} \hookrightarrow X \right) \end{aligned}$$

(5.1)

As in the smooth case, if one can show that $| \textsf {PBs}( X ) | < \infty$, then the semiorthogonal indecomposability of ${{\text{Perf }}}(X)$ follows. This follows from that any semiorthogonal decomposition of ${{\text{Perf }}}(X)$ is stable under the action of ${{\,\mathrm{{Pic}}\,}}^{ 0 } \left( X \right)$ by Theorem 3.8 in [8]. Indeed, take a closed point $x \in X _{ \text{sm} } {\setminus } \textsf {PBs}( X )$. Let ${{\text{Perf }}}(X) = \langle \mathcal{A}, \mathcal{B}\rangle$ be a semiorthogonal decomposition and

$$\begin{aligned} b \rightarrow \mathcal{O}_{ x } \rightarrow a \rightarrow b [ 1 ] \end{aligned}$$

(5.2)

be the corresponding distinguished triangle. Then for any $L \in {{\,\mathrm{{Pic}}\,}}^{ 0 } ( X )$ it follows that $b \otimes L \in \mathcal{B}$ and $a \otimes L \in \mathcal{A}$ by the stability. Hence from (5.2) $\otimes L$ we get $b \otimes L \simeq b$ and $a \otimes L \simeq a$. By assumption there are $L \in {{\,\mathrm{{Pic}}\,}}^{ 0 } ( X )$ and $s \in H ^{ 0 } \left( X, L \otimes \omega _{ X / {\textbf {k}}} \right)$ such that $s ( x ) \ne 0$. Thus we get a map

$$\begin{aligned} b \simeq b \otimes L ^{ - 1 } \xrightarrow []{ s \cdot } b \otimes \omega _{ X / {\textbf {k}}} \end{aligned}$$

(5.3)

which is an isomorphism on an open neighborhood of x. Then by standard arguments (see, say, the proof of Theorem 3.3) we can conclude that $\mathcal{O}_{ x }$ is contained in either $\mathcal{A}$ or $\mathcal{B}$. Then, finally, one can show that all points of $X _{ \text{sm} } {\setminus } \textsf {PBs}( X )$ are simultaneously contained in either $\mathcal{A}$ or $\mathcal{B}$ by the arguments of Corollary 3.9.

In the rest we show that $\textsf {PBs}( X )$ is a finite set if X is a semistable curve. By Corollary 1.2 in [22],¹ it is enough to show that a general closed point of any LCRT component (or a separating line, after [22]) is not contained in $\textsf {PBs}( X )$.

Let ${\mathbb P} ^{ 1 } \simeq E \hookrightarrow X$ be an LCRT component. By definition, there are mutually disjoint subcurves $X _{ 1 }, \ldots , X _{ r } \hookrightarrow X$

for some $r \ge 2$ such that $X = \left( \bigcup _{ i = 1 } ^{ r } X _{ i } \right) \cup E$ and $| X _{ i } \cap E | = 1$ for $i = 1, \ldots , r$ (hence the name comb). Then it follows for each i that $\dim _{ {\textbf {k}}} H ^{ 1 } \left( X _{ i }, \mathcal{O}_{ X _{ i } } \right) > 0$. Indeed, otherwise $X _{ i}$ is a tree of ${\mathbb P} ^{ 1 }$s and hence the component of $X _{ i }$ which corresponds to a leaf of the dual graph of $X _{ i }$ would destabilize X. Hence we can apply Lemma 5.3 to each $X _{ i }$ to obtain a non-trivial invertible sheaf $L _{ i } \in {{\,\mathrm{{Pic}}\,}}^{ 0 } \left( X _{ i } \right)$. Now let $L \in {{\,\mathrm{{Pic}}\,}}^{ 0 } ( X )$ be such that $L \vert _{ X _{ i } } \simeq L _{ i }$ for all $i = 1, \ldots , r$ (see, say, the 1st paragraph of Example 0.7.2 in [5] for the existence of such L).

For a general closed point $x \in E$ consider the following short exact sequence

$$\begin{aligned} 0 \rightarrow L \otimes \omega _{ X / {\textbf {k}}} ( - x ) \rightarrow L \otimes \omega _{ X / {\textbf {k}}} \rightarrow \mathcal{O}_{ x } \rightarrow 0, \end{aligned}$$

(5.4)

from which we obtain the following exact sequence.

$$\begin{aligned} H ^{ 0 } \left( X, L \otimes \omega _{ X / {\textbf {k}}} \right) \rightarrow H ^{ 0 } \left( \mathcal{O}_{ x } \right) \rightarrow H ^{ 1 } \left( X, L \otimes \omega _{ X / {\textbf {k}}} ( - x ) \right) \end{aligned}$$

(5.5)

The last term is isomorphic to the dual of the following vector space by the Serre duality.

$$\begin{aligned} H ^{ 0 } \left( X, L ^{ - 1 } ( x ) \right) \end{aligned}$$

(5.6)

By Lemma 5.2, for each $i = 1, \ldots , r$ we have $H ^{ 0 } \left( X _{ i }, L ^{ - 1 } ( x ) \vert _{ X _{ i } } \right) = 0$. Finally, as $L ^{ - 1 } ( x ) \vert _{ E } = \mathcal{O}_{ E } ( 1 )$ and $r \ge 2$, we conclude that (5.6) vanishes.

Conversely, let X be a curve as in the assertion which is not semistable. Then there must be an irreducible component $C \hookrightarrow X$ such that $C \simeq {\mathbb P} ^{ 1 }$ and C meets the other irreducible components of X in exactly 1 point. Then Proposition 6.15 in [14] implies that there is a nontrivial semiorthogonal decomposition of ${D}^b_c( X)$, which implies that ${{\text{Perf }}}(X)$ admits a nontrivial semiorthogonal decomposition by Corollary 6.6 in [13]. $\square$

Remark 5.4

When the components $X _{ 1 },\ldots , X _{ r }$ in the proof of Proposition 5.1 are all smooth, then we can prove Proposition 5.1 by means of the relative canonical base locus. This can be regarded as a sophisticated version of Lin’s argument.

Indeed, in this case we have a morphism as follows, which contracts the LCRT E in the proof of Proposition 5.1 to a point.

$$\begin{aligned} f :X \rightarrow \prod _{ i = 1 } ^{ r } {{\,\mathrm{{Pic}}\,}}^{ 0 } \left( X _{ i } \right) \end{aligned}$$

(5.7)

One can easily show that $\text{Bs} |f| = \emptyset$ (recall that $r \ge 2$). Hence there is no f-linear semiorthogonal decomposition of ${{\text{Perf }}}(X)$ by Theorem 3.6. Now since $\prod _{ i = 1 } ^{ r } {{\,\mathrm{{Pic}}\,}}^{ 0 } \left( X _{ i } \right)$ is an abelian variety, any semiorthogonal decomposition of ${{\text{Perf }}}(X)$ is f-linear by Theorem 1.4 in [21]. Hence the conclusion. It is interesting to ask if one can push the same strategy without assuming $X _{ i }$ are smooth.