The Conley–Zehnder indices of the spatial Hill three-body problem

Abstract

We explore the interaction between the Conley–Zehnder index and bifurcation points of symmetric planar as well as spatial periodic orbits in the spatial Hill three-body problem. We start with the fundamental families of planar periodic orbits which are those of direct (family g) and retrograde periodic orbits (family f). Since the spatial system is invariant under a symplectic involution, whose fixed point set corresponds to the planar problem, planar orbits have planar and spatial Floquet multipliers, and planar and spatial Conley–Zehnder indices. When the Floquet multipliers move through a root of unity, new families of periodic orbits bifurcate and the index jumps. For very low energies, the families g and f arise dynamically from the rotating Kepler problem, and in a recent work (Aydin From Babylonian lunar observations to Floquet multipliers and Conley-Zehnder Indices) we determined analytically their indices. By their numerical continuations for higher energies, we determine the index of various families of planar and spatial periodic orbits bifurcating from g and f. Since these families can bifurcate again and meet each other, this procedure can get complicated. This index leads to a grading on local Floer homology. Since the local Floer homology and its Euler characteristic stay invariant under bifurcation, the index provides important information about the interconnectedness of such families, which we illustrate in form of bifurcation graphs. Since the solutions of Hill’s system may serve as orbits for space mission design or astronomical observations, our results promote the interaction between Symplectic Geometry and practical problems.

1 Introduction, tools and results

The Hill three-body problem is a limiting case of the restricted three-body problem which was firstly proposed by Hill (1878), whose motivation was to describe the motion of the Moon. In this limiting case the massless body is attracted by two primaries, one of which is infinitely much heavier than the second one. In Hill’s original set-up, the primaries are the Sun and the Earth. In rotating coordinates, one shifts the smaller primary to the origin and zooms in a region around it by pushing the huge primary off to infinity (see Fig. 1). The goal is to understand the dynamics of the massless body.

Fig. 1

The Hill three-body problem, e.g., for the Sun–Earth–Moon system

The Hamiltonian describing the motion of the massless body in the spatial problem is

$$\begin{aligned} H :T^* \big ( \mathbb {R}^3 \setminus \{ (0,0,0) \} \big ) \rightarrow \mathbb {R},\quad (q,p) \mapsto \underbrace{\frac{1}{2}|p|^2 {-} \frac{1}{|q|} {+} p_1q_2 {-} p_2q_1}_{\text {rotating Kepler problem}} {-} q_1^2 {+} \frac{1}{2}q_2^2 {+} \frac{1}{2}q_3^2. \end{aligned}$$

(1)

Note that the phase space is the trivial cotangent bundle $T^*\left( \mathbb {R}^3 {\setminus } \{(0,0,0)\} \right)$ endowed with the canonical symplectic form $\omega = \sum dq_i \wedge dp_i$, where $q=(q_1,q_2,q_3)$ denotes the position of the massless body and $p=(p_1,p_2,p_3)$ its momentum in the fiber. For the derivation of the Hamiltonian we refer to (Belbruno et al. 2019, pp. 3–4). The Hamiltonian (1) consists of the rotating Kepler problem (e.g., Earth–Moon) with a velocity independent gravitational perturbation produced by the massive body (e.g., Sun). The restriction of the spatial system to $\{q_3 = p_3 = 0\}$ gives the planar problem. Moreover, completing the squares yields

$$\begin{aligned} H(q,p) = \frac{1}{2}\big ( (p_1 + q_2)^2 + (p_2 - q_1)^2 + p_3^2 \big ) +V(q), \end{aligned}$$

where the effective potential is defined as $V(q) = - \frac{1}{|q|} - \frac{3}{2}q_1^2 + \frac{1}{2}q_3^2$. Since we work with symplectic coordinates, we treat the spatial Hill equation in (q, p)-coordinates given by

$$\begin{aligned} {\left\{ \begin{array}{ll} \dot{q}_1 = p_1 + q_2,\quad \quad \quad &{} {\dot{p}_1 = p_2 - q_1 - \frac{\partial V}{\partial q_1}},\\ \dot{q}_2 = p_2 - q_1, &{} {\dot{p}_2 = - p_1 - q_2 - \frac{\partial V}{\partial q_2}},\\ \dot{q}_3 = p_3, &{} {\dot{p}_3 = - \frac{\partial V}{\partial q_3}}. \end{array}\right. } \end{aligned}$$

(2)

Remark 1.1

The equation (2) is equivalent, via the transformations $\ddot{q}_1 = \dot{p}_1 + \dot{q}_2$, $\ddot{q}_2 = \dot{p}_2 - \dot{q}_1$ and $\ddot{q}_3 = \dot{p}_3$, to the equation in $(q,\dot{q})$-coordinates given by

$$\begin{aligned} \begin{pmatrix} \ddot{q}_1\\ \ddot{q}_2\\ \ddot{q}_3 \end{pmatrix} + 2 \begin{pmatrix} 0 &{} -1 &{} 0\\ 1 &{} 0 &{} 0\\ 0 &{} 0 &{} 0 \end{pmatrix} \cdot \begin{pmatrix} \dot{q}_1\\ \dot{q}_2\\ \dot{q}_3 \end{pmatrix} + \nabla V (q) = 0 \quad \Leftrightarrow \quad {\left\{ \begin{array}{ll} {\ddot{q}_1 = 2 \dot{q}_2 + 3q_1 - \frac{q_1}{|q|^3}} \\ {\ddot{q}_2 = -2\dot{q}_1 - \frac{q_2}{|q|^3}} \\ {\ddot{q}_3 = -q_3\bigg (\frac{1}{|q|^3} + 1 \bigg )} \end{array}\right. } \end{aligned}$$

Fig. 2

Direct and retrograde periodic orbits for very low energies

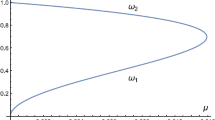
The fundamental families of planar periodic orbits around the smaller primary located at the origin are those of direct and retrograde periodic orbits, which since the work of Elis Strömgren and his associates in the Copenhagen Observatory are traditionally called family g resp. f (see the Copenhagen category in Szebehely 1967, Chapter 9.4). For low energy values, these two families begin with infinitesimal circular direct resp. retrograde periodic orbits, see Fig. 2. Based on them, we determine the Conley–Zehnder index of various families.

1.1 Why is the Conley–Zehnder index a powerful tool?

• (1) The Conley–Zehnder index is a kind of a mean winding number for the linearized flow, whose non-trivial eigenvalues are called “Floquet multipliers”. These have deep astronomical significance. Notice that in Hill’s system the first return time of a planar periodic orbit around the smaller primary corresponds to the synodic month. In particular, Hill found numerically in Hill (1878) a planar direct periodic orbit with the period of the synodic month. In our recent work (Aydin 2022) we relate Hill’s orbit to the astronomical lunar periods of our moon, which date back to the Babylonians until around 500 BCE. In fact, the anomalistic and draconitic months can be expressed in terms of its Floquet multipliers and indices.

• (2) The index jumps by integers when bifurcation points are crossed. In particular, when the Floquet multipliers move through a root of unity, new families of periodic orbits bifurcate. Since these families can bifurcate again and meet each other, this procedure can get complicated. This index leads to a grading on local Floer homology which stays invariant under bifurcation. Therefore the index provides important information about the interconnectedness of such families.

• (3) If one wants to study the poles of a planet or of a moon one needs to leave the ecliptic and find spatial orbits, for instance through bifurcation from planar orbits. A “bridge" is an orbit cylinder between two orbits, i.e., it is a connection by a 1-parameter family of orbits with varying energy and constant index. Since the planar direct and retrograde orbits move in the ecliptic around the smallest primary in opposite direction, a bridge of spatial orbits between them is of special interest.

1.2 Tools

In order to derive the relation from (1) a general framework and construction for investigations of planar as well as spatial periodic orbits, their bifurcations and Conley–Zehnder indices were developed. In the following we summarize these methods in five steps and refer to Aydin (2022) for details.

1.2.1 Symplectic splitting for planar periodic orbits

The Hamiltonian 1 is invariant under the symplectic involution

$$\begin{aligned} \sigma :T^* \mathbb {R}^3 \rightarrow T^* \mathbb {R}^3,\quad (q,p) \mapsto (q_1,q_2,-q_3,p_1,p_2,-p_3), \end{aligned}$$

which arises by reflection at the ecliptic $\{q_3=0\}$. The planar problem can be viewed as the restriction of this system to the fixed point set of $\sigma$. Let $q=(q,p)$ be a planar periodic orbit with $q_0 = \big (q(0),p(0)\big )$ and first return time $T_q$. Consider the time $T_q$ map of the linearized Hamiltonian flow, which is a $6 \times 6$ symplectic matrix and called “monodromy”. Since q is invariant under $\sigma$, its differential induces a symplectic involution commuting with the flow, i.e.,

$$\begin{aligned} d \sigma (q_0) \circ d \varphi _H^{T_q} (q_0) \circ d \sigma (q_0) = d \varphi _H^{T_q} (q_0). \end{aligned}$$

Therefore the monodromy leaves the eigenspaces $T_{q_0}\text {Fix}(\sigma )$ and $E_{-1} \big ( d \sigma ( q_0 ) \big )$ invariant. The first space is formed by 4-dimensional planar coordinates and the latter by 2-dimensional spatial coordinates. In particular, since these two eigenspaces are symplectically orthogonal, each of them is a symplectic vector spaces and the monodromy admits a symplectic decomposition

$$\begin{aligned}{} & {} d \varphi _H^{T_q} (q_0) :T_{q_0}\text {Fix}(\sigma ) \oplus E_{-1} \big ( d \sigma ( q_0 ) \big ) \rightarrow T_{q_0}\text {Fix}(\sigma ) \oplus E_{-1} \big ( d \sigma ( q_0 ) \big ), \\{} & {} d \varphi _H^{T_q} (q_0) = \begin{pmatrix} A_p &{} 0 \\ 0 &{} A_s \end{pmatrix},\quad A_p \in \text {Sp}(2),\quad A_s \in \text {Sp}(1) = \text {SL}(2,\mathbb {R}). \end{aligned}$$

Note that $A_p$ is the monodromy of q viewed in the planar problem and $A_s$ is a $2 \times 2$ symplectic matrix which arises by linearization only along the spatial components.

Remark 1.2

The symplectic involution $-\sigma$ leaves the Hamiltonian (1) invariant as well, whose underlying geometry is the rotation around the $q_3$-axis by $\pi$. Its fixed point set $\text {Fix}(-\sigma )$ corresponds to the 2-dimensional symplectic complement of $T_{q_0}\text {Fix}(\sigma )$.

The restriction to the 5-dimensional energy hypersurface $\Sigma$ induces on the quotient by the line bundle ker$\omega |_{\Sigma } = \langle X_H | _{\Sigma } \rangle \subset T\Sigma$ the “reduced monodromy”, whose eigenvalues are called “Floquet multipliers”. In view of the symplectic splitting of the induced 4-dimensional symplectic vector space into

$$\begin{aligned} T_{q_0} \Sigma / \text {ker} \omega _{q_0} = \big ( T_{q_0} \text {Fix}(\sigma |_{\Sigma }) / \text {ker} \omega _{q_0} \big ) \oplus \Big ( E_{-1} \big ( d \sigma ( q_0 ) \big ) \Big ), \end{aligned}$$

the reduced monodromy is of the form

$$\begin{aligned} \overline{d \varphi _H^{T_q} | _{\Sigma } (q_0) } = \begin{pmatrix} \overline{A}_p &{} 0 \\ 0 &{} A_s \end{pmatrix},\quad \overline{A}_p, A_s \in \text {Sp}(1) = \text {SL}(2,\mathbb {R}), \end{aligned}$$

(3)

where $\overline{A}_p$ is the reduced monodromy of q viewed in the planar problem. In particular, we obtain the following two important properties.

• (i) The Floquet multipliers are determined by those of $\overline{A}_p$ and $A_s$, which are real or lie on the unit circle. Consequently, it is not possible that the Floquet multipliers are given by four different complex numbers of the form $\lambda , 1/\lambda , \overline{\lambda }$ and $1/\overline{\lambda }$.

• (ii) The transversal Conley–Zehnder index of q splits additively $\mu _{CZ} = \mu _{CZ}^p + \mu _{CZ}^s$, where $\mu _{CZ}^p$ and $\mu _{CZ}^s$ are the Conley–Zehnder indices of the path of symplectic matrices generated by the planar and spatial part of the linearized Hamiltonian flow, respectively.

Remark 1.3

To compute $\overline{A}_p$ and $A_s$ we linearize the equation (2) along a periodic orbit, i.e., we expand $( q + \Delta q, p + \Delta p )$ near (q, p) and obtain the linearized equation

$$\begin{aligned} {\left\{ \begin{array}{ll} \Delta \dot{q}_1 = \Delta p_1 + \Delta q_2,\quad \quad \quad &{} \Delta \dot{p}_1 = \Delta p_2 - \Delta q_1 - \left( \text {H}_V (q) \cdot \Delta q \right) _1,\\ \Delta \dot{q}_2 = \Delta p_2 - \Delta q_1, &{} \Delta \dot{p}_2 = - \Delta p_1 - \Delta q_2 - \left( \text {H}_V (q) \cdot \Delta q \right) _2,\\ \Delta \dot{q}_3 = \Delta p_3, &{} \Delta \dot{p}_3 = - \left( \text {H}_V (q) \cdot \Delta q \right) _3, \end{array}\right. } \end{aligned}$$

which reads for planar periodic orbits

$$\begin{aligned} {\left\{ \begin{array}{ll} \Delta \dot{q}_1 = \Delta p_1 + \Delta q_2,\quad \quad \quad &{} \Delta \dot{p}_1 = \Delta p_2 - \Delta q_1 + {\bigg (\frac{2q_1^2 - q_2^2}{|q|^5} + 3\bigg ) \Delta q_1 + \frac{3q_1q_2}{|q|^5} \Delta q_2},\\ \Delta \dot{q}_2 = \Delta p_2 - \Delta q_1, &{} \Delta \dot{p}_2 = - \Delta p_1 - \Delta q_2 + {\frac{3q_1q_2}{|q|^5} \Delta q_1 + \frac{2q_2^2 - q_1^2}{|q|^5} \Delta q_2}, \\ \Delta \dot{q}_3 = \Delta p_3, &{} \Delta \dot{p}_3 = { - \bigg (\frac{1}{|q|^3} + 1\bigg ) \Delta q_3 }. \end{array}\right. } \end{aligned}$$

1.2.2 Stability, index and iteration

Each of the planar and spatial stability behavior is partitioned into three classes, depending on the traces of $\overline{A}_p$ and $A_s$. In view of the symplectic decomposition of the reduced monodromy given by (3), for each index we consider the transversal Conley–Zehnder index with standard normalization or counter-clockwise normalization for non-degenerate paths, as defined by Hofer et al. (2003), Appendix, Hofer et al. (1998), Sect. 3. Since the discussion of the planar and spatial properties is similar, in the following we restrict to the planar case.

• (a) Elliptic $|\text {tr}(\overline{A}_p)|<2$ and the eigenvalues are on the unit circle and of the form $e^{\pm \text {i}\theta _p}$. Then $\overline{A}_p$ is conjugate to a rotation in $\mathbb {R}^2$. The index $\mu _{CZ}^p$ measures the number of complete rotations during $T_q$, which we denote by $\text {rot}^p(q)$. They are related by

  $$\begin{aligned} \mu _{CZ}^p = 1 + 2 \cdot \text {rot}^p(q). \end{aligned}$$

  Therefore for every complete rotation the index jumps by 2 and is odd. By $\varphi _p$ we denote the angle of rotation and define the anomalistic period $T_a$ as the period for a complete rotation, which in terms means

  $$\begin{aligned} T_a = \frac{T_q}{\text {rot}^p(q) + \varphi _p/(2\pi )}. \end{aligned}$$

  The same spatial formalism gives rise to the definition of the draconitic period $T_d$.

• (b) Positive hyperbolic $\text {tr}(\overline{A}_p)>2$ and the eigenvalues are positive real and of the form $\lambda _p$, $1/\lambda _p$. If the orbit changes its stability from elliptic to positive hyperbolic, or vice versa, then the eigenvalue 1 is crossed and the index jumps by $\pm 1$. If the rotation angle moves through 0 to positive hyperbolic, then $\mu _{CZ}^p$ jumps by $-1$ and if it goes through $2 \pi$ to positive hyperbolic, then $\mu _{CZ}^p$ jumps by $+1$. For the other way, the change of index is exactly backwards (see Fig. 3). Note that in the positive hyperbolic case the index is even.

• (c) Negative hyperbolic $\text {tr}(\overline{A}_p)<2$ and the eigenvalues are negative real and of the form $\lambda _p$, $1/\lambda _p$. For the cases from elliptic to negative hyperbolic, or vice versa, the index does not change, i.e., in the negative hyperbolic case the index is odd as well.

Fig. 3

The index jump by $\pm 1$ in the elliptic and positive hyperbolic transitions

Let $q^n$ be the n times iteration of q with first return time $nT_q$, $n \ge 1$. We call $q^n$ the n-th cover of q. Assume that $q^n$ is non-degenerate for all $n \ge 1$, then the index iteration (we refer to Hofer et al. (2003), p. 249 for details) is given by Table 1.

Table 1 Index iteration

Recall that for the cases from elliptic to negative hyperbolic, or vice versa, the index does not change. Nevertheless, its double cover crosses the eigenvalue 1 and the index of its double cover jumps by $\pm 1$. Notice that the double cover of a negative hyperbolic periodic orbits is positive hyperbolic. In the elliptic case, if the rotation angle is a $\tilde{k}$-th root of unity, for $\tilde{k} \ge 3$ the $\tilde{k}$-th cover is still elliptic and goes through the eigenvalue 1, thus its index jumps by $\pm 2$.

1.2.3 Bifurcation, local Floer homology, good and bad orbits

We now work locally near a family of non-degenerate periodic orbits. The crossing of the eigenvalue 1, and thereby the index jump, generates bifurcations of new families of planar or spatial periodic orbits, respectively. For details on the existence and properties of such bifurcations we refer to the book of Abraham and Marsden (1978, pp. 597–604). The Conley–Zehnder index leads to a grading on the local Floer homology. At a bifurcation point, the local Floer homology and its Euler characteristic stay invariant. Since its discussion goes beyond the scope of this article, we refer the curious reader for details on local Floer homology to the article by Ginzburg (2010). Moreover we consider unparametrized periodic orbits, hence we need to use the equivariant Floer homology, whose local version is described by Ginzburg and Gürel (2020). In our set-up, we need to use the local version of the $S^1$-equivariant Floer homology of the Rabinowitz action functional. This functional is given by

$$\begin{aligned} \mathscr {A}^H :\mathcal {L} \times \mathbb {R}_{>0} \rightarrow \mathbb {R},\quad (\gamma ,\tau ) \mapsto \int _{\textrm{S}^1} \lambda \big ( \dot{\gamma }(t) \big ) - \tau \int _{\textrm{S}^1} H\big (\gamma (t)\big ) \mathrm{{d}}t, \end{aligned}$$

where $\mathcal {L} = C^{\infty }(S^1,T^* \mathbb {R}^3)$ is the free loop space of $T^* \mathbb {R}^3$. It can be thought as the Lagrange multiplier functional of the area functional for the constraint given by the mean value of the Hamiltonian. Rabinowitz used this functional to prove existence of periodic orbits on starshaped hypersurfaces in $\mathbb {R}^{2n}$. The critical points of $\mathscr {A}^H$ consist of pairs $(\gamma ,\tau )$ which are solutions of

$$\begin{aligned} {\left\{ \begin{array}{ll} \dot{\gamma }(t) = \tau X_H\big ( \gamma (t) \big )\\ 0 = H\big (\gamma (t)\big ), \end{array}\right. } \end{aligned}$$

i.e., the critical points of $\mathscr {A}^H$ are parametrized periodic orbits of $X_H$ of period $\tau$ on the fixed energy level set $H^{-1}(0)$. Note that since we consider unparametrized periodic orbits, we mod out the circle $S^1$ action given by reparametrization of the free loop space. The Floer homology for the Rabinowitz action functional was introduced by Cieliebak and Frauenfelder (2009) and the $S^1$-equivariant Rabinowitz–Floer homology was discussed by Frauenfelder and Schlenk (2016), whose local version is our tool. Notice that for every $\tilde{k}$-th cover of a periodic orbit there is a local $S^1$-equivariant Rabinowitz–Floer homology associated to this $\tilde{k}$-th cover. Given a family $\tilde{\gamma }$ of non-degenerate unparametrized periodic orbits, we denote by $RFH^{S^1}_*(\tilde{\gamma })$ its local $S^1$-equivariant Rabinowitz–Floer homology and by $\chi (\tilde{\gamma })$ its Euler characteristic

$$\begin{aligned} \chi (\tilde{\gamma }) = \sum _{m \in \mathbb {Z}} (-1)^m \dim RFH^{S^1}_m (\tilde{\gamma }). \end{aligned}$$

The following two important examples are analogous to those given in Ginzburg and Gürel (2020), p. 540.

Example 1.4

Let $\tilde{\gamma }$ be a family of simple closed non-degenerate periodic orbits. Then, $RFH^{S^1}_*(\tilde{\gamma })$ has rank one when $*$ equals the Conley–Zehnder index of $\tilde{\gamma }$ and zero otherwise.

Example 1.5

Assume that an iterated planar periodic orbit $q^{\tilde{k}}$ is non-degenerate for all $\tilde{k} \ge 1$. Its indices we denote by $\mu _{CZ}^p(q^{\tilde{k}})$ and $\mu _{CZ}^s(q^{\tilde{k}})$ which are determined by the index iteration in Table 1. Let $\mu _{CZ}^p(q)$ and $\mu _{CZ}^s(q)$ be the indices of the underlying simple closed periodic orbit q, then if

$$\begin{aligned} \mu _{CZ}^p(q^{\tilde{k}}) \equiv \mu _{CZ}^p(q) \text { mod } 2, \quad \mu _{CZ}^s(q^{\tilde{k}}) \equiv \mu _{CZ}^s(q) \text { mod } 2, \end{aligned}$$

(4)

or both equations in (4) are not simultaneously satisfied, then $q^{\tilde{k}}$ is called a good orbit. Otherwise, $q^{\tilde{k}}$ is called a bad orbit. Therefore, all simple closed periodic orbits are good. Furthermore, bad orbits occur as $\tilde{k}$-th cover of planar periodic orbits which are either planar or spatial negative hyperbolic, where $\tilde{k}$ is even. If $\tilde{\gamma }$ is a family of good orbits, then

$$\begin{aligned} RFH^{S^1}_* (\tilde{\gamma }; \, \mathbb {Q}) = {\left\{ \begin{array}{ll} \mathbb {Q}, &{} * = \mu _{CZ}\\ 0, &{} \text {otherwise}. \end{array}\right. } \end{aligned}$$

If $\tilde{\gamma }$ is a family of bad orbits, then $RFH^{S^1}_* (\tilde{\gamma } \,; \, \mathbb {Q}) = 0$ in all degrees. Hence bad orbits contribute nothing to the local homology and the Euler characteristic. For planar families we have two local homologies, namely one viewed in the planar problem and one in the spatial problem. They differ only by the index shift given by $\mu _{CZ}^s$ and we denote them by

$$\begin{aligned} pRFH^{S^1}_* (\tilde{\gamma }; \, \mathbb {Q}),\quad \chi _p(\tilde{\gamma }),\qquad \quad sRFH^{S^1}_* (\tilde{\gamma }; \, \mathbb {Q}),\quad \chi _s(\tilde{\gamma }). \end{aligned}$$

Note that a planar periodic orbit can be a good orbit viewed in the planar system but a bad orbit considered in the spatial system, and vice versa.

1.2.4 Indices of family g and f for very low energies

In order to determine the Conley–Zehnder indices, one studies the birth of families of orbits, i.e., where and how do the families of orbits dynamically arise? One source of known periodic orbits in the Hill three-body problem is the rotating Kepler problem. For very low energies, one approaches the rotating Kepler problem which after regularization becomes the geodesic flow. In fact, for very low energies the families g and f bifurcate from the geodesic flow, resp. from the circular direct and retrograde periodic orbits in the rotating Kepler problem (illustrated in Fig. 2). For very small energy values their indices and rotation numbers are

$$\begin{aligned} \mu _{CZ}^p = \mu _{CZ}^s = {\left\{ \begin{array}{ll} 3 &{} \text { for family }g\\ 1 &{} \text { for family }f \end{array}\right. } \quad \Leftrightarrow \quad \text {rot}^p(q) = \text {rot}^s(q) = {\left\{ \begin{array}{ll} 1 &{} \text { for family }g\\ 0 &{} \text { for family }f. \end{array}\right. } \end{aligned}$$

Geometrically, while for family g the anomalistic and draconitic period are shorter than the synodic period, for family f they are longer. Moreover,

$$\begin{aligned}{} & {} pRFH^{S^1}_* (g; \, \mathbb {Q}) = {\left\{ \begin{array}{ll} \mathbb {Q}, &{} *=3\\ 0, &{}\text {otherwise}, \end{array}\right. }\quad sRFH^{S^1}_* (g; \, \mathbb {Q}) = {\left\{ \begin{array}{ll} \mathbb {Q}, &{} *=6\\ 0, &{}\text {otherwise}, \end{array}\right. } \\{} & {} pRFH^{S^1}_* (f; \, \mathbb {Q}) = {\left\{ \begin{array}{ll} \mathbb {Q}, &{} *=1\\ 0, &{}\text {otherwise}, \end{array}\right. }\quad sRFH^{S^1}_* (f; \, \mathbb {Q}) = {\left\{ \begin{array}{ll} \mathbb {Q}, &{} *=2\\ 0, &{}\text {otherwise}, \end{array}\right. } \\{} & {} \chi _p(g) {=} (-1)^3 {=} {-}1,\quad \chi _s(g) {=} (-1)^6 {=} 1,\quad \chi _p(f) {=} (-1)^1 {=} -1,\quad \chi _s(f) {=} (-1)^2 {=} 1. \end{aligned}$$

Remark 1.6

In addition, for very low energies two families of spatial collision periodic orbits bifurcate from the Kepler problem as well. Recall that planar orbits are in $\text {Fix}(\sigma )$, whose 2-dimensional symplectic complement corresponds to $\text {Fix}(-\sigma ) = \{ (0,0,q_3,0,0,p_3) \}$. These two families of spatial collision orbits are in $\text {Fix}(-\sigma )$. While one family collides with the smaller primary at the origin from above, the other family collides from below. Each of these two families has index 4.

1.2.5 Symmetry specifies the Floquet multipliers and the index jump

In view of Fig. 3 a natural question to ponder is how can we decide the index jump. In other words, how can we specify the Floquet multipliers, e.g., in the elliptic case how can we find out whether the rotation is by $\theta _p$ or $- \theta _p$? While their calculation does not provide enough information, the orbit’s invariance under an anti-symplectic involution plays the key role.

Linear symmetries Hill’s system is equipped with important linear symmetries which are traditionally well-known. A symmetry $\rho$ is, by definition, a symplectic or anti-symplectic involution of the phase space which leaves the Hamiltonian invariant, i.e. $H \circ \rho = H,\ \rho ^2 = \text {id}$ and $\rho ^* \omega = \pm \omega$. Besides the symplectic symmetries $\pm \sigma$ other symplectic symmetries are $\pm \text {id}$. Four anti-symplectic symmetries of the Hamiltonian (1) are given in Table 2.

Table 2 Linear anti-symplectic symmetries

Notice that these eight linear symmetries form the group $\mathbb {Z}_2 \times \mathbb {Z}_2 \times \mathbb {Z}_2$. Since the maps $\sigma$, $\rho _1$, $\rho _2$, $\overline{\rho _1}$ and $\overline{\rho _2}$ leave $\text {Fix}(\sigma )$ invariant, the two maps

$$\begin{aligned} \rho _1 |_{\text {Fix}(\sigma )} (q,p) = \overline{\rho _1} |_{\text {Fix}(\sigma )} (q,p) \text { and }\rho _2 |_{\text {Fix}(\sigma )} (q,p) = \overline{\rho _2} |_{\text {Fix}(\sigma )} (q,p) \end{aligned}$$

are the two linear anti-symplectic involutions known from the planar problem. In fact, their product is the symplectic symmetry −id, and they correspond to the reflections about the $q_1$- resp. $q_2$-axes, i.e., it is not possible to say whether we are going to the sun or away from it. Together with id, these four linear symmetries in the planar problem form a Klein four-group $\mathbb {Z}_2 \times \mathbb {Z}_2$. In Aydin (2023) it is shown that these are the only linear symmetries in Hill’s system.

Special form of the reduced monodromy and the signatures Let q be a periodic orbit, not necessarily a planar one, which is invariant under an anti-symplectic symmetry $\rho$. Let the starting point $q_0 \in \text {Fix}(\rho )$. The differential $d \rho (q_0)$ induces an anti-symplectic involution, i.e., the Hamiltonian vector field $X_H$ is anti-invariant under $\rho$. In other words, this differential conjugate the monodromy with its inverse, i.e.,

$$\begin{aligned} d \rho (q_0) \circ d \varphi _H^{T_q} (q_0) \circ d \rho (q_0) = \big ( d \varphi _H^{T_q} (q_0) \big )^{-1}. \end{aligned}$$

(5)

Furthermore, the tangent space at $q_0$ decomposes into a Lagrangian splitting

$$\begin{aligned} T_{q_0}\text {Fix}(\rho ) \oplus E_{-1}\big ( d \rho (q_0) \big ), \end{aligned}$$

meaning that the two eigenspaces are Lagrangian submanifolds. We call a symplectic basis with respect to this Lagrangian splitting a “Lagrangian basis", determined by bases $(v_1,v_2,v_3)$ of $T_{q_0}\text {Fix}(\rho )$ and $(w_1,w_2,w_3)$ of $E_{-1}\big ( d \rho (q_0) \big )$ such that $\omega _{q_0}(v_i,w_j) = \delta _{ij}$ for $i,j=1,2,3$. Then the differential $d \rho (q_0)$ is represented by the standard anti-symplectic involution $\begin{pmatrix} I_3 &{} 0\\ 0 &{} - I_3 \end{pmatrix}$. By using the identity (5), the monodromy is of the form

$$\begin{aligned} d \varphi _H^{T_q} (q_0) \in \left\{ \begin{pmatrix} A &{} B\\ C &{} A^T \end{pmatrix} :B, C, CA, AB \text { are symmetric and } A^2 - BC = I_3 \right\} =: \widetilde{\text {Sp}}(3). \end{aligned}$$

For the reduced monodromy, a Lagrangian splitting of the induced 4-dimensional symplectic vector space is given by

$$\begin{aligned} T_{q_0}\Sigma / (\text {ker}\omega _{q_0} | _{T_{q_0}\Sigma }) = \big ( T_{q_0} \text {Fix}(\rho | _{\Sigma }) \big ) \oplus \Big ( E_{-1} \big ( d\rho |_{\Sigma } (q_0) \big ) / ( \text {ker}\omega _{q_0} | _{T_{q_0}\Sigma } ) \Big ). \end{aligned}$$

The basis vectors $v_1,v_2,v_3$ and the energy condition induce two basis vectors which we denote by $\tilde{v}_1,\tilde{v}_2$. By the Steinitz exchange lemma on $w_1,w_2,w_3$ and $X_H|_{\Sigma }(q_0)$, we choose $\tilde{w}_1,\tilde{w}_2$ such that $\omega _{q_0}(\tilde{v}_i,\tilde{w}_j) = \delta _{ij}$ for $i,j=1,2$, and

$$\begin{aligned} T_{q_0} \Sigma = \langle \tilde{v}_1,\tilde{v}_{2} \rangle _{\mathbb {R}} \oplus \langle \tilde{w}_1,\tilde{w}_{2}, X_H|_{\Sigma }(q_0) \rangle _{\mathbb {R}}. \end{aligned}$$

With respect to this basis, the reduced monodromy is of the form

$$\begin{aligned} \overline{d \varphi _H^{T_q} | _{\Sigma } (q_0) } \in \left\{ \begin{pmatrix} A &{} B\\ C &{} A^T \end{pmatrix} :B, C, CA, AB \text { are symmetric and } A^2 - BC = I_2 \right\} = \widetilde{\text {Sp}}(2). \end{aligned}$$

Remark 1.7

The spectrum of the monodromy and the reduced monodromy is determined by the spectrum of the resp. matrix A. For instance,

• (i) the characteristic polynomial of a matrix from $\widetilde{\text {Sp}}(2)$ is given by

  $$\begin{aligned} \lambda ^4 - 2 \text {tr}A \lambda ^3 + (2 + 4 \det A)\lambda ^2 - 2 \text {tr}A \lambda + 1. \end{aligned}$$

• (ii) The characteristic polynomial of a matrix from $\widetilde{\text {Sp}}(1) = \left\{ \begin{pmatrix} a &{} b\\ c &{} a \end{pmatrix} :a^2 - bc = 1 \right\}$ is given by $\lambda ^2 - 2a \lambda + 1$. Note that a is the half of its trace.

Definition 1.8

Let $\lambda$ be an eigenvalue of A of multiplicity 1, v an eigenvector of A and $\tilde{v}$ an eigenvector of $A^T$ to the eigenvalue $\lambda$. The signature with respect to C of a symmetric periodic orbit is defined as the signature of $v^TCv$ and the signature with respect to B as the signature of $\tilde{v}^T B \tilde{v}$. We denote them by

$$\begin{aligned} \text {sign}_C (\lambda ) = \text {sign} (v^TCv), \quad \text {sign}_B (\lambda ) = \text {sign}(\tilde{v}^T B \tilde{v}), \end{aligned}$$

respectively.

Remark 1.9

Neither of the signatures depends on the resp. eigenvectors, since for a constant $k \in \mathbb {R}^*$,

$$\begin{aligned} \text {sign}\big ( (kv)^T C (kv) \big ) = k^2 \text {sign}(v^T C v) = \text {sign} (v^T C v) = \text {sign}_C(\lambda ). \end{aligned}$$

Remark 1.10

A symplectic basis change of the Lagrangian basis is given by the action of an invertible matrix R, which is of same dimension as the matrix A, by conjugation

$$\begin{aligned} \left( \begin{array}{ll} R &{} 0\\ 0 &{} (R^{-1})^T \end{array}\right) \left( \begin{array}{ll} A &{} B\\ C &{} A^T \end{array}\right) \left( \begin{array}{ll} R^{-1} &{} 0\\ 0 &{} R^T \end{array}\right) = \begin{pmatrix}RAR^{-1} &{} RBR^{T} \\ (R^{-1})^{T} C R^{-1} &{} (RAR^{-1})^{T}\end{pmatrix} \end{aligned}$$

Notice that the matrix A transforms as linear maps, and the matrices B and C transform as bilinear forms. Moreover, both signatures are invariant under the Lagrangian basis change, i.e., they are independent of the choice of the Lagrangian basis.

Example 1.11

(Lagrangian basis and the reduced monodromy for the families g and f) The families g and f are invariant under $\rho _1(q,p) = (q_1,-q_2,q_3,-p_1,p_2,-p_3)$ from Table 2. Let the starting point $q_0 \in \text {Fix}(\rho _1)$. Since $\rho _1$ commutes with $\sigma$, a Lagrangian basis with respect to the symplectic decomposition

$$\begin{aligned} T_{q_0}\text {Fix}(\sigma ) \oplus E_{-1} \big ( d \sigma ( q_0 ) \big ) \end{aligned}$$

into the 4-dimensional planar and 2-dimensional spatial components is given by

$$\begin{aligned} \left\{ \begin{pmatrix} 1\\ 0\\ 0\\ 0 \end{pmatrix}, \begin{pmatrix} 0\\ 0\\ 0\\ 1 \end{pmatrix}, \begin{pmatrix} 0\\ 0\\ -1\\ 0 \end{pmatrix}, \begin{pmatrix} 0\\ 1\\ 0\\ 0 \end{pmatrix} \right\} \text { and } \left\{ \begin{pmatrix} 1\\ 0 \end{pmatrix}, \begin{pmatrix} 0\\ -1 \end{pmatrix} \right\} . \end{aligned}$$

These bases yield that the monodromy is of the form

$$\begin{aligned} d \varphi _H^{T_q} (q_0) = \begin{pmatrix} A_p &{} 0 \\ 0 &{} A_s \end{pmatrix},\quad A_p \in \widetilde{\text {Sp}}(2),\quad A_s \in \widetilde{\text {Sp}}(1). \end{aligned}$$

For the planar reduced monodromy $\overline{A}_p$, we need a basis of the form

$$\begin{aligned} T_{q_0} \text {Fix}(\sigma |_{\Sigma }) = \langle \tilde{v},\tilde{w} \rangle _{\mathbb {R}} \oplus \langle X_H \vert _{\Sigma }(q_0) \rangle _{\mathbb {R}} \end{aligned}$$

such that $\omega _{q_0} (\tilde{v},\tilde{w})=1$. Note that $\omega _{q_0} \big ( \tilde{v}, X_H \vert _{\Sigma }(q_0) \big ) = \omega _{q_0}\big ( \tilde{w}, X_H \vert _{\Sigma }(q_0) \big ) = 0$. The Hamiltonian (1) implies the energy condition

$$\begin{aligned} \Delta p_2(0) = \frac{1}{p_2(0) - q_1(0)} \bigg ( \frac{- q_1(0)}{|q|^3} + 2q_1(0) + p_2(0) \bigg )\Delta q_1(0), \end{aligned}$$

(6)

hence $\Delta q_1(0)$ and $\Delta p_2(0)$ determine each other such that a first basis vector is given by $\tilde{v}= (\Delta q_1(0),0,0,\Delta p_2(0))$. For simplicity we choose $\Delta q_1(0)=1$ such that the second basis vector is given by $\tilde{w} = (0,0,-1,0)$. The Hamiltonian vector field $X_H \vert _{\Sigma } (q_0) \in \langle (0,0,-1,0),(0,1,0,0) \rangle _{\mathbb {R}}$ is of the form

$$\begin{aligned} \big ( 0, \Delta q_2(0), \Delta p_1(0), 0 \big ) = \big ( 0, \dot{q}_2(0), \dot{p}_1(0), 0 \big ), \end{aligned}$$

which is determined by the initial conditions and the Eq. (2). With this basis, the reduced monodromy is of the form

$$\begin{aligned} \overline{d \varphi _H^{T_q} | _{\Sigma } (q_0) } = \begin{pmatrix} \overline{A}_p &{} 0 \\ 0 &{} A_s \end{pmatrix},\quad \overline{A}_p, A_s \in \widetilde{\text {Sp}}(1). \end{aligned}$$

Note that scaling on the Lagrangian yields

$$\begin{aligned} \begin{pmatrix} k &{} 0\\ 0 &{} \frac{1}{k} \end{pmatrix} \begin{pmatrix} a &{} b\\ c &{} a \end{pmatrix} \begin{pmatrix} \frac{1}{k} &{} 0\\ 0 &{} k \end{pmatrix} = \begin{pmatrix} a &{} k^2 b\\ \frac{1}{k^2}c &{} a \end{pmatrix},\quad k \in \mathbb {R}^*, \end{aligned}$$

hence each of the traces is invariant as well under conjugation.

GIT quotient and the signatures Let G be a Lie group acting on a manifold M and denote by Gm the orbit through $m \in M$. The space of orbits is the quotient space M/G which is in general not a Hausdorff space. To ensure the Hausdorff property we consider the orbit closure relation on M which is defined by

$$\begin{aligned} m \sim n \quad : \Leftrightarrow \quad \overline{Gm} \cap \overline{Gn} \ne \emptyset , \end{aligned}$$

meaning that m is related to n if the closure of the orbits through m and n intersect. It is clear that this relation is reflexive and symmetric, but it is not necessarily transitive. If it is an equivalence relation, then the GIT quotient (geometric invariant theory quotient) is defined as

$$\begin{aligned} M /\!/ G:= M / \sim . \end{aligned}$$

Fig. 4

Topology of $\widetilde{\text {Sp}} (1) /\!/ \text {GL}(1,\mathbb {R})$

Example 1.12

Let $\mathbb {R}_{>0}$ acting on $\mathbb {R}$ by multiplication, then there are exactly the three orbits $\mathbb {R}^-, \{0\}$ and $\mathbb {R}^+$. It is easy to verify that on the one hand the orbit space is not Hausdorff, but on the other hand the GIT quotient is

$$\begin{aligned} \mathbb {R} /\!/ \mathbb {R}_{>0} = \{\text {pt}.\}. \end{aligned}$$

Example 1.13

Let $\text {GL}(n,\mathbb {R})$ act on $\text {Mat}(n,\mathbb {R})$ by conjugation. The GIT quotient avoids Jordan factors, therefore two matrices A, B are equivalent if and only if their characteristic polynomials are the same (see Frauenfelder and Moreno, 2022). For a matrix A let $\chi _A(\lambda ) = \lambda ^n + a_{n-1}\lambda ^{n-1} +... + a_0$ be its characteristic polynomial. Then a homeomorphism is given by

$$\begin{aligned} \text {Mat}(n,\mathbb {R}) /\!/ \text {GL}(n,\mathbb {R}) \rightarrow \mathbb {R}^n,\quad [A] \mapsto (a_{n-1},...,a_0). \end{aligned}$$

Example 1.14

For this paper the relevant GIT quotient is $\widetilde{\text {Sp}}(1) /\!/ \text {GL}(1,\mathbb {R})$, which is well studied in (Zhou (2022), pp. 25–28). Each of the positive and negative hyperbolic cases consists of two subcases, namely

Topologically, the GIT quotient $\widetilde{\text {Sp}} (1) /\!/ \text {GL}(1,\mathbb {R})$ is isomorphic to a circle with four spikes (see Fig. 4). Geometrically, the unit circle $\{ z \in \ \mathbb {C} \mid |z| = 1 \} \setminus \{ \pm 1 \}$ corresponds to equivalence classes of elliptic matrices, and each spike minus $\{ \pm 1 \}$ represents a hyperbolic subcase.

Note that the eigenvalues of the hyperbolic matrices are $e^{\pm x}$ for the pos. hyperb. and $-e^{\pm x}$ for the neg. hyperb. cases, which equal the Floquet multipliers $\lambda$ and $1/\lambda$. Furthermore, in the elliptic case, if $b<0$, then the rotation is by $\theta \in (0,\pi )$ and if $b>0$, then it is by $-\theta$, so the rotation angle equals $2\pi - \theta \in (\pi ,2\pi )$.

1.3 Bifurcation graph and results

While the beginning of the families g and f for very low energies is analytical, their continuation is numerical. In particular, by using our tools, we determine the Conley–Zehnder index of the families in the Table 3. Notice that from the second row all the families arise through bifurcation from g and f, hence they can be traced back to the families g and f.

Table 3 The families of planar and spatial periodic orbits in this paper

Table 4 The family g

Table 5 The family $g'$

Table 6 Euler characteristics before and after the emergence of the family $g'$

Fig. 5

The bifurcation graph between the 3rd cover of g, the 3rd cover of $g'$ and the 5th cover of f with the families $f_g^{(2,3)}$ (blue Family), $f_g^{(2cut,3)}$ (green Family), $f_{g'}^{(2,3)}$ (red Family) and $f_{g'}^{(2cut,3)}$ (Magenta)

In this paper we use the traditional Jacobi integral $\Gamma =-2c$, where c is the energy value given by the Hamiltonian. Our results of the family g are given in Table 4.

At the planar transition from elliptic to pos. hyperb. I, $\mu _{CZ}^p$ jumps from 3 to 2 and there bifurcates the family $g'$, whose data are collected in Table 5. The orbits of the family $g'$ are simply-symmetric with respect to the reflection at the $q_1$-axis, and by using the reflection at the $q_2$-axis one obtains its symmetric family, hence the family $g'$ appears twice.

Let us verify that this is in accordance with the Euler characteristics before and after bifurcation of $g'$. These are given in Table 6.

At the spatial transition of the family g from elliptic to pos. hyperb. II, where $\mu _{CZ}^s$ jumps from 3 to 4 (see Table 4), the family $g_{2v}$ bifurcates. Its orbits are doubly-symmetric with respect to $\rho _1$ and $\rho _2$, and by using $\sigma$ one obtains its symmetric family which is doubly-symmetric with respect to $\overline{\rho _1}$ and $\overline{\rho _2}$. They have $\mu _{CZ} = 5$. The Euler characteristics before and after bifurcation are

$$\begin{aligned} (-1)^5 = -1,\quad \quad 2 \cdot (-1)^5 + (-1)^6 = -1. \end{aligned}$$

Neither the planar nor the spatial index of the simple closed f-orbits jumps, i.e., they are planar and spatial elliptic for all times. In other words, their indices are $\mu _{CZ}^p = \mu _{CZ}^s = 1$. The smallest cover of an f-orbit from which new families of spatial orbits bifurcate, is a 5-th cover. Furthermore, in the limit case for very high energy values, we analytically show that these orbits converge to a degenerate planar retrograde periodic orbit. In this limit case all three periods (i.e., the first return time and the planar and spatial rotation angles) becomes $2\pi$, which corresponds to 365.25 days - the period of the Earth around the Sun.

The other families of planar and spatial orbits from Table 3 bifurcate from the respective iteration of the underlying planar periodic orbits. Moreover, we want to emphasize the following special bifurcation result, which is illustrated in Fig. 5. We interpret this figure as a graph and call such a graph a “bifurcation graph”.

The bifurcation graph is constructed as follows:

• (1) We draw from bottom to top in the direction of increasing energy. Each vertex corresponds to a degenerate periodic orbit and each edge to a family of periodic orbits with their (constant) Conley–Zehnder index. We distinguish two kinds of edges. The first kind corresponds to the underlying family of planar periodic orbits where the index jumps and the bifurcations happen. We draw these edges in black, vertically and shortly before and after the bifurcations. The second kind corresponds to a new family branching out from the index jump. We draw such edges coloured, and every new family gets his own colour. If there is a symmetric family, then these edges are drawn in the same colour, but dashed.

• (2) The cross stands for collision and the term “b-d” for a periodic orbit of birth-death type. In general, a periodic orbit of birth-death type is a degenerate orbit from which two families bifurcate with an index difference of 1 and into the same energy direction. Its local Floer homology and its Euler characteristic are therefore zero.

To the Fig. 5:

• (1) The branches (not dashed) correspond to the families $f_g^{(2,3)}$, $f_g^{(2cut,3)}$, $f_{g'}^{(2,3)}$ and $f_{g'}^{(2cut,3)}$ with their resp. colours. In view of the symmetry $\sigma$, these families give rise to a second bifurcation branch (dashed).

• (2) At $\Gamma = 3.876$ the index of the 3rd cover of the family g jumps from 13 to 15. At this transition the two families $f_g^{(2,3)}$ and $f_g^{(2cut,3)}$ bifurcate. Their indices are 14 and 13, respectively. The orbits of the first family are doubly-symmetric with respect to $\overline{\rho _1}$ and $\overline{\rho _2}$, hence they are invariant under $-\sigma$. They end at the value $\Gamma = 0.755$ at the 5th cover of f, and inbetween there is an index jump from 14 to 15. In the second family, the orbits are doubly-symmetric with respect to $\rho _1$ and $\rho _2$, thus they are invariant under $-\sigma$ as well. At the value $\Gamma = 3.280$ the index jumps from 13 to 14, and the orbits eventually undergo collision. Until then, this family consists of branches that bifurcate from a periodic orbit of birth-death type. The dashed branches are obtained by using $\sigma$. Note that the orbits of the symmetric family (dashed) of $f_g^{(2,3)}$ are doubly-symmetric with respect to $\rho _1$ and $\rho _2$, and the orbits of the symmetric family (dashed) of $f_g^{(2cut,3)}$ are doubly-symmetric with respect to $\overline{\rho _1}$ and $\overline{\rho _2}$.

• (3) Consider the family $g'$, where at $\Gamma = 4.347$ the index of the 3rd cover jumps from 14 to 16. At this value of $\Gamma$ the two families $f_{g'}^{(2,3)}$ and $f_{g'}^{(2cut,3)}$ bifurcate, with indices 15 resp. 14. According to (Kalantonis 2020, p. 11), the two families $f_{g'}^{(2,3)}$ and $f_{g'}^{(2cut,3)}$ terminate at the 3rd cover of the resp. planar orbit of $g'$ which is symmetric to the planar orbit of $g'$, from which they have bifurcated. These are the two not-dashed branches, respectively. We have a deeper insight:

  • (i) The orbits of the family $f_{g'}^{(2,3)}$ are simply-symmetric with respect to $\overline{\rho _1}$, and its two not-dashed branches are symmetric by $\overline{\rho _2}$. Recall that the orbits of $f_g^{(2,3)}$ are doubly-symmetric with respect to $\overline{\rho _1}$ and $\overline{\rho _2}$. Therefore, very close to the value $\Gamma = 3.274$, by comparing the initial data, and by using these symmetries and especially the indices, we conclude that the family $f_{g'}^{(2,3)}$ ends at the first index jump of the family $f_g^{(2,3)}$. This explains why they come together at the value $\Gamma = 3.274$.

  • (ii) The same happens for the family $f_{g'}^{(2cut,3)}$, namely its orbits are simply-symmetric with respect to $\rho _1$ and its two not-dashed branches are symmetric by $\rho _2$. Recall that the orbits of $f_g^{(2cut,3)}$ are doubly-symmetric with respect to $\rho _1$ and $\rho _2$. Hence very close to the value $\Gamma = 3.280$, by comparing the initial data, and by using these symmetries and especially the indices, we obtain that $f_{g'}^{(2cut,3)}$ ends at the first index jump of the family $f_g^{(2cut,3)}$ (at the value $\Gamma = 3.280$).

  • (iii) Each family yields the bifurcation of a second branch (dashed) by using $\sigma$. Furthermore, note that the symmetry properties of the orbits of the spatial families $f_{g'}^{(2,3)}$ compared to $f_g^{(2,3)}$, and of $f_{g'}^{(2cut,3)}$ compared to $f_g^{(2cut,3)}$, are similar to the symmetry properties of the orbits of the planar family $g'$ compared to g.

In particular, the bifurcation graph organizes the local bifurcations and thereby helps to check the Euler characteristic of the local Floer homology groups. For instance,

• (i) at the value $\Gamma = 3.876$ at $g^3$ it is $(-1)^{13} = -1$ before, and $2\cdot (-1)^{13} + 2\cdot (-1)^{14} + (-1)^{15} = -1$ after bifurcation.

• (ii) At the value $\Gamma = 0.755$ at $f^5$ it is $2\cdot (-1)^{15} + (-1)^{16} = -1$ before, and $(-1)^{14} = 1$ after bifurcation. Here we see that at this bifurcation point there are still undiscovered families branching out from $f^5$.

In Sect. 2 we present the data for our numerical results for all the families from Table 3. They consist of all relevant data such as the initial data, the Floquet multipliers, the signatures, the indices and the periods. We also plot these periodic orbits in the configuration space and give overviews in form of bifurcation graphs such as in the Fig. 5.

Some of our numerical computations for symmetric planar and spatial periodic orbits, including explicit Python codes, can be found in Aydin (2022).

Fig. 6

The family g

2 Data and detailed results

2.1 Planar direct periodic orbits

2.1.1 The family g

Our first plot in Fig. 6 is similar to the well-known pictures from (Hill 1878, p. 261) and (Hénon 1969, p. 228). It goes until the energy 2.55788, which is the last value with a periodic orbit found by Hill. However, Hénon (1969) found further ones for higher energy values. These orbits are all doubly-symmetric with respect to $\rho _1$ and $\rho _2$. The data are collected in the Table 7. We read it, and all tables of this type in this section, in the direction of decreasing $\Gamma$. Recall that this corresponds to the increase of the energy.

Table 7 The family g

Fig. 7

The family $g'$

We denote by $T_s$ the synodic period which is the first return time $T_q$ expressed in days. Recall that $T_a$ is the anomalistic and $T_d$ the draconitic period. Remarkably, the orbit’s periods from the second row corresponds to the astronomical lunar periods which date back to the Babylonians.

The maximum of $q_1(0)$ is reached at about ${\Gamma }=3.75$ and the orbits come closer and closer to the earth. According to (Hénon 1969, pp. 230–234), based on numerical results, the distance $q_1(0)$ converges to 0 if the energy $\Gamma$ goes to $-\infty$. Hence in the limit there is a collision. In that case the period is $4 \pi$, thus the synodic month $T_s$ takes 730.5 days. In addition, by (Hénon 1974, p. 319), tr$(A_s)$ converges to 2 from above. Notice that the speed of the orbit increases if it is closer to the earth. Very shortly above $\Gamma = 4.49999$ the planar index $\mu _{CZ}^p$ jumps from 3 to 2 since the rotation by $\varphi _p$ goes to zero at that point. From then on the orbits are planar pos. hyperb. I. Slightly before this transition the anomalistic period is almost the synodic period. At this transition a new family of planar periodic orbits bifurcates (see the family $g'$ in the next Subsection 2.1.2). This bifurcation arises below the critical value $3^{4/3}$ and above the energy value for the Moon. Furthermore, these orbits are all spatial elliptic until shortly before the energy value $\Gamma = 1.383094$ where they become spatial pos. hyperb. II and $\varphi _s$ goes through $2 \pi$. Thus $\mu _{CZ}^s$ jumps from 3 to 4, and shortly before this change, the draconitic period is almost half of the synodic period. At this transition a new family of spatial periodic orbits bifurcates from the planar (see the family $g_{2v}$ in the Subsection 2.3.1). We note that all initial data are from Hénon (1969, 1974) and Hill (1878), except the ones for the energy values 4.278924, 3.876616, 2.073537 and 1.746370, where $\varphi _s$ is a 3th resp. 4th root of unity, which are from Kalantonis (2020).

Table 8 The family $g'$

2.1.2 The family $g'$

The family $g'$ branches out from the family g at the energy value $\Gamma =4.49999$ (see Table 7), which was discovered by Hénon (1969). At this bifurcation the double-symmetry breaks, meaning that the orbits are only symmetric with respect to $\rho _1$ (see Fig. 7). By using $\rho _2$, i.e., the reflection on the $q_2$-axis, these orbits appear twice. The data of the family $g'$ are collected in the Table 8. The family $g'$ and its symmetric family start being planar as well as spatial elliptic and its both indices start with 3. In Table 6 we checked that the Euler characteristics before and after bifurcation coincide.

In view of Table 8, we see the same behaviour as for the family g of the distances $q_1(0)$, namely there is a collision in the limit. Note that we have found the initial conditions for the $\Gamma$ value $-4.69849$ by ourselves. Since at this $\Gamma$ we have $\varphi _p=4.75$, we can imply that the planar index jumps from 3 to 4 in which we can see that $\varphi _p$ goes through $2 \pi$ and hence $\mu _{CZ}^p$ jumps from 3 to 4. The initial data for the energy values 4.435711, 4.347942, 4.242877, 4.200105, 0.063099 and $-0.081977$, where $\varphi _s$ is a 3rd resp. 4th root of unity, are from Kalantonis (2020) and the others from Hénon (1969, 1974).

Fig. 8

The family f

Table 9 The family f

2.2 Planar retrograde periodic orbits

2.2.1 The family f

Some of its orbits are plotted in Fig. 8 and its data are given in Table 9. We observe that the retrograde periodic orbits are all planar and spatial elliptic and are at larger and larger distance from the earth. Therefore the index of the simple closed orbits does not change. Moreover, the planar rotation angle $\varphi _p$ as well as the spatial one $\varphi _s$ decrease and then increase. The orbits for the $\Gamma$ values from $-0.2154$ to $-0.5269$ we have found ourselves. They show that $\varphi _s$ never becomes a 4th root of unity, hence the smallest one is a 5th one. Both rotation angles approach $2\pi$ for higher energy values. In other words, all three periods approach 365.25 days, which is the period of the Earth around the Sun. The initial data for the $\Gamma$ values 1.359293 and 0.755141, where $\varphi _s$ is a 6th resp. 5th root of unity, are from the data provided on request by Kalantonis (2020). All the others are from Hénon (2003, 1969).

The limit case Like (Hénon 1969, p. 227) for $\Gamma \rightarrow -\infty$ in the planar problem, we can neglect the gravitational force of smaller primary at the origin for a first approximation, since $q_1(0)$ increases for higher energies. Then a short calculation yields a planar solution given by

$$\begin{aligned} q_1(t) = c \cos t, \quad q_2(t)= - 2c \sin t,\quad p_1(t) = c \sin t,\quad p_2(t) = -c \cos t,\quad c \in \mathbb {R}, \end{aligned}$$

(7)

which is in q-variables an ellipse in a retrograde motion with the origin as its center, semi-major axis c and semi-minor axis $-2c$. Through the Jacobi integral $\Gamma$ one obtains the relation

$$\begin{aligned} q_1(0) = c = - \sqrt{- \Gamma }, \end{aligned}$$

(8)

which we use for Table 9, from $\Gamma = -15$ on. To obtain the Hamiltonian in the limit, for a constant $\gamma < 0$ we zoom out by the coordinate transformation

$$\begin{aligned} \phi _\gamma :T^* \mathbb {R}^3 \rightarrow T^* \mathbb {R}^3,\quad (q,p) \mapsto \big ( \sqrt{(-2 \gamma )} q, \sqrt{(-2 \gamma )} p \big ), \end{aligned}$$

which is conformally symplectic, i.e. $\phi _\gamma ^* \omega = -2 \gamma \omega$. We introduce the family of Hamiltonians

$$\begin{aligned} H_\gamma :T^* \big ( \mathbb {R} \setminus \{(0,0,0)\} \big ) \rightarrow \mathbb {R},\quad (q,p) \mapsto - \frac{1}{2 \gamma } (H \circ \phi _\gamma ) (q,p), \end{aligned}$$

and compute

$$\begin{aligned} H_\gamma (q,p) = \frac{1}{2} \big ( (p_1 + q_2)^2 + (p_2 - q_1)^2 + p_3^2 \big ) - \frac{3}{2}q_1^2 + \frac{1}{2}q_3^2 - \frac{1}{|q|2 \gamma \sqrt{(-2 \gamma )}}. \end{aligned}$$

For $\gamma \rightarrow -\infty$, $H_\gamma$ converges uniformly in the $C^{\infty }$-topology on each compact subset to

$$\begin{aligned} \widetilde{H} :T^* \mathbb {R}^3 \rightarrow \mathbb {R},\quad (q,p) \mapsto \frac{1}{2} \big ( (p_1 + q_2)^2 + (p_2 - q_1)^2 + p_3^2 \big ) - \frac{3}{2}q_1^2 + \frac{1}{2}q_3^2. \end{aligned}$$

Note that this limit Hamiltonian does not contain the gravitational force of the earth, in contrast to the spatial Hill lunar problem (1). We restrict to the planar case and consider the energy hypersurface $\Sigma := \widetilde{H}^{-1}(\frac{1}{2})$. Hence by the relation (8) and the limit solution (7) we obtain

$$\begin{aligned} c = -1,\quad q_1(t) = - \cos t,\quad q_2(t) = 2 \sin t,\quad p_1(t) = - \sin t,\quad p_2(t) = \cos t, \end{aligned}$$

with the first return time $T_q = 2 \pi$. Thus the synodic period is 365.25 days. The linearized equation is given by

$$\begin{aligned} \left\{ \begin{array}{l} { \Delta q_1(t) = c_1 \cos t + c_2 \sin t + 2c_3 } \\ { \Delta q_2(t) = -2c_1 \sin t + 2 c_2 \cos t - 3 c_3 t + c_4 } \\ { \Delta p_1(t) = c_1 \sin t - c_2 \cos t + 3 c_3 t - c_4 } \\ { \Delta p_2(t) = - c_1 \cos t - c_2 \sin t - c_3, } \end{array} \right. \end{aligned}$$

where $c_1$, $c_2$, $c_3$ and $c_4$ are constants. Note that these solutions are not periodic along the flow if $c_3 \ne 0$. The basis vectors of the tangent space at $q_0$ are of the form

$$\begin{aligned} \big ( \Delta q_1(0), \Delta q_2(0), \Delta p_1(0), \Delta p_2(0) \big ) = ( c_1 + 2c_3, 2c_2 + c_4, - c_2 - c_4, - c_1 - c_3 ). \end{aligned}$$

The energy condition (6) implies $\Delta q_1(0) = - \Delta p_2(0)$, and hence $c_3= 0$. Therefore the linearized solutions on the energy hypersurface are $2 \pi$ periodic, which means that $\overline{A}_p = \left( \begin{array}{ll} 1 &{} 0\\ 0 &{} 1 \end{array}\right)$, where the eigenvalue 1 has algebraic and geometric multiplicity 2. A real fundamental system for the spatial equation is given by $\{ \cos t, \sin t \}$, hence the $2 \pi$-periodic solutions are of the form

$$\begin{aligned} \left\{ \begin{array}{l} \Delta q_3(t) = c_1 \cos t + c_2 \sin t \\ \Delta p_3(t) = -c_1 \sin t + c_2 \cos t, \end{array}\right. \end{aligned}$$

where $c_1$ and $c_2$ are constants. In particular, we have $A_s = \left( \begin{array}{ll} 1 &{} 0\\ 0 &{} 1 \end{array}\right)$, where the eigenvalue 1 has algebraic and geometric multiplicity 2 as well. Therefore the limit orbit is planar and spatial degenerate, and the planar and spatial neighbouring orbits make exactly one complete rotation during $T_q$, which corresponds to 365.25 days.

2.2.2 Family $g_3$: planar bifurcation from a 3rd cover of f to a 3rd cover of f

At the $\Gamma$ values 0.015388 and $-1.411618$ for the family f, where $\varphi _p$ is a 3th root of unity (see Table 9), the planar index of each 3rd cover jumps from 5 to 3 resp. from 3 to 5. Before and after each transition of each 3rd cover of these two retrograde orbits, new families of planar retrograde periodic orbits bifurcate. These families were discovered by Hénon (1970); Hénon (2003) in which he named them family $g_3$. The orbits in these families do not lose the symmetry from f, i.e., they are also doubly-symmetric with respect to $\rho _1$ and $\rho _2$ (see Fig. 9). Their data are collected in Table 10.

Since the planar index of the 3rd cover of the f-orbit at 0.015388 jumps from 5 to 3 and the one of the f-orbit at $-1.411618$ from 3 to 5, and at each transition each new family is planar positive hyperbolic, at each transition each new family has $\mu _{CZ}^p = 4$. Thereby the Euler characteristics before and after each bifurcation are zero, see Table 11.

Fig. 9

The family $g_3$

Table 10 The family $g_3$

Table 11 Planar Euler characteristics for the bifurcation points between $f^3$ and $g_3$

Furthermore, the family starting at 0.015388 and ending at $-1.411618$ has constant Conley–Zehnder index, thus it forms a planar bridge between these 3rd covers. Note that this bridge is planar pos. hyperb. II and spatial elliptic.

If the energy decreases, then the family $g_3$ ends at a degenerate periodic orbit which is of birth-death type. In other words, the family $g_3$ starts from one branch of a degenerate planar periodic orbit which is of birth-death type. Recall from the introduction that a periodic orbit of birth-death type is a degenerate orbit from which two families bifurcate with an index difference of 1 and into the same energy direction. Its local Floer homology and its Euler characteristic are therefore zero.

To the family $f_g^{(2cut,3)}$: The orbits are doubly-symmetric with respect to $\rho _1$ and $\rho _2$. Some of these orbits are plotted in Fig. 16. Its symmetric family is obtained by using $\sigma$ (see the last row in Fig. 16). The data for the orbits are given in Table 16.

Fig. 10

From the spatial index jump of g (family $g_{2v}$)

Table 12 From the spatial index jump of g (family $g_{2v}$)

2.3 Spatial periodic orbits bifurcating from planar ones

2.3.1 From the spatial index jump of g

From the family g at the $\Gamma$ value 1.383094, where $\mu _{CZ}^s$ jumps from 3 to 4 (see Table 7), a new family of spatial periodic orbits bifurcates, which was found by Batkhin and Batkhina (2009) and called family $g_{2v}$. Some of its orbits are plotted in Fig. 10 and their data are collected in Table 12. Its initial data was provided on personal request by the first author of Batkhin and Batkhina (2009). These spatial orbits are doubly-symmetric with respect to $\rho _1$ and $\rho _2$, hence they start perpendicularly and hit perpendicularly the Lagrangian submanifolds

$$\begin{aligned} \text {Fix}(\rho _1) = \{ (q_1,0,q_3,0,p_2,0) \},\quad \text {Fix}(\rho _2) = \{ (0,q_2,q_3,p_1,0,0) \}. \end{aligned}$$

In view of $\rho _1 \circ \rho _2 = \rho _2 \circ \rho _1 = -\sigma$, the orbits are invariant under the symplectic involution $-\sigma$, but by using $\sigma$, these spatial orbits give raise to yet another family of spatial orbits. Since the relation $\sigma \circ \rho _i = \rho _i \circ \sigma = \overline{\rho _i}$, for $i \in \{1,2\}$, the orbits of the symmetric family are doubly-symmetric with respect to $\overline{\rho _1}$ and $\overline{\rho _2}$ (see the last row in Fig. 10).

To the family $f_{g'}^{(2cut,3)}$: The orbits are simply-symmetric with respect to $\rho _1$. Some of these orbits are plotted in Fig. 18. The 3rd row in Fig. 18 shows the symmetric orbits obtained by using $\rho _2$, which bifurcate from the planar orbit which is symmetric to $g'$. The symmetry $\sigma$ yields the symmetric family bifurcation from the same planar orbit of $g'$ (see the last row in Fig. 18). The data for the orbits are given in Table 17.

At the bifurcation point the index of g jumps from 5 to 6, and the family $g_{2v}$ and its symmetric family begin with the index 5. Therefore the Euler characteristics before and after bifurcation are

$$\begin{aligned} (-1)^5 = -1,\quad 2 \cdot (-1)^5 + (-1)^6 = -1. \end{aligned}$$

Fig. 11

g-orbit at the $\Gamma$ value 3.057471 and $g'$-orbits at the $\Gamma$ value 4.285183

Fig. 12

The bifurcation graph between the 2nd cover of $g'$ and the 2nd cover of g with the families g1v (blue) and $g_{1v}^{YOZ}$ (green)

Fig. 13

From the 2nd cover of g to the 2nd cover of $g'$ (family g1v) (blue)

Fig. 14

From $g^3$ to $f^5$ (family $f_g^{(2,3)}$)

2.3.2 The 2nd cover of g and the 2nd cover of $g'$

From the double cover of the g-orbits at the $\Gamma$ value 3.057471 the spatial index jumps from 9 to 11 (see Table 7), and from the double cover of the $g'$-orbits at the $\Gamma$ value 4.285183 the spatial index jumps from 10 to 11 (see Table 8). The underlying simple closed orbits are plotted in Fig. 11. In view of Example 1.5, in the latter there exist bad orbits, which are the double covers of the $g'$-orbits after the index jump. Recall that the bad orbits do not contribute to the local Floer homology groups nor to the Euler characteristics.

The underlying simple closed orbits of $g'$ are planar elliptic, and the spatial behaviour changes from elliptic to neg. hyperb. II with the indices $\mu _{CZ}^p(g') = \mu _{CZ}^s(g') = 3$. The indices of the double cover before and after this transition are

$$\begin{aligned} \mu _{CZ}^p(g'^2) = \mu _{CZ}^s(g'^2) = 5,\quad \quad \mu _{CZ}^p(g'^2) = 5,\quad \mu _{CZ}^s(g'^2) = 6. \end{aligned}$$

At these transitions new families of spatial symmetric periodic orbits bifurcate. In particular, two families bifurcate from the double cover of g and one family from the double cover of $g'$. All these families bifurcate after the index jump. Using the same notation as in Fig. 5 of the introduction, the bifurcation graph for this scenario is shown in Fig. 12. Note that we draw the families of bad orbits by dashed black edges, thus the dashed black edges do not contribute to the local Floer homology groups nor to the Euler characteristics.

The family g1v (blue) bifurcates from the double cover of g. It was found by Michalodimitrakis (1980) where the initial conditions for our data given in Table 13 are from. Some of its orbits are plotted in Fig. 13. If the energy increases, then the orbits end at a degenerate periodic orbit of birth-death type. The other branch bifurcation of this birth-death type periodic orbit is the branch bifurcation from the double cover of $g'$. All the orbits of the family g1v are doubly-symmetric with respect to $\overline{\rho _1}$ and $\rho _1$, therefore they start perpendicularly and hit perpendicularly

$$\begin{aligned} \text {Fix}(\overline{\rho _1}) = \{ (q_1,0,0,0,p_2,p_3) \},\quad \text {Fix}(\rho _1) = \{ (q_1,0,q_3,0,p_2,0) \}. \end{aligned}$$

Moreover, they are invariant under $\sigma$, but the symmetry $-\sigma$ yields the symmetrical family (dashed in the bifurcation graph), whose orbits are also symmetric with respect to $\rho _2$ and $\overline{\rho _2}$ (see the last row in Fig. 13).

The other family $g_{1v}^{YOZ}$ (green) bifurcating from the double cover of g was discovered by Batkhin and Batkhina (2009). The orbits are doubly-symmetric with respect to $\overline{\rho _2}$ and $\rho _2$. Note that we have not studied them further, but since at the value $\Gamma = 3.057$ the index of the double cover of g jumps from 9 to 11 and the family g1v (blue) and its symmetric family start with index 9, the family $g_{1v}^{YOZ}$ (green) and its symmetric family have to start with index 10.

2.3.3 The 3rd cover of g, the 5th cover of f and the 3rd cover of $g'$

In this subsection we collect the underlying data for the bifurcation graph in Fig. 5 and its discussion from the introduction with the families $f_g^{(2,3)}$)(blue), $f_g^{(2cut,3)}$(green), $f_{g'}^{(2,3)}$(red) and $f_{g'}^{(2cut,3)}$(magenta). All the initial data are provided on personal request from Kalantonis (2020).

To the family $f_g^{(2,3)}$: The orbits are doubly-symmetric with respect to $\overline{\rho _1}$ and $\overline{\rho _2}$. Some of its orbits are plotted in Fig. 14. Its symmetric family is obtained by using $\sigma$ (see the last row in Fig. 14). The data for the orbits are given in Table 14.

To the family $f_{g'}^{(2,3)}$: The orbits are simply-symmetric with respect to $\overline{\rho _1}$. Some of its orbits are plotted in Fig. 15. The 3rd row in Fig. 15 shows the symmetric orbit obtained by using $\overline{\rho _2}$, which bifurcates from the planar orbit which is symmetric to $g'$. The symmetry $\sigma$ yields the symmetric family bifurcation from the same planar orbit of $g'$ (see the last row in Fig. 15). The data for the orbits are given in Table 15.

Fig. 15

From the 3rd cover of $g'$ to the index jump $(14 \rightarrow 15)$ in Table 14 (family $f_{g'}^{(2,3)}$)

2.3.4 The 4th cover of g, the 6th cover of f and the 4th cover of $g'$

At the value $\Gamma = 4.435711$ the spatial index of the 4th cover of the orbit of the family $g'$ jumps from 18 to 20 (see Table 8). Moreover, at the value $\Gamma = 4.278924$ the spatial index of the 4th cover of g jumps from 17 to 19 (see Table 7). The bifurcation graph for this case is illustrated in Fig. 20.

Fig. 16

From the 3rd cover of g to collision (family $f_g^{(2cut,3)}$)

Fig. 17

From the 4th cover of $g'$ to the 4th cover of g (family $f_{g'}^{(1,4)}$)

Fig. 18

From the 3rd cover of $g'$ to the index jump $(13 \rightarrow 14)$ in Table 16 (family $f_{g'}^{(2cut,3)}$)

The three families $f_{g'}^{(1,4)}$ (blue), $f_{g'}^{(1cut,4)}$ (green) and $f_{g}^{(1,4)}$ (red) were found by Kalantonis (2020), who provided on personal request the initial data. All orbits are doubly-symmetric with respect to $\overline{\rho _1}$ and $\rho _1$, hence they are invariant under $\sigma$. Each symmetric family (dashed) is obtained by using the symmetry $-\sigma$. Note that the orbits of each symmetric family are doubly-symmetric with respect to $\overline{\rho _2}$ and $\rho _2$.

Table 13 From the 2nd cover of g to the 2nd cover of $g'$ (family g1v) (blue)

Table 14 From the 3rd cover of g to the 5th cover of f (family $f_g^{(2,3)}$)

Table 15 From the 3rd cover of $g'$ to the index jump $(14 \rightarrow 15)$ in Table 14 (family $f_{g'}^{(2,3)}$)

To the family $f_{g'}^{(1,4)}$: It consists of two branches bifurcating respectively from the 4th cover of $g'$ at the value $\Gamma = 4.435$ and from the 4th cover of g at the value $\Gamma = 4.278$. The two branches meet at the value $\Gamma = 4.066$ at a degenerate orbit of birth-death type. Some orbits of the family $f_{g'}^{(1,4)}$ are plotted in Fig. 17, where the last row shows a symmetric orbit. The data for the orbits are collected in Table 18.

To the family $f_{g'}^{(1cut,4)}$: This family bifurcating from the 4th cover of $g'$ at the value $\Gamma = 4.435$ ends planar at the 6th cover of the retrograde orbit at the value $\Gamma = 1.359$. Note that inbetween there are two index jumps. In view of Table 9, the index of $f^6$ at the value $\Gamma = 1.359$ jumps from 20 to 18. At this transition, the Euler characteristics show that there are still undiscovered families branching out from $f^6$. Some of the orbits of the family $f_{g'}^{(1cut,4)}$ are plotted in Fig. 19, where the last row shows a symmetric orbit, and the data are collected in Table 19.

Table 16 From the 3rd cover of g to collision (family $f_g^{(2cut,3)}$)

Table 17 From the 3rd cover of $g'$ to the index jump $(13 \rightarrow 14)$ in Table 16 (family $f_{g'}^{(2cut,3)}$)

Fig. 19

From the 4th cover of $g'$ to the 6th cover of f (family $f_{g'}^{(1cut,4)}$)

To the family $f_{g}^{(1,4)}$: We have not studied it further, but since at the value $\Gamma = 4.278$ the index of the 4th cover of g jumps from 17 to 19 and the family $f_{g'}^{(1,4)}$ and its symmetric family start with index 17, the family $f_{g}^{(1,4)}$ and its symmetric family have to start with index 18.

Table 18 From the 4th cover of $g'$ to the 4th cover of g (family $f_{g'}^{(1,4)}$)

Fig. 20

The bifurcation graph between the 4th cover of $g'$, the 4th cover of g and the 6th cover of f with the families $f_{g'}^{(1,4)}$, $f_{g'}^{(1cut,4)}$ and $f_{g}^{(1,4)}$

Table 19 From the 4th cover of $g'$ to the 6th cover of f (family $f_{g'}^{(1cut,4)}$)