1 Introduction

Railway track infrastructure consists of linear positioned objects; therefore, the reliability and availability of the whole railway operation is heavily dependent on the reliability of the local parts. The railway track and train interaction in the local irregularities like rail discontinuities (crossings, switches, turnouts) or rail support irregularities (bridge transition zones, ballastless track connection) cause dynamic loadings. The local irregularities are unfavourable for the long-term performance of the track structure due to the appearance of track local instabilities such as differential track settlements, sleeper voids, ballast pulverization and mud pumping. The local instabilities require enormous maintenance costs and severely influence the overall railway operation [1]. The ballast layer and the related track geometry are the most rapidly deteriorated in the zones of local instabilities [2].

The dynamic interaction in zones of local instabilities, and especially transition zones, is presented in many studies [3,4,5,6,7,8,9,10,11,12,13,14,15,16,17,18,19,20]. Measurements and simulations of the dynamic responses of a bridge and embankment transition zone on a heavy haul railway line are presented in [4]. In situ accelerations on a typical bridge–embankment combination were measured and a three-dimensional finite element model (3D FEM) of a moving load was developed. The study concludes the increase in dynamic loadings to 300 kN and the fundamental frequency of acceleration between 30 and 35 Hz. Experimental analysis, short- and long-term numerical analysis and design variation analysis in the transition zone are presented in papers [6, 8]. The study results show the amplification of wheel loads and ballast stress near the bridge and the increase in dynamic responses with differential settlement and train velocity. The study [11] presents an estimation of the influence of the variable stiffness section on the conditions of track and rolling stock interaction in a zone of local irregularity. The effects of reduced mass, stiffness and dissipation factors are studied. The article [12] investigates by using a FEM model the effects of approach slab length, thickness, and travel speed on the transition zone. Results show that an increase in the cruising speed from 120 to 300 km/h increases deformation by 25%. Climatic factors like freezing can have the consequence that the structure may be affected by the resulting reduction of resistance of these layers to traffic load as presented in [13]. The authors in the paper [17] indicate that the transition zones cause a shock wave when the train passes, which can cause undesirable effects on the stability of its construction, durability, and passengers’ comfort. The research deals with the analysis of the long-term settlement processes in transition zones. The paper [14] presents a critical review of various problems associated with transition zones and the measures adopted to mitigate them. Recommendations for the performance of the ballasted track at transitions are also presented after reviewing the various design approaches. Various examples of anomalies on ballast railway tracks resulting in accelerated degradation of railway sleepers and ballast layer are described in the paper [15]. Based on the experimentally obtained data, a simplified numerical model which interconnects the individual measured and estimated parameters of the railway track and the processes of further degradation has been developed using an artificial neural network. The results reveal that the presence of welds is the single most important influential parameter, which accounts for around 40%. The remaining influential parameters are of the same order and amount to around 10% each.

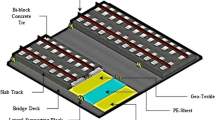
Various countermeasures are used to reduce the intensity of development or to stabilize the development of track geometry in zones of local instabilities (Fig. 1). The methods can be categorized into two groups: ballast loading reduction one and ballast layer and the subgrade resilience improvement group.

Fig. 1
figure 1

Approaches for track geometry stabilization in zones of local instabilities

Full size image

The most frequently applied approaches for improvement of resilience are glueing [21] and subgrade reinforcement [22]. The general disadvantage of the approaches for the case of transition zones involves the difficulty in controlling of the smooth transition between the stable zone of the bridge of ballastless track and the floating ballasted track that is subjected to the long-term settlements.

Another group of methods is based on ballast pressure control. The ballast loading could be controlled in different ways. The simplest way consists of the improvement of support stiffness that can be achieved with fastenings, under-sleeper pads or under-ballast mats. The support stiffness variation causes a redistribution of the loading transmission from the rail to the ballast layer. Many studies present estimation of the track geometry stabilization in transition zones [3,4,5,6,7,8,9, 12,13,14, 16,17,18,19,20]. The theoretical study [5] presented an influence estimation of gradual stiffness transition, such as the use of gradual pad stiffness, long sleepers and auxiliary rail in the transition zone on the subgrade loading, accelerations and deflections. The study states that the auxiliary rail installation results in a high rate of track dynamic improvement that is most evident in the reduction of vertical stress in the subgrade from 20 to 14 kPa. The increase of sleeper length to 3.65 m had no influence on the subgrade pressure, and the subgrade improvement had a negative influence. However, the influence of the auxiliary rail was overestimated due to high fastening stiffness and the stiff connection of the auxiliary rail to the sleepers. In contrast to the study in the paper [6], the long and wide sleepers are recommended from the performance point of view since they provide the largest reduction of the maximum of the ballast stress. In the study [7], numerical models with and without under-sleeper pad (USP) components are built for the selected transition zone. Numerical models simulate dynamic load from train passages and impact hammer load. It was observed from simulation results that the integration of the USP component into the track provides an approximately 25% decrease in ballast acceleration. The paper [9] proposed “…the world’s first investigation…” of the application of baseplate fastening systems to fix the transition zone problem. The numerical simulations show that the 400 MN/m/m2 pad stiffness provides the best solution as the slab track stiffness can be equalized to the stiffness of ballasted track. However, the conclusion is based only on the elastic differential settlements and no cost-effectiveness comparison to the conventional fastening types is presented. The study [16] investigates the behaviour of an integral abutment bridge with a transition zone subjected to train loading. The results indicate that the trapezoidal approach slab influences the track displacement significantly. The transition zone thickness and material properties of the backfill have a greater effect on the overall track response. Sleeper shape and size could be used to control ballast loading, as described in [23, 24]. However, due to constraints of maintenance requirements, the width of the sleeper cannot be significantly changed and the increase of the sleeper length is not appropriate, e.g., in ballasted bridges. Additionally, the long-term behaviour of the track with different sleeper forms cannot be as good as the ballast loading transmission control that could cause the appearance of additional local instabilities.

The most appropriate method of track geometry stabilization in transition zones is based on control of track bending stiffness. This method, in contrast to others, causes smoothing of track inhomogeneous settlements along the track. The track bending stiffness causes the “automatic” redistribution of rail support loading while differential settlements, thus smoothing the track geometry. This property is especially important for transition zones. The track bending stiffness can be improved by application of additional bending elements that are usually attached to the sleepers. The most frequently used are auxiliary rails over the transition zones. A similar solution is presented by Dynatrans [3] (Fig. 2, left) that applies up to four auxiliary rails together with under-sleeper pads, and variable sleeper length to control the transition zone from a ballasted track to a ballastless one. A quite different solution is presented by V-Tras [10] (Fig. 2, right) in which the bending stiffness is formed by under-sleeper beams with variable longitudinal stiffness. The structure is pivotally connected to the bridge slab track and smooths differential track settlements over 8 (24) m.

Fig. 2
figure 2

Solutions for stabilization of track geometry in transition zones: Left—Dynatrans [3], Right—V-Tras [10]

Full size image

The track bending stiffness improvement methods can be classified by the bending stiffness location (Table 1): connected to the sleepers’ top surface, under the sleepers and in the rail stiffness. The last one can be theoretically grouped as the increased rail bending stiffness, e.g., in crossings and CWR breathing devices. The methods have different advantages and shortcomings that usually refer to their efficiency to stabilize track geometry and the corresponding cost aspects.

Table 1 Methods of bending stiffness improvement for zones with local instabilities
Full size table

The efficiency of the bending stiffness methods depends on the local position of the bending reinforcement and the spring-elastic connections in the track. The importance of the bending stiffness for the ballasted tracks is highlighted in the study [25]. Optimization of transition areas between ballastless track and ballasted track in the area of the Tunnel Turecky Vrch is presented in the study [18]. The study is based on the diagnostics data and mathematical modelling of the transition areas. The application of the stiffening rails and with the use of under-sleeper pads and the track ballast of variable thickness is estimated. The estimation criterion was rail vertical displacement. The application of stiffening rails was not considered as a significant improvement. Application of the under-sleeper pads has proved to be an efficient way of optimization of the transition area. The study [3] that investigated the solution Dynatrans (Fig. 2) shows a 14.12% reduction in rail vertical displacement for the solution compared with the standard solution with one auxiliary rail. A reduction of 3.70% in positive shear stress and 1.49% of negative shear stress is reported with Dynatrans over the common solution. However, the minimum vertical accelerations when the train travelled from the ballast track to slab track were 22.55% higher in the Dynatrans solution. This study [19] presents the research of spatial variance in the railway track support stiffness to the expected long-term track degradation. The study focuses on a locally reduced support stiffness (hanging sleeper) along the track that usually appears in zones of local instabilities. It is stated that rail pad stiffness has a significant effect; especially for high-speed tracks, a high pad stiffness is very unfavourable. Other influencing factors are degraded sleeper supports, sleeper spacing and the rail cross-sectional properties. Application of 60E1 rail profile instead of 54E1 rail may reduce energy dissipation by roughly 30% on a high-speed track. The paper [20] presents a numerical analysis of dynamic track behaviour at transition zones using the 3D finite element method (3D FEM). The model takes into account auxiliary rails and loaded rails as stiffly connected to the sleepers without fastenings. The model was validated experimentally. It is qualitatively stated that using two auxiliary rails can improve the dynamic characteristics of the track. However, a comparison of the experimental rail displacements and the validated displacements shows almost no difference between the cases with and without auxiliary rails. Also the simulation of the ballast compressive stress shows less than a 5% reduction by the application of auxiliary rails.

The reviewed studies show that there are many approaches for the stabilization of track geometry in transition zones; however, the efficiency of the methods cannot be directly compared due to many influence factors. Moreover, the influence of certain countermeasures is not clear. For example, some authors indicate the favourable influence of the auxiliary rails, while others claim a rather low influence. Most of the studies are based on complex dynamic FEM simulations as a replacement for the experiments. Nevertheless, the simulation results are analysed phenomenologically without deep analysis and explanation of the reasons. The application of under-sleeper beams and their efficiency is not presented in the papers. Thus, there is a need for systematic analysis of the factor influence by application of various approaches to bending and elastic improvement of track.

The aim of the present paper is a comparison of the different reinforcement methods that are based on bending element applications, namely auxiliary beams and beam structures under sleepers. The static interaction is considered to gain clear insight into the factor influence. The influence of bending stiffness and track support stiffness on track loading transmission is analysed for a simple analytical model with free model parameters and for a constrained one with constant track stiffness. The influence of bending elements such as auxiliary beam and under-sleeper ones on loading transmission is estimated with the help of 1D FEM numerical model that takes into account vertical stiffness variation and shear interaction. A criterion of equivalent bending stiffness is proposed to compare different technical solutions for track stabilization.

2 Analysis of Factors Influencing Track Support Loading Transmission for a Simple Beam on Elastic Foundation

2.1 Influence of Track Bending Stiffness and Support Stiffness on Track Support Loading Transmission

Train wheel loading on rail is distributed on the rail support due to rail bending stiffness. Therefore, the sleeper loading is reduced compared to rail loading, and the reduction is about 40% for standard conditions [2]. Simple analytical relations are used to explore the main factors of track support loading transmission and estimate the influence of track stabilization by bending stiffness improvement.

The analysis was performed for the following conditions. The track bed modulus on old lines is between 0.05 and 0.15 N/mm3, on new lines 0.3–0.4 N/mm3 [2]. Thus, two limit values are considered:

  • stiff track bed: \(c_{{{\text{stB}}}}\) = 350 MN/m3,

  • soft track bed: \(c_{{{\text{sfB}}}}\) = 150 MN/m3.

Effective contact area of the sleeper: \(A_{{{\text{Scw}}}}\) = 0.57 m2 and per rail: \(A_{{{\text{Scw}}}}\) = 0.285 m2.

This results in the following spring stiffness of rail supports:

  • stiff track bed: CB = cB AScw = 350 · 0.285 = 99.75 kN/m,

  • soft track bed: CB = cB   AScw = 150 · 0.285 = 42.75 kN/m.

The rail pad type is considered Zw700 with stiffness CZw = 70 kN/m.

The resulting stiffness of sleeper support is as follows:

  • stiff track bed: \(C_{{{\text{res}},{\text{h}}}} = \frac{{C_{Zw} C_{{\text{B}}} }}{{C_{Zw} + C_{{\text{B}}} }} = \frac{99.75 \cdot 70}{{99.75 + 70}} = 41.1\) kN/m

  • soft track bed: \(C_{{{\text{res}},{\text{w}}}} = \frac{42.75 \cdot 70}{{42.75 + 70}} = 26.5\) kN/m

The maximal loading on a sleeper under rail loading \(P_{{\text{R}}}\) is calculated using the analytical expression:

$$P_{{{\text{Sl}}, \max }} = \frac{{P_{{\text{R}}} \cdot k \cdot l}}{2},$$
(1)

where \(k\)—coefficient of the relative stiffness of rail support and rail bending:

$$k = \sqrt[4]{{\frac{{U_{z} }}{{4{\text{EI}}}}}},$$
(2)

where EI = 6.10 MN m2—bending stiffness of a rail UIC 60; l = 0.6 m—sleeper spacing; \(U_{z} = C_{{{\text{res}}}} /l\)—stiffness of 1 m rail support.

The influence of the track bending stiffness improvement, which is shown in Fig. 3, demonstrates that the four times bending stiffness increase causes sleeper loading reduction from 39 to 27%. Therefore, the steps of reduction of the relative loading rapidly decrease with the increase of bending stiffness from 1EI to 4EI. Thus, the decrement of the relative loading amounts to less than 2.5% for an increase in bending stiffness of more than three times. Despite the seemingly low effectiveness of support loading reduction by the bending stiffness increase, it is not possible to conclude the proportional influence of the bending stiffness on differential ballast settlements and track irregularity development.

Fig. 3
figure 3

Influence of rail bending stiffness on rail support loading distribution

Full size image

The loading transmission from rail to sleeper depends on both track bending stiffness EI and support stiffness U. The influence of the factors on the loading according to (1, 2) is equivalent: reduction of the sleeper loading can be equally reached by the increase in the bending stiffness EI or the equivalent decrease of the support stiffness U (Fig. 4). However, the possible variation range of the factors is practically limited. The track support stiffness can be controlled by fastening stiffness and additionally by sleeper pads. Figure 4, which presents a comparison of different support stiffness, shows that it is possible to reduce the loading transmission from 0.4 for the standard solution to about 0.3 for the most elastic support. The factor of track bending stiffness could be controlled much better by application of different technical solutions from simple auxiliary rails to under-sleeper beams that due to high structural stiffness provide many times increase of the track bending stiffness.

Fig. 4
figure 4

Influence of the track support stiffness and track bending stiffness on the transmission of the loading on track support

Full size image

2.2 Influence of Bending Stiffness on Support Loading for Constant Track Stiffness

The track bending stiffness and track support stiffness are considered in (1, 2) and Fig. 4 as equivalent factors that can be independently controlled. However, these factors are not actually independent in the case of track stabilization solutions at local zones such as transition zones where the overall track stiffness should be controlled. Assuming that the track stiffness is constant or prescribed and the bending stiffness is a free parameter, the support stiffness can be expressed from both of them. The track stiffness \(c_{{{\text{tr}}}}\) is calculated from the maximal rail deflection \(Z_{{{\text{R}}, \max }}\) and rail loading \(P_{{\text{R}}}\) that could be replaced by the coefficient of the relative stiffness k and the stiffness of rail support \(U_{z}\):

$$Z_{{{\text{R}}, \max }} = \frac{{P_{{\text{R}}} \cdot k}}{{2 \cdot U_{z} }},$$
(3)
$$c_{{{\text{tr}}}} = \frac{{P_{{\text{R}}} }}{{Z_{{{\text{R}}, \max }} }} = \frac{{2 \cdot U_{z} }}{k} = {\text{const}}.$$
(4)

The exclusion of the parameter \(k\) from the relation (2) provides the functional relation of the rail support stiffness \(U_{z}^{{}}\) from the track stiffness \(c_{{{\text{tr}}}}\) and track bending stiffness:

$$k = \sqrt[4]{{\frac{{U_{z} \left( {c_{{{\text{tr}}}} } \right)}}{{4{\text{EI}}}}}},$$
(5)
$$c_{{{\text{tr}}}} = \sqrt[4]{{64{\text{EI}} \cdot U_{z}^{3} }},$$
(6)
$$U_{z}^{{}} \left( {c_{{{\text{tr}}}} } \right) = \sqrt[3]{{\frac{{c_{{{\text{tr}}}}^{4} }}{{64{\text{EI}}}}}}.$$
(7)

The application of constant track stiffness constraint affects loading transmission. A comparison of the constant support stiffness approach with the constant track stiffness approach is presented in Fig. 5. The increase in bending stiffness causes the reduction in the sleeper loading transmission and at the same time the increase in track stiffness. Thus, to preserve the constant track stiffness, the rail support stiffness should be reduced, which again causes the reduction of the relative loading. Figure 5 shows that the increase in bending stiffness from 1EI to 4EI requires a reduction in track support stiffness from 41 to 26 kN/mm for the stiff subgrade. The reduction of track support stiffness is possible by the application of two times less stiff fastenings with vertical stiffness 50 kN/mm that is located in practical range Fig. 4. The combined effect of bending stiffness increases up to 4EI, and the dependent track support stiffness reduction is 22–24% of the loading transmission from the rail to the track support. The effect can also be expressed as equivalent to a 40–50% increase in bending stiffness without changing the sleeper support.

Fig. 5
figure 5

Rail support loading transmission (top) and the necessary rail support stiffness (bottom) for the constant track stiffness case

Full size image

3 Influence of Bending Stiffness Location Over and Under the Sleeper on Ballast Loading

The presented in the previous section estimation of the possibilities of sleeper and ballast loading reduction by bending and support stiffness control is based on a simple analytical model that assumes allocation of the bending stiffness in the rail axis. However, the scope of the rail bending stiffness control is limited. Practical approaches by bending stiffness improvement are based on the allocation of bending elements on a sleeper (auxiliary rail) or under sleepers such as the V-Tras solution. A one-dimensional FEM model is developed to estimate the influence of bending stiffness allocation in different vertical locations of the track. The model (Fig. 6) consists of two Euler beams with different vertical locations that are elastically connected with separate springs. The beams correspond to the rail and the auxiliary bending element under the sleepers or over them. The elastic springs correspond to fastening stiffness \(c_{{\text{f}}}\), ballast and subgrade stiffness \(c_{{{\text{su}}}}\) and the stiffness of the elements connecting the sleeper with auxiliary bending elements: under-sleeper beam \(c_{{{\text{sb}}}}\) and auxiliary rail \(c_{{{\text{aux}}}}\). The model additionally takes into account the structural stiffness due to the shear interaction between the rail and under-sleeper beam that are located with vertical distance \(h.\)

Fig. 6
figure 6

Beam FEM model of multilayer beam for calculation of ballast loading transmission (equivalent stiffness)

Full size image

The model with two beams (Fig. 6) can be configured for two cases:

  • Case 1—the auxiliary rail (upper beam) is elastically connected and the loaded rail (bottom beam) that is located on support with stiffness including fastenings, sleepers, ballast and subgrade.

  • Case 2—the loaded rail (upper beam) is elastically connected to the under-sleeper beam (bottom one) that is supported with ballast and subgrade stiffness. The connection between the two beams corresponds to fastening and sleeper with an under-sleeper beam connection.

The model is based on 1D FEM beam elements (Fig. 7) that take into account vertical displacements \(z\) and angles \(\theta\) of the beam in each node.

Fig. 7
figure 7

Finite element formulations for a 2D beam element

Full size image

The stiffness matrix of one bending element is following:

$$K_{{}} = \frac{{2{\text{EI}}}}{{l^{3} }}\left[ {\begin{array}{*{20}c} 6 & { - 6} & {3l} & {3l} \\ { - 6} & 6 & { - 3l} & { - 3l} \\ {3l} & { - 3l} & {2l^{2} } & {l^{2} } \\ {3l} & { - 3l} & {l^{2} } & {2l^{2} } \\ \end{array} } \right]\;w = \left\{ {\begin{array}{*{20}c} {\begin{array}{*{20}c} {z_{1} } \\ {z_{2} } \\ {\theta_{1} } \\ \end{array} } \\ {\theta_{2} } \\ \end{array} } \right\}$$
(8)

where \(l\)—length of the element; EI—bending stiffness of the element.

The beam elements are connected with other beams with spring elements. The stiffness matrix for a fragment of two pairs of bending elements with springs between them (Fig. 8) corresponds to the blocks of stiffness matrixes (9–14) that take into account bending deflections, angles, vertical spring connection and shear interaction.

Fig. 8
figure 8

Elastic vertical and shear interactions between layers

Full size image

The stiffness matrix block that corresponds to the beam vertical deflections (Fig. 8):

$$K_{{{\text{b}}z}} = \frac{2}{{l^{3} }}\left[ {\begin{array}{*{20}c} {6{\text{EI}}_{1} } & { - 6{\text{EI}}_{1} } & 0 & 0 & 0 & 0 \\ { - 6{\text{EI}}_{1} } & {12{\text{EI}}_{1} } & { - 6{\text{EI}}_{1} } & 0 & 0 & 0 \\ 0 & { - 6{\text{EI}}_{1} } & {6{\text{EI}}_{1} } & 0 & 0 & 0 \\ 0 & 0 & 0 & {6{\text{EI}}_{2} } & { - 6{\text{EI}}_{2} } & 0 \\ 0 & 0 & 0 & { - 6{\text{EI}}_{2} } & {12{\text{EI}}_{2} } & { - 6{\text{EI}}_{2} } \\ 0 & 0 & 0 & 0 & { - 6{\text{EI}}_{2} } & {6{\text{EI}}_{2} } \\ \end{array} } \right]\;w_{z} = \left\{ {\begin{array}{*{20}c} {\begin{array}{*{20}c} {z_{1} } \\ {z_{2} } \\ {z_{3} } \\ \end{array} } \\ {z_{4} } \\ {z_{5} } \\ {z_{6} } \\ \end{array} } \right\}$$
(9)

The stiffness matrix block that corresponds to the beam angles of the fragment:

$$K_{{{\text{b}}\theta }} = \frac{2}{l}\left[ {\begin{array}{*{20}c} {2{\text{EI}}_{1} } & {{\text{EI}}_{1} } & 0 & 0 & 0 & 0 \\ {{\text{EI}}_{1} } & {4{\text{EI}}_{1} } & {{\text{EI}}_{1} } & 0 & 0 & 0 \\ 0 & {{\text{EI}}_{1} } & {2{\text{EI}}_{1} } & 0 & 0 & 0 \\ 0 & 0 & 0 & {2{\text{EI}}_{2} } & {{\text{EI}}_{2} } & 0 \\ 0 & 0 & 0 & {{\text{EI}}_{2} } & {4{\text{EI}}_{2} } & {{\text{EI}}_{2} } \\ 0 & 0 & 0 & 0 & {{\text{EI}}_{2} } & {2{\text{EI}}_{2} } \\ \end{array} } \right]\;w_{\theta } = \left\{ {\begin{array}{*{20}c} {\begin{array}{*{20}c} {\theta_{1} } \\ {\theta_{2} } \\ {\theta_{3} } \\ \end{array} } \\ {\theta_{4} } \\ {\theta_{5} } \\ {\theta_{6} } \\ \end{array} } \right\}$$
(10)

The diagonal blocks of deflection-angle interactions are presented with the following matrixes:

$$K_{{\text{b,intUp}}} = \frac{2}{{l^{2} }}\left[ {\begin{array}{*{20}c} {3{\text{EI}}_{1} } & {3{\text{EI}}_{1} } & 0 & 0 & 0 & 0 \\ { - 3{\text{EI}}_{1} } & 0 & {3{\text{EI}}_{1} } & 0 & 0 & 0 \\ 0 & { - 3{\text{EI}}_{1} } & { - 3{\text{EI}}_{1} } & 0 & 0 & 0 \\ 0 & 0 & 0 & {3{\text{EI}}_{2} } & {3{\text{EI}}_{2} } & 0 \\ 0 & 0 & 0 & { - 3{\text{EI}}_{2} } & 0 & {3{\text{EI}}_{2} } \\ 0 & 0 & 0 & 0 & { - 3{\text{EI}}_{2} } & { - 3{\text{EI}}_{2} } \\ \end{array} } \right]\;w_{z,\theta } = \left\{ {\begin{array}{*{20}c} {\begin{array}{*{20}c} {z_{1} } \\ {z_{2} } \\ {z_{3} } \\ \end{array} } \\ {z_{4} } \\ {z_{5} } \\ {z_{6} } \\ \end{array} } \right\}$$
(11)
$$K_{{\text{b,intDn}}} = \frac{2}{{l^{2} }}\left[ {\begin{array}{*{20}c} {3{\text{EI}}_{1} } & { - 3{\text{EI}}_{1} } & 0 & 0 & 0 & 0 \\ {3{\text{EI}}_{1} } & 0 & { - 3{\text{EI}}_{1} } & 0 & 0 & 0 \\ 0 & {3{\text{EI}}_{1} } & { - 3{\text{EI}}_{1} } & 0 & 0 & 0 \\ 0 & 0 & 0 & {3{\text{EI}}_{2} } & { - 3{\text{EI}}_{2} } & 0 \\ 0 & 0 & 0 & {3{\text{EI}}_{2} } & 0 & { - 3{\text{EI}}_{2} } \\ 0 & 0 & 0 & 0 & {3{\text{EI}}_{2} } & { - 3{\text{EI}}_{2} } \\ \end{array} } \right]\;w_{\theta ,z} = \left\{ {\begin{array}{*{20}c} {\begin{array}{*{20}c} {\theta_{1} } \\ {\theta_{2} } \\ {\theta_{3} } \\ \end{array} } \\ {\theta_{4} } \\ {\theta_{5} } \\ {\theta_{6} } \\ \end{array} } \right\}$$
(12)

The vertical springs with the stiffness \(k_{{\text{v}}}\) that connect upper and bottom beams:

$$K_{{\text{v}}} = k_{{\text{v}}} \left[ {\begin{array}{*{20}c} 1 & 0 & 0 & { - 1} & 0 & 0 \\ 0 & 1 & 0 & 0 & { - 1} & 0 \\ 0 & 0 & 1 & 0 & 0 & { - 1} \\ { - 1} & 0 & 0 & 1 & 0 & 0 \\ 0 & { - 1} & 0 & 0 & 1 & 0 \\ 0 & 0 & { - 1} & 0 & 0 & 1 \\ \end{array} } \right]\;w_{z} = \left\{ {\begin{array}{*{20}c} {\begin{array}{*{20}c} {z_{1} } \\ {z_{2} } \\ {z_{3} } \\ \end{array} } \\ {z_{4} } \\ {z_{5} } \\ {z_{6} } \\ \end{array} } \right\}$$
(13)

The shear interaction between two layered beams is presented by a stiffness matrix that takes into account horizontal spring stiffness \(k_{{\text{h}}}\), beam angles \(w_{\theta }\) and vertical distance between beams \(h\):

$$K_{{\text{h}}} = \frac{{k_{{\text{h}}} h^{2} }}{4}\left[ {\begin{array}{*{20}c} 1 & 0 & 0 & { - 1} & 0 & 0 \\ 0 & 1 & 0 & 0 & { - 1} & 0 \\ 0 & 0 & 1 & 0 & 0 & { - 1} \\ { - 1} & 0 & 0 & 1 & 0 & 0 \\ 0 & { - 1} & 0 & 0 & 1 & 0 \\ 0 & 0 & { - 1} & 0 & 0 & 1 \\ \end{array} } \right]\;w_{\theta } = \left\{ {\begin{array}{*{20}c} {\begin{array}{*{20}c} {\theta_{1} } \\ {\theta_{2} } \\ {\theta_{3} } \\ \end{array} } \\ {\theta_{4} } \\ {\theta_{5} } \\ {\theta_{6} } \\ \end{array} } \right\}$$
(14)

The overall matrix for the beam fragment (Fig. 9) consists of blocks (9–14):

$$K_{g} = \left[ {\begin{array}{*{20}c} {K_{{{\text{b}},z}} + K_{v} } & \vdots & {K_{{\text{b,intUp}}} } \\ \cdots & \vdots & \cdots \\ {K_{{\text{b,intDn}}} } & \vdots & {K_{{{\text{b}},\theta }} + K_{{\text{h}}} } \\ \end{array} } \right]\;w = \left\{ {\begin{array}{*{20}c} {w_{z} } \\ \ldots \\ {w_{\theta } } \\ \end{array} } \right\}$$
(15)
Fig. 9
figure 9

Relative loading on sleeper support (bottom) and reactions of AR (top) for different fastenings of AR

Full size image

The whole FEM model consists of 18 bending elements that are connected with 19 vertical and horizontal spring elements between beams and 19 spring elements under the bottom beam with 76 degrees of freedom. The model is used to explore the loading transmission from rail to rail support for the following cases.

3.1 Influence of the Bending Stiffness of Auxiliary Rail (Case 1)

The standard auxiliary rails (Case 1) are usually mounted between the rails on the sleeper upper surface by the standard elastic fastenings. Under the wheel loading, sleepers are deflected together with the auxiliary rail (AR) that causes AR reactions, which tend to distribute the deflections and support loading on more sleepers and thus to reduce loading transmission. The AR deflections are much lower than the rail deflections due to the elasticity of both fastenings. Therefore, the loading distribution properties of AR are always lower than for the ordinary rail and depend on the elasticity of the fastenings. Figure 9 presents the comparison of loading transmission (bottom) and the AR relative reactions (bottom) for different stiffness of the AR fastenings: for compressive stiffness (ZW700), for tensile stiffness of rail clips (20% of ZW700) and for the case with absolutely stiff AR fastenings. The rail fastening stiffness is constant for case ZW700.

The diagram of AR reactions (Fig. 9, top) shows that the AR reactions counteract the external loading under its location and overload the 4–6 sleepers of both sides. The maximal unloading reactions are 4% of the maximal sleeper loading for the tensile stiffness and 7% the compressive one. The application of stiff AR fastenings can improve the loading reaction to 11%. However, the influence of the AR bending stiffness on the support loading transmission is low − 37% compared to 39% for the case without AR. Application of stiff AR fastenings causes a negligible reduction in the relative loading which is explained by the much lower deflection of the sleepers than that of the loaded rail. The maximally possible effect of AR is equivalent to the case \(2\mathrm{EI}\) in Fig. 3—32%. However, it could be reached only with a stiff connection of AR and rails to the sleepers, which is practically limited by the other effects. Thus, the actual effect of the AR on elastic fastenings is equivalent to 1.2–1.4 times of track bending \(\mathrm{EI}\) increase.

3.2 Influence of the Bending Stiffness Under the Sleepers

The under-sleeper beams (Case 2) application has an advantage over the AR because the elastic connection to the sleeper additionally influences track support stiffness. Therefore, the elastic connection can be allocated between the sleeper foot and the beam and between the beam and ballast layers. These variants and additionally stiff sleeper-beam-ballast connection are compared by their influence on the loading transmission in Fig. 10. The stiffness of connecting layers is chosen to be the same as Case 1 for ZW700 to have the comparable results. The beam reactions (Fig. 10, top) are on average higher than for the same bending stiffness AR beam (Fig. 9, top); however, the highest reactions correspond to the variant of the elastic layer under the beam. It is about equal to that of the stiff connection of the AR rail with the sleepers. Nevertheless, the loading transmission for Case 2 is noticeably lower than for Case 1 with auxiliary rail: from 34% for the stiff connection with sleepers to 28% with the elastic layer under the beam. The loading transmission effect of the under-sleeper beam application with elastic connections is equivalent to the \(3\mathrm{EI}\) track bending stiffness increase (Fig. 3).

Fig. 10
figure 10

Relative loading on sleeper support (bottom) and reactions of the beam under sleeper (top) for different allocations of elastic layers

Full size image

3.3 Influence of the Bending Stiffness Under Sleeper and Structural Stiffness due to Shear Interaction Between Beams

An additional advantage of the under-sleeper beam application (Case 2) is the horizontal shear interaction between the rail and the beam, which could cause a high increase in the structural stiffness. The shear interaction depends on the horizontal stiffness \({k}_{\mathrm{h}}\) of the connecting layers and the vertical distance \(h\) between the beam axles (Fig. 6). The horizontal stiffness \({k}_{\mathrm{h}}\) is dependent on the vertical one and is usually assumed 25–50% of that. The distance \(h\) consists of the sleeper height and half of the beam heights, and is assumed for the calculation 0.46 m.

The loading transmission for the same elastic connection variants as in Fig. 10 is presented in Fig. 11. The maximal reduction of the rail support loading due to the shear interaction is 25–30%, which is equivalent to the 3–5EI track bending stiffness.

Fig. 11
figure 11

Relative loading on sleeper support (bottom) and reactions of the beam under sleeper (top) for different allocations of elastic layers and shear interaction

Full size image

3.4 Influence of the Shear Stiffness and Support Stiffness Reallocation in the Layer Under the Under-Sleeper Beam

The previous estimations in the sections b–c of the loading transmission for Case 2 assumed the constant stiffness ZW700 of the rail fastenings. The application of the elastic fastenings causes a redistribution of the deformations over the under-sleeper beam, and therefore, a reduction in the efficiency of the approach. On the other hand, the reallocation of the connecting layer stiffness from the fastening layer to the layer under the under-sleeper beam can cause activation of its bending and reduced efficiency of ballast loading. Therefore, the reallocation assumes the constant overall stiffness of the connecting layers. Additionally, the bending stiffness of the under-sleeper beam can be structurally increased, which again could improve the bending stiffness. Figure 12 demonstrates the influence of the stiffness reallocation and the under-beam stiffness together with shear interaction on the loading transmission on the sleeper support. The lines for the case without the shear interaction show that the stiffness reallocation has the effect in a narrow range (up to 100 kN/mm reallocated) of the stiffness support, but together with the own bending stiffness improvement it could cause significant reduction of the loading transmission. The effect can be approximately estimated from 30% for the present case to 23% for \(4\mathrm{EI}\) bending stiffness improvement and 100 kN/mm reallocated.

Fig. 12
figure 12

Relative loading on sleeper support for simple and shear connected rail and under-sleeper beams, with reallocated stiffness of support (1/c1 + 1/c2 = const)

Full size image

The shear interaction consideration (Fig. 12) shows a quite different influence of the stiffness reallocation on loading transmission. Stiffer rail fastenings activate the shear interaction between the rails and the under-sleeper beams in a wide range of the stiffness reallocation. This causes the reduction in the loading transmission to 18% for the practically possible reallocation range 500 kN/mm and \(1\mathrm{EI}\) bending stiffness. The additional increase in the bending stiffness of the under-sleeper beam causes a comparatively small reduction in the relative loading to 16%. Further reduction of the relative loading by the increase of the bending stiffness and the stiffness reallocation is theoretically possible but it would not provide the high effect since the minimally possible limit for the stiff connection is 14% of the rail loading. The theoretical limit follows from the Steiner's Theorem for the stiffly connected beams with the cross-section A at the distance h between them. The part \(A{h}^{2}\), which corresponds to the huge increment of the bending stiffness \(54\mathrm{EI}\), which is also visible from the converging lines to the limit value in Fig. 12. However, the increment in bending stiffness has a low effect after 5–8EI of the rail stiffness.

4 Comparison of Technical Solutions Using Equivalent Bending Stiffness

The previous sections have shown that the influence of track stabilization approaches on the loading transmission is complex. The same loading transmission can be achieved by improvement of bending stiffness with different combinations of support stiffness allocations between beams and the additional influence of shear interaction. On the other side, the variation of the relative loading is highly disproportional to the bending and linear stiffness parameters, as explained in (1, 2) and depicted in Fig. 3. Moreover, the variation of the elastic parameters can influence the track stiffness and the wheel trajectory. Therefore, the comparison of different technical solutions based on the loading transmission is complicated. A much more appropriate comparison criterion would be the equivalent bending stiffness of the whole track and the resulting track stiffness. The concept of equivalent track stiffness is based on reducing the FEM model to a simple analytical one as a beam on elastic foundation (Fig. 13).

Fig. 13
figure 13

Equivalent bending stiffness of rail track and track support

Full size image

The locally distributed bending stiffness corresponds to the equivalent bending stiffness \({\text{EI}}_{{{\text{eq}}}}\) of the beam in the simple model and linear stiffness to the stiffness of the elastic foundation \(c_{{{\text{eq}}}}\). The model reduction and the equivalent parameter estimation are based on the idea that if both models provide the same results, then they are equivalent. Thus, the fitting of the analytical model to the results of the complex numerical FEM model is produced. The most appropriate for fitting results are the support loading \(P_{{{\text{Sl}}, \max }}\) and rail reflection \(Z_{{{\text{R}}, \max }}\) under the external loading point. The support loading is considered for the part under all bending elements. The parameters \(P_{{{\text{Sl}}, \max }}\) and \(Z_{{{\text{R}}, \max }}\) appear in the following relations from (1–7):

$$P_{{{\text{Sl}}, \max }} = \frac{{P_{{\text{R}}} \cdot k \cdot l}}{2},$$
(16)
$$Z_{{{\text{R}}, \max }} = \frac{{P_{{\text{R}}} \cdot k}}{{2 \cdot U_{z} }}.$$
(17)

Equation (16) is used to derive the coefficient of the relative stiffness of rail support and rail bending \(k\):

$$k = \frac{{2P_{{{\text{Sl}}, \max }} }}{P \cdot l}.$$
(18)

The known parameter \(k\) is used to derive the equivalent track stiffness \(c_{{{\text{tr}},{\text{eq}}}}\) from (17) and the equivalent track bending stiffness \({\text{EI}}_{{{\text{tr}},{\text{eq}}}}\):

$$c_{{{\text{tr}},{\text{eq}}}} = \frac{{Z_{{{\text{R}}, \max }} }}{P} = \frac{{2 \cdot U_{z} }}{k},$$
(19)
$${\text{EI}}_{{{\text{tr}},{\text{eq}}}} = \frac{{U_{z} }}{{4 \cdot k^{4} }}.$$
(20)

Figure 14 presents an analysis of the influence of the bending element application (Case 1) on the equivalent track bending stiffness and track stiffness. The influence parameters are bending stiffness of track for \(N \times {\text{EI}}\) auxiliary rails, and the stiffness of the upper layer that assumes rail fastening and auxiliary rail one. The stiff track bed is considered, and the variation of the upper layer stiffness corresponds to the practically achievable scope by fastening systems. The range of the bending stiffness of the auxiliary rail is selected up to 8EI to make the comparison to the under-sleeper case possible. Practically, the most 2EI is structurally possible in the Case 1. The equivalent track bending stiffness dependence is presented in Fig. 14, left. The bottom line for the low fastening stiffness shows that even very high 8EI bending stiffness of the auxiliary rails causes a small effect on the overall track stiffness that corresponds to 1.3–1.7EI of the rail stiffness. Thus, the application of the auxiliary rails with elastic fastenings and also on the track with elastic rail fastenings is very ineffective.

Fig. 14
figure 14

Equivalent bending stiffness of track for N × EI auxiliary rails, variable stiffness of upper layer

Full size image

On the other side, an increase in the fastening stiffness up to 540 kN/mm for the 1EI auxiliary rail would provide an equivalent track stiffness of 1.8EI, which is close to the limit value of 2EI. This means that for stiff fastenings, the auxiliary rail and the loaded rail act approximately as one rail of double bending stiffness. The further increase of the auxiliary rail stiffness with stiff fastenings results in a high increase of the equivalent track bending stiffness. Nevertheless, the range of the auxiliary rail stiffness improvement is structurally limited and practically achievable track bending stiffness with the two auxiliary rails (4 to the track) and stiff fastenings ZW678 is not more than 2.5EI. A similar result could be reached with constructively possible three auxiliary rails and a lower fastening stiffness of 150 kN/mm, as follows from Fig. 14, left.

The resulting track stiffness (Fig. 14, right) increases together with the increase in the parameters of the auxiliary bending elements and the fastenings. This increases in the track stiffness could be compensated by the application of elastic elements under the sleepers or under the ballast layer that would additionally result in some increase in the track bending stiffness.

The analysis of the under-sleeper bending element application (Case 2) and its influence on the equivalent track bending stiffness and track stiffness is presented in Fig. 15. Two subcases are considered: without the shear interaction (Fig. 15, left-side) and with (Fig. 15, right-side) between the rail and the beam. Therefore, in the stiffness of the upper layer are included the elastic layers between the rail and the under-sleeper beam: rail fastenings and the possible elastic connection between the sleeper and the beam.

Fig. 15
figure 15

Equivalent bending stiffness (top) and track stiffness (bottom) of track for N × EI beam under sleepers, variable stiffness of upper layer, with structural stiffness (left) and without (right) due to shear interaction

Full size image

Figure 15, top-left, presents the equivalent bending stiffness depending on the under-sleeper beam stiffness and the spring stiffness of the layers over the beam. The range of the equivalent stiffness variation is similar to the Case 1 with the auxiliary beam solution (Fig. 14, right). However, the influence of the layer stiffness is higher, especially in combination with high bending stiffness of the under-sleeper beam. The equivalent bending stiffness is about \(2.7\mathrm{EI}\) for the actual \(8\mathrm{EI}\) of the under-sleeper beam instead 1.7 for the auxiliary rail case (Fig. 15). The reason is the lower support and therefore track stiffness for Case 2 (Fig. 14) than for Case 1 (Fig. 15, left bottom). The different track stiffness variation ranges for both cases can be explained by the fact that the bottom beam with its basis has higher overall stiffness than the upper layer. Similar to the Case 1, it could be observed that the same limit value \(2EI\) of the equivalent bending stiffness: the bending stiffness of the track can be arithmetically summed with that of the bending elements if the layer stiffness between is sufficiently high.

The shear interaction between the rail and the under-sleeper beam has the most significant influence on the track equivalent stiffness. It is especially noticeable if the layer stiffness is high: the equivalent stiffness can reach a considerably higher value than the summarized bending stiffness of the beams, e.g., the resulting equivalent bending stiffness is about \(7\mathrm{EI}\) in the case of the under-sleeper beam of the same bending stiffness as the rail EI but for the stiff connection in the upper layer. On the other side, similar to the Case 1, low stiffness of the connecting layer causes a low increase of the track stiffness even with a high increment in the bending stiffness. In contrast to the case without shear interaction (Fig. 15, left top), where the track bending stiffness is close to the limit state, the case with the interaction (Fig. 15, right top) is far from it, since the calculations were done for the horizontal stiffness as a quarter of the vertical one for the connecting layer. Thus, the equivalent track bending stiffness can be at least four times increased with the theoretical limit for one rail stiffness of the under-sleeper beam 54EI. Therefore, the increase in the structural stiffness due to shear interaction is the most effective approach for track geometry stabilization in zones of local instabilities.

5 Analytical Relation of Equivalent Bending Stiffness of Track for Application of Elastically Connected Auxiliary Beams

The analysis of track bending stiffness depending on the elastic and geometrical properties of the bending element as presented herein was produced using a numerical model. The analysis shows the complex influence of the main factors that cannot be clearly estimated. On the other hand, the simple analytical expressions (1–7) provide a clear understanding of the factor influence. Therefore, in the present section, we attempted to derive the simple analytical expressions. The differences between the Case 1 and the Case 2 are formulated by the same numerical model (Fig. 16) but with the different loading position. The shear interaction is available for Case 2.

Fig. 16
figure 16

Equivalent track bending stiffness depending on the stiffness of the auxiliary rail in the Case 1 (right) and under-sleeper beam (left) with connecting elastic layers in the Case 2

Full size image

The general expression of the equivalent stiffness for both cases is as follows:

$$N_{{{\text{tr}},{\text{eq}}}} = 1 + k_{1} \left( {c_{{{\text{v}}1}} ,c_{{{\text{v}}2}} } \right) \cdot N_{{{\text{EI}}}} + k_{2} \left( {c_{{{\text{h}}1}} } \right) \cdot h^{2} /I_{{\text{r}}}$$
(21)

where \(I_{{\text{r}}}\) is the inertia moment of rail, m4; \(N\) is the equivalent number of rails \(I_{{\text{r}}}\) corresponding to bending stiffness of auxiliary beam; \(h^{2}\) is the distance between beam and rail neutral axles, m; \(k_{1} \left( {c_{{{\text{v}}1}} ,c_{{{\text{v}}2}} } \right) \le 1\) is the coefficient of bending stiffness reduction if the connecting layers are elastic; \(k_{2} \left( {c_{{{\text{h}}1}} ,c_{{{\text{v}}1}} ,c_{{{\text{v}}2}} } \right) \le 1\) is the coefficient of structural stiffness reduction if the shear connecting layers are elastic.

If the elastic connections between the rail and the beam are high, then the coefficient \(k_{1}\) is close to 1 and the track bending stiffness is equal to the sum of the bending elements. For the case with auxiliary rail the coefficient \(k_{1}\) can be approximately calculated using the analytical semi-empirical expression:

$$k_{1} \left( {c_{{{\text{v}}1}} ,c_{{{\text{v}}2}} } \right) = \frac{{\left( {c_{{{\text{v}}1}} /c_{{{\text{v}}2}} } \right)^{0.75} \cdot N_{{{\text{EI}}}}^{{}} }}{{\sqrt[4]{{8N_{{{\text{EI}}}}^{2} }} + \left( {c_{{{\text{v}}1}} /c_{{{\text{v}}2}} } \right)^{0.75} }},$$
(22)

where \(c_{{{\text{v}}1}}\) is the vertical stiffness of the elastic layer between the auxiliary rail and sleeper; \(c_{{{\text{v}}2}}\) is the vertical stiffness of the elastic layer under the rail.

For the case with a bending beam under sleepers (Case 2), the influence of bending stiffness of the under-sleeper beam is higher than for Case 1 and the coefficient \(k_{1}\) can be calculated by the analytical semi-empirical expression:

$$k_{1} \left( {c_{{{\text{v}}1}} ,c_{{{\text{v}}2}} } \right) = \frac{{\left( {c_{{{\text{v}}1}} /c_{{{\text{v}}2}} } \right)^{0.75} \cdot N_{{{\text{EI}}}}^{{}} }}{{\sqrt[4]{{0.033N_{{{\text{EI}}}}^{2} }} + \left( {c_{{{\text{v}}1}} /c_{{{\text{v}}2}} } \right)^{0.75} }},$$
(23)

where \(c_{{{\text{v}}1}}\) is the vertical stiffness of elastic layer between rail and under-sleeper beam, kN/m; \(c_{{{\text{v}}2}}\) is the vertical stiffness of elastic layer under an under-sleeper beam, kN/m.

Both formulae (22, 23) show that the layers stiffness is not absolute but relative \(c_{{{\text{v}}1}} /c_{{{\text{v}}2}}\). The coefficient of the shear interaction \(k_{2}\) could be determined by the following relation:

$$k_{2} \left( {c_{{{\text{h}}1}} ,c_{{{\text{v}}1}} ,c_{{{\text{v}}2}} } \right) = \frac{{N_{{{\text{EI}}}}^{0.3} \cdot 0.22c_{{{\text{v}}1}} }}{{N_{{{\text{EI}}}}^{0.3} + c_{{{\text{v}}2}}^{0.68} }} \cdot \left( {6.8} \right)^{2} \cdot \left( {\frac{{4 \cdot c_{{{\text{h}}1}} }}{{c_{{{\text{v}}1}} }}} \right)^{0.75} ,$$
(24)

where \(c_{{{\text{h}}1}}\) is the horizontal stiffness of the elastic layer between the rail and under-sleeper beam, kN/m.

The maximal value of the shear part \(k_{2} \left( {c_{{{\text{h}}1}} } \right) \cdot h^{2}\) in the formula (1) should not be more than \(A \cdot h^{2}\), where \(A\) is the area of the auxiliary beam.

Figure 17 presents a comparison of the track equivalent bending stiffness for the application of auxiliary rails and under-sleeper beams with and without the shear interaction. Therefore, different parameters of the track reinforcement are considered: relation of the spring stiffness in the upper and the bottom layer, the bending stiffness of the auxiliary rail or the under-sleeper beam. The range of the variation is selected for convenience of comparison, and the actual one could be wider for some cases.

Fig. 17
figure 17

Benchmark of track bending stiffness for the application of auxiliary rails and under-sleeper beams with shear interaction

Full size image

6 Discussion

The literature review shows that there is no systematic analysis of the factor influence by application of various approaches of bending and elastic improvement of track and their local position in the structure of rail track. The low effect of auxiliary rails is known in the practice of maintenance for a long time. However, many theoretical studies claim good effects of auxiliary rails and fastenings improvements [3, 5, 6, 9]. Other studies [18, 20] indicate a low efficiency of the auxiliary rails. Most studies are based on computer simulations. Nevertheless, despite more and more complex simulation models or rather because of them, they are not able to give simple and reasonable answers.

The novelty of the present study is the estimation of the absolute technical efficiency within the possible range of improvement. The equivalent bending stiffness parameters were introduced as the criterion that allows to compare different technical solutions. Another novelty of the research is the consideration of the reinforcement using and under-sleeper beam that enables high improvement of the track structural bending stiffness due to activation of the longitudinal shear interaction. Therefore, the present study considers only the static effects in order to reach a clear understanding of the influence of the main factors.

A preliminary analysis of the factors of track bending stiffness and support spring stiffness using simple analytical relations shows that both factors have an equivalent effect on the loading transmission from rail to support (Fig. 4). However, the effect is highly nonlinear, e.g., more than three times increase in bending stiffness or the equivalent decrease in the support one amounts to less than a 2.5% reduction of the maximal relative loading (Fig. 3).

On the other side, the factors are not independent in the case of track reinforcement solutions at local zones such as transition zones where the overall track stiffness should be controlled. The increase in the track bending stiffness should be accompanied with the decrease in the support stiffness to preserve the track stiffness and the wheel trajectory. The combined effect could be estimated as the equivalent 40–50% increase in bending stiffness without changing the sleeper support (Fig. 5).

The analysis of the local position of the bending reinforcement was done for two cases: the auxiliary rail on elastic fastenings on the sleepers and the under-sleeper beam. The reduction of loading transmission to the rail support due to the application of the auxiliary rail is about 2–3% of the rail loading (Fig. 9). The reason for the low effect of the auxiliary rail is explained by elastic fastenings that do not allow the bending of the auxiliary rail to be activated. The application of the stiff connection of the auxiliary rail to the sleepers increased the effect to 4%, but still the auxiliary rail is not bent the same as the wheel loaded rail. The maximally possible effect 7% which corresponds to the double bending stiffness can be achieved with both stiff connection of the rails to the sleepers that is structurally implausible. The results correspond well to that of the study [20] that indicated less than a 5% reduction of the ballast compressive stress by the application of auxiliary rails. Low efficiency of auxiliary rails was also reported in the study [18] which used rail vertical displacement as an estimation criterion. However, the present study results do not correspond with those of the study [5] where the application of an auxiliary rail would cause vertical stress in the subgrade from 20 to 14 kPa, or a more than 25% improvement in efficiency. This divergence could be explained by unrealistically stiff fastenings in the simulation models. Another study [20] qualitatively states positive improvements of two auxiliary rails. However, the results are based on simulations that do not consider rail fastenings (or as absolutely stiff), and the experimental measurements of the authors indicate almost no difference between rail displacements with and without auxiliary rails.

The influence of the same bending stiffness under the sleepers for the same elastic connection has a significantly higher effect on the loading transmission than for the auxiliary rail—9–14% relative loading reduction. However, the higher influence is caused not by the under-sleeper beam bending but by activation of rail bending due to an increase in the support elasticity. The share part of the under-sleeper bending increases rather moderately, as can be observed from beam reactions (Fig. 10, top). For the auxiliary rail, the best effect from the under-sleeper beam can be obtained for the stiff beam-sleeper connection. However, also the under-sleeper beam is not bending the same as the loaded rail; in other words, its stiffness is not fully activated.

The activation of the bending stiffness of both the auxiliary rail and the under-sleeper beam can be done either by a stiffer connecting layer to the rail or a more elastic layer under both beams. Thus, the redistribution of the spring stiffness (with the constant overall one) could produce an additional 2–5% of the relative loading reduction (Fig. 12). An additional increase of the bending stiffness of the reinforcement beam more than 1EI can increase the further loading reduction, but the factor is structurally limited: not more than four auxiliary rails can be applied and a maximum of 10–20EI bending stiffness can be applied for under-sleeper beam.

The highest effect of the loading transmission can be achieved by activation of the horizontal shear interaction between the under-sleeper beam and the rail. The beam, different from the auxiliary rail, is located at a significant distance from the loaded rail. This can result in high Steiner’s share part of the structural stiffness that can be theoretically reached at about 13% of the loading transmission for the absolutely stiff beam-rail connection. Practically, the connection has limited stiffness that depends on the stiffness of rail fastenings and the elastic connection between the under-sleeper beam and the sleeper. The maximal effect of the loading transmission for the shear interaction and the feasible stiffness reallocation could be estimated to 18% of the rail loading.

The nonlinear decrease in the support loading is not the best indicator since with it one can still not be clear about the resulting settlements intensity. The nonlinearity does not allow us to estimate the increase in the improvement of some reinforcement solutions (Fig. 3). For the convenience of the comparison, the criterion would be better to present as the value of the track overall bending stiffness, i.e., the equivalent increase in the rail bending stiffness. The proposed method allows a simple estimation of the track bending stiffness from the FEM simulation of the multiple beam track model. Figures 14 and 15 clearly show the track bending stiffness improvement by the application of various bending stiffness of the auxiliary rail and the under-sleeper beam, layers spring stiffness and the shear interaction. The diagrams show that solutions with auxiliary rails on elastic fastenings are inefficient and are equivalent to the increase in the rail bending stiffness to 1.2–1.4EI, and the application of two auxiliary rails (for a half of the track) could increase the track bending stiffness up to 1.9EI.

Application of the under-sleeper beam without the horizontal shear interaction with elastic layer to the sleeper has a relatively low effect 1.4EI for the same bending stiffness under the sleeper as the rail bending stiffness. However, the further increase in the bending stiffness under the sleepers has a considerably higher effect than that for the auxiliary rail: 1.8–2.7EI of the bending parameters as in the V-Tras solution.

The additional reallocation of the layer stiffness from the fastenings to the under sleepers could produce up to an additional 0.4EI bending stiffness activation. Practically, it could be reached by application of more stiff fastenings together with under-sleeper pads or an elastic layer under the under-sleeper beam. However, the potentials of the reallocation are limited by the possible dynamic effects for the stiff fastenings.

The structural stiffness due to the horizontal shear interaction between the under-sleeper beam and the rail has the highest potential for improving track bending stiffness. Its effect could be estimated from 1.7EI for the lowest besting stiffness and the lowest spring stiffness of the upper layer to 7–16EI for high spring and bending stiffness. Therefore, the layer stiffness is a dominating factor. The track bending stiffness for the V-Tras solution could be estimated as not less than 3.2EI.

The study has additionally presented the analytical relation of the equivalent bending stiffness of track for the application of elastically connected auxiliary beams. The equivalent bending stiffness is presented as the sum of the rail stiffness, the reduced bending stiffness of the bending reinforcement and the shear component. The relations show that the equivalence coefficients depend on the relative stiffness of the upper and bottom layers and not on the absolute values. The influence of the beam bending stiffness is close to linear. The benchmark of the various reinforcement methods (Fig. 17) clearly shows the loading distribution effect of the auxiliary rail in comparison with the other approaches. Nevertheless, the life-cycle costing (LCC) effect of the approaches could be different. The not considered effect of the train-track dynamic interaction could also bring additional influence on loading transmission in the superstructure.

7 Conclusions

The following main conclusions could be formulated from the study:

  • Conventional solutions based on auxiliary rails on elastic fastening reinforcement are ineffective. The bending stiffness of the auxiliary rail is poorly activated due to the high elasticity of the fastening clips and main rail fastenings. The share of an auxiliary rail is maximally 20% in the track bending stiffness.

  • The support loading effect of the track reinforcement approaches by application of auxiliary rails or under-sleeper beams heavily depends on the connection layers between the bending elements.

  • The increase in the bending stiffness by the increase in the number of auxiliary rails with the same elastic fastenings does not result in significant loading distribution properties. The equivalent bending stiffness of the track increases not more than 30–40% of the rail bending stiffness for two additional auxiliary rails (for a half of track) and elastic fastenings.

  • The under-sleeper beam-based reinforcement solutions show noticeably higher efficiency. The equivalent bending stiffness of the track can be increased more than 1.9 times of the rail bending stiffness for the possible increase of the under-sleeper stiffness more than three times the rails’ stiffness.

  • The activation of the bending stiffness of the reinforcement beams depends on the relative ratio of the rail fastenings stiffness and track support stiffness under sleepers (or under the under-sleeper beam).

  • Reallocation of the linear spring stiffness from the fastenings under the sleeper is an efficient measure for the growth of track bending stiffness. Application of stiff rail fastenings together with under-sleeper pads could significantly activate the bending of the reinforcement.

  • The highest effect can be achieved by the activation of the horizontal shear interaction between the under-sleeper beam and the rail. The interaction depends on the distance between the beam and the rail and the horizontal stiffness. The additional track bending stiffness of the under-sleeper-based solutions is about 3.5 times that of the rails and could be potentially increased 6–10 times.