Topological Effects with Inverse Quadratic Yukawa Plus Inverse Square Potential on Eigenvalue Solutions

Abstract

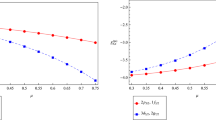
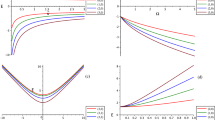
We study the nonrelativistic Schrödinger wave equation under the influence of a quantum flux field with an interaction potential in the background of a pointlike global monopole (PGM). In fact, we consider an inverse quadratic Yukawa plus inverse square potential and derive the radial equation employing the Greene–Aldrich approximation scheme in the centrifugal term. We determine the approximate eigenvalue solution using the parametric Nikiforov–Uvarov method and analyze the result. Afterwards, we derive the radial wave equation using the same potential employing a power series expansion method in the exponential potential and solve it analytically. We show that the energy eigenvalues are shifted by the topological defects of a pointlike global monopole as compared to the flat space result. In addition, we see that the energy eigenvalues depend on the quantum flux field that shows an analogue to the Aharonov–Bohm effect.

Appendix

1.1 THE NIKIFOROV–UVAROV (NU) PARAMETRIC METHOD

The Nikiforov–Uvarov method is a helpful technique to calculate exact and approximate energy eigenvalues and wave functions of Schrödinger-like equations and other second-order differential equations of physical interest. According to this method, the wave functions of a second-order differential equation [42]

$$\frac{d^{2}\psi(s)}{ds^{2}}+\frac{(\alpha_{1}-\alpha_{2}\,s)}{s\,(1-\alpha_{3}\,s)}\frac{d\psi(s)}{ds}$$

$${}+\frac{(-\xi_{1}\,s^{2}+\xi_{2}\,s-\xi_{3})}{s^{2}\,(1-\alpha_{3}\,s)^{2}}\psi(s)=0$$

(A.1)

are given by

$$\psi(s)=s^{\alpha_{12}}(1-\alpha_{3}s)^{-\alpha_{12}-\frac{\alpha_{13}}{\alpha_{3}}}$$

$${}\times P^{(\alpha_{10}-1,\frac{\alpha_{11}}{\alpha_{3}}-\alpha_{10}-1)}_{n}(1-2\alpha_{3}s),$$

(A.2)

and that the energy eigenvalue equation holds,

$$\alpha_{2}\,n-(2\,n+1)\,\alpha_{5}+(2\,n+1)\,(\sqrt{\alpha_{9}}+\alpha_{3}\,\sqrt{\alpha_{8}})$$

$${}+n\,(n-1)\,\alpha_{3}+\alpha_{7}+2\,\alpha_{3}\,\alpha_{8}$$

$${}+2\,\sqrt{\alpha_{8}\,\alpha_{9}}=0.$$

(A.3)

The parameters $\alpha_{4},\ldots,\alpha_{13}$ are obatined from the six parameters $\alpha_{1},\ldots,\alpha_{3}$ and $\xi_{1},\ldots,\xi_{3}$ as follows:

$$\alpha_{4}=\frac{1}{2}\,(1-\alpha_{1}),\quad\alpha_{5}=\frac{1}{2}\,(\alpha_{2}-2\,\alpha_{3}),$$

$$\alpha_{6}=\alpha^{2}_{5}+\xi_{1},\quad\alpha_{7}=2\,\alpha_{4}\,\alpha_{5}-\xi_{2},$$

$$\alpha_{8}=\alpha^{2}_{4}+\xi_{3},\quad\alpha_{9}=\alpha_{6}+\alpha_{3}\,\alpha_{7}+\alpha^{2}_{3}\,\alpha_{8},$$

$$\alpha_{10}=\alpha_{1}+2\,\alpha_{4}+2\,\sqrt{\alpha_{8}},$$

$$\alpha_{11}=\alpha_{2}-2\,\alpha_{5}+2\,(\sqrt{\alpha_{9}}+\alpha_{3}\,\sqrt{\alpha_{8}}),$$

$$\alpha_{12}=\alpha_{4}+\sqrt{\alpha_{8}},$$

$$\alpha_{13}=\alpha_{5}-(\sqrt{\alpha_{9}}+\alpha_{3}\,\sqrt{\alpha_{8}}).$$

(A.4)

In a special case where $\alpha_{3}=0$, we find

$$\lim_{\alpha_{3}\to 0}P^{(\alpha_{10}-1,\frac{\alpha_{11}}{\alpha_{3}}-\alpha_{10}-1)}_{n}\,(1-2\alpha_{3}\,s)$$

$${}=L^{\alpha_{10}-1}_{n}(\alpha_{11}\,s),$$

(A.5)

and

$$\lim_{\alpha_{3}\to 0}(1-\alpha_{3}\,s)^{-\alpha_{12}-\frac{\alpha_{13}}{\alpha_{3}}}=e^{\alpha_{13}\,s}.$$

(A.6)

Therefore the wave function from (A.2) becomes

$$\psi(s)=s^{\alpha_{12}}\,e^{\alpha_{13}\,s}\,L^{\alpha_{10}-1}_{n}(\alpha_{11}\,s),$$

(A.7)

where $L^{(\beta)}_{n}(x)$ denotes the generalized Laguerre polynomial.

The energy eigenvalues equation reduces to

$$n\,\alpha_{2}-(2\,n+1)\,\alpha_{5}+(2\,n+1)\,\sqrt{\alpha_{9}}+\alpha_{7}$$

$${}+2\,\sqrt{\alpha_{8}\,\alpha_{9}}=0.$$

(A.8)