Hostname: page-component-76fb5796d-25wd4 Total loading time: 0 Render date: 2024-04-28T08:12:18.254Z Has data issue: false hasContentIssue false
  • English
  • Français

Some measure rigidity and equidistribution results for β-maps

Part of: Measure-theoretic ergodic theory Elementary number theory

Published online by Cambridge University Press:  23 October 2023

NEVO FISHBEIN
NEVO FISHBEIN*
Affiliation:
Independent Scholar, Israel
*
e-mail: nevofi@gmail.com
Get access Rights & Permissions [Opens in a new window]

Abstract

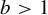We prove $\times a \times b$ measure rigidity for multiplicatively independent pairs when $a\in \mathbb {N}$ and $b>1$ is a ‘specified’ real number (the b-expansion of 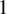$1$ has a tail or bounded runs of 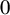$0$s) under a positive entropy condition. This is done by proving a mean decay of the Fourier series of the point masses average along 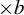$\times b$ orbits. We also prove a quantitative version of this decay under stronger conditions on the $\times a$ invariant measure. The quantitative version together with the 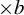$\times b$ invariance of the limit measure is a step toward a general Host-type pointwise equidistribution theorem in which the equidistribution is for Parry measure instead of Lebesgue. We show that finite memory length measures on the a-shift meet the mentioned conditions for mean convergence. Our main proof relies on techniques of Hochman.

Keywords

β-mapsParry measuremeasure rigidity

MSC classification

Primary: 28D05: Measure-preserving transformations
Secondary: 11A63: Radix representation; digital problems
Type
Original Article
Information
Ergodic Theory and Dynamical Systems , First View , pp. 1 - 18
Check for updates
Copyright
© The Author(s), 2023. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Billingsley, P.. Probability and Measure, 2nd edn. Wiley, New York, 1986.Google Scholar
Cornfeld, I. P., Fomin, S. V. and Sinai, Y. G.. Ergodic Theory. Springer, Berlin, 1982.CrossRefGoogle Scholar
Furstenberg, H.. Disjointness in ergodic theory, minimal sets, and a problem in diophantine approximation. Math. Syst. Theory 1 (1967), 1–49.CrossRefGoogle Scholar
Hochman, M.. A short proof of Host’s equidistribution theorem. Israel J. Math. 251 (2022), 527–539.CrossRefGoogle Scholar
Hochman, M. and Shmerkin, P.. Equidistribution from fractal measures. Invent. Math. 202 (2015), 427–479.CrossRefGoogle Scholar
Host, B.. Nombres normaux, entropie, translations. Israel J. Math. 91 (1995), 419–428.CrossRefGoogle Scholar
Johnson, A. S. A.. Measures on the circle invariant under multiplication by a nonlacunary subsemigroup of the integers. Israel J. Math. 77 (1992), 211–240.CrossRefGoogle Scholar
Lyons, R.. Seventy years of Rajchman measures. J. Fourier Anal. Appl. (1995), 363–377 (Kahane Special Issue).Google Scholar
Parry, W.. On the $\beta$ -expansions of real numbers. Acta Math. Hungar. 11 (1960), 401–416.CrossRefGoogle Scholar
Rudolph, D. J.. $\times$ 2 and $\times$ 3 invariant measures and entropy. Ergod. Th. & Dynam. Sys. 10(2) (1990), 395–406.CrossRefGoogle Scholar
Schmeling, J.. Symbolic dynamics for $\beta$ -shifts and self-normal numbers. Ergod. Th. & Dynam. Sys. 17(3) (1997), 675–694.CrossRefGoogle Scholar
Shields, P. C.. The Ergodic Theory of Discrete Sample Paths (Graduate Studies in Mathematics, 13). American Mathematical Society, Providence, RI, 1996.CrossRefGoogle Scholar
Varjú, P. and Yu, H.. Fourier decay of self-similar measures and self-similar sets of uniqueness. Anal. PDE 15(3) (2020), 843–858.CrossRefGoogle Scholar
Walters, P.. An Introduction to Ergodic Theory (Graduate Texts in Mathematics, 79). Springer, New York, 2000.Google Scholar