Intrapersonal price discrimination and welfare in a dominant firm model

Abstract

In a homogeneous good industry composed of a dominant firm and a fringe of followers that can choose non-linear pricing contracts to sell the good, we demonstrate that only the dominant firm uses them. Compared with the standard dominant firm model in which only linear pricing contracts are feasible, the dominant firm supplies an inefficiently low number of customers as a way to extract more surplus, since the alternative for customers is a fringe cluttered by excess demand. Thus, allowing market-power firms to deploy non-linear pricing contracts leads to market segmentation, and customers end up worse off than under linear pricing contracts. Fringe firms, in contrast, are better off since they end up charging a higher price for the good. Finally, aggregate welfare under non-linear pricing increases (decreases) as compared to linear pricing if the dominant firm’s share of production capacity is (is not) large enough.

Appendix

Proof of Lemma 1

(i) When $0\le CS\left(F,q\right)\le \underline{CS}$, it follows that $\frac{{\partial \pi }_{f}\left({q}_{f},{a}_{f}|CS(F,q)\right)}{\partial {a}_{f}}>0$ when evaluated at ${a}_{f}=\frac{1}{1-k}$. Thus, fringe firms compete among them to offer customers the competitive quantity ${q}_{min}$ and a strictly better deal than that offered by the DF and leading to consumer surplus CS. (If there were only one firm in the fringe with $1-k$ productive plants, then it would sell to all customers, and would offer the quantity ${q}_{min}$ in Eq. (10) in exchange for a payment that leads to a consumer surplus slightly above $CS\left(F,q\right)$.)

(ii) When $CS\left(F,q\right)>\underline{CS}$, it follows that $\frac{{\partial \pi }_{f}\left({q}_{f},{a}_{f}|CS(F,q)\right)}{\partial {a}_{f}}=0$ in Eq. (8) for a quantity ${q}_{f}$ above ${q}_{min}$ and ${a}_{f}<\frac{1}{1-k}$. Therefore, $CS\left(F,q\right)>\underline{CS}$ guarantees that the fringe does not hoard all customers and DF has strictly positive sales. Moreover, the fact that $\frac{{\partial \pi }_{f}\left({q}_{f},{a}_{f}|CS(F,q)\right)}{\partial {a}_{f}}=0$ in Eq. (8) shows that fringe firms optimally choose linear prices.

Proof of Lemma 2

(i) From Eq. (11) we know that, in equilibrium, the number of customers the DF chooses to serve, ${a}^{l}$, leads to ${U}^{\prime}\left({q}_{f}\right)>{c}^{\prime}\left(\frac{{a}^{l}}{k}{q}_{f}\right)$. Both the condition given in Eq. (10) and the strict convexity of the firms’ cost functions imply that $\frac{{a}^{l}}{k}{q}_{f}<\frac{1-{a}^{l}}{1-k}{q}_{f}$, so this part of the lemma is straightforwardly proved.

(ii) In the first-best scenario, fringe firms supply the good to 1 − k customers, whereas part (i) of this lemma shows that they supply, in equilibrium, more than 1 − k customers. Hence, from Eq. (11) it follows that ${q}_{f}<{q}^{fb}$, since the larger the customer base, the lower the quantity offered to each customer.

(iii) Immediate from (ii).

Proof of Lemma 3.

(i) From the FOC given in Eq. (14), the DF chooses the pair $(a,q)$ satisfying

$$U\left(q\right)-{U}^{\prime}\left(q\right) q>U\left({q}_{f}\right)-{U}^{\prime}\left({q}_{f}\right) {q}_{f}$$

(23)

and from the inequality stated in Eq. (23), the fact that $\frac{\partial \left(U\left(x\right)-{U}^{\prime}\left(x\right) x\right)}{\partial x}=-{U}^{{\prime}{\prime}}\left(x\right) x>0$ holds, for $x\in \left\{q,{q}_{f}\right\}$, leads to $q>{q}_{f}$.

(ii) and (iii) From ${U}^{{\prime}{\prime}}<0$, the inequality $q>{q}_{f}$ implies that ${U}^{\prime}\left(q\right)<{U}^{\prime}\left({q}_{f}\right)$. From Eqs. (6) and (12), this requires that ${c}^{\prime}\left(\frac{a q}{k}\right)<{c}^{\prime}\left(\frac{(1-a){q}_{f}}{1-k}\right)$ holds, which implies that $\frac{a q}{k}<\frac{(1-a){q}_{f}}{1-k}$ by the fact that ${c}^{{\prime}{\prime}}\left(\cdot \right)>0$. In turn, the inequality $\frac{a q}{k}<\frac{(1-a){q}_{f}}{1-k}$ and the fact that $q>q(a)$ together imply that $a<{a}^{fb}=k$. Since fringe firms supply more customers and the DF supplies fewer customers than in the first-best scenario and, since all customers receive the efficient quantity of the good (given the number of customers supplied), it immediately follows that ${q}_{f}^{nl}<{q}^{fb}<{q}^{nl}$.

(iv) The inequality ${q}_{f}^{nl}<{q}^{fb}$ implies that customers supplied by fringe firms are worse off than if they were supplied in a competitive environment. On the other hand, customers supplied by the DF consume a larger quantity of the good than customers supplied by fringe firms, but are charged a positive fixed fee that yields the same consumer surplus as if they were supplied by fringe firms.

Proof of Proposition 1.

To prove this proposition, we first need to state two preliminary lemmas denoted by Lemmas A1 and A2.

Lemma A1

$${q}_{f}\left({a}^{l}\right)<q\left({a}^{nl}\right)$$

Proof

That customers supplied by the DF receive a smaller quantity of good if linear pricing rather than non-linear is allowed is proved as follows. From Lemmas 2 and 3 we know that $\mathrm{max }\left\{{a}^{l},{a}^{nl}\right\}<k$. Assume that ${a}^{l}>{a}^{nl}$. In this case, ${q}_{f}\left({a}^{l}\right)<q\left({a}^{nl}\right)<q\left({a}^{l}\right)$, where the first inequality, ${q}_{f}\left({a}^{l}\right)<q\left({a}^{nl}\right),$ holds from Eqs. (7) and (13) and the fact that ${a}^{l}<k$, whereas the second inequality, $q\left({a}^{nl}\right)<q\left({a}^{l}\right)$, holds from assuming ${a}^{nl}<{a}^{l}$.

Assume now that ${a}^{l}<{a}^{nl}$. In this case, ${q}_{f}\left({a}^{l}\right)<{q}_{f}\left({a}^{nl}\right)<q\left({a}^{nl}\right),$ where the first inequality, ${q}_{f}\left({a}^{l}\right)<{q}_{f}\left({a}^{nl}\right),$ follows from the factsa that fringe firms supply fewer customers when ${a}^{l}<{a}^{c}$, and the second inequality, ${q}_{f}\left({a}^{nl}\right)<q\left({a}^{nl}\right),$ holds from Eqs. (10) and (16) and the fact that ${a}^{nl}<k$

Now, with Lemma A1 in mind, let $H\left(a,q\right)$ be the function defined as:

$$H\left(a,q\right)=\left[U\left(q\right)-{c}^{\prime}\left(\frac{aq}{k}\right)q-CS\left({q}_{f}(a)\right)\right]+\frac{{U}^{{\prime}{\prime}}\left(q\right)q+{U}^{\prime}\left(q\right)-{c}^{\prime}\left(\frac{aq}{k}\right)}{{U}^{{\prime}{\prime}}\left(q\right)q}{U}^{{\prime}{\prime}}\left({q}_{f}\left(a\right)\right){q}_{f}\left(a\right)a\frac{\partial {q}_{f}\left(a\right)}{\partial a}=0$$

(24)

Note that $H\left({a}^{l},{q}_{f}({a}^{l})\right)$ is equivalent to the FOC of the DF’s maximization problem when only linear pricing contracts are feasible; therefore, it follows that $H\left({a}^{l},{q}_{f}({a}^{l})\right)=0$. In turn, since $H\left({a}^{nl},q({a}^{nl})\right)$ is equivalent to the FOC of the DF’s maximization problem when non-linear pricing contracts are allowed, it also follows that $H\left({a}^{nl},q({a}^{nl})\right)=0$.

Since ${q}_{f}\left({a}^{nl}\right)<q\left({a}^{nl}\right)$ from Lemma A1, we can analyze how the number of customers, $a$, evolves when $q$ increases in the interval $\left[{q}_{f}\left({a}^{l}\right), q\left({a}^{nl}\right)\right]$ to satisfy $H\left(a(q),q\right)=0$ in Eq. (24). We can therefore state the following lemma.

Lemma A2.

$\frac{\partial a}{\partial q}<0$ along $H\left(a(q),q\right)=0$.

Proof.

The part in brackets of function $H\left(a,q\right)$ defined in Eq. (24) is always positive in the interval $q\in \left[{q}_{f}\left({a}^{l}\right),q\left({a}^{nl}\right)\right]$, since

$$U\left(q\right)-{c}^{\prime}\left(\frac{aq}{k}\right)q>U\left(q\right)-{U}^{\prime}\left(q\right)q>CS\left({q}_{f}\left(a\right)\right)$$

(25)

where the first inequality, $U\left(q\right)-{c}^{\prime}\left(\frac{aq}{k}\right)q>U\left(q\right)-{U}^{\prime}\left(q\right)q,$ holds from Eq. (12), and where $q\le q\left({a}^{nl}\right) \text{ and } {c}^{{\prime}{\prime}}\left(\cdot \right)>0,$ whereas the second inequality, $U\left(q\right)-{U}^{\prime}\left(q\right)q>CS\left({q}_{f}\left(a\right)\right),$ derives from the fact that ${{CS}^{{\prime}{\prime}}\left(q\right)=-U}^{{\prime}{\prime}}\left(q\right)q>0$ and ${q>q}_{f}\left({a}^{l}\right).$

From the implicit function theorem, it holds that $\frac{\partial a}{\partial q}=-\frac{\frac{\partial H\left(a,q\right)}{\partial q}}{\frac{\partial H\left(a,q\right)}{\partial a}},$ whereby it follows that $\mathrm{sign} \frac{\partial a}{\partial q}=\mathrm{sign}\frac{\partial H\left(a,q\right)}{\partial q}$. Thus, taking into account that

$$\frac{\partial H\left(a,q\right)}{\partial q}={U}^{\prime}\left(q\right)-{c}^{\prime}\left(\frac{aq}{k}\right)-{c}^{{\prime}{\prime}}\left(\frac{aq}{k}\right)\frac{aq}{k}+\frac{\partial \left(\frac{{U}^{{\prime}{\prime}}\left(q\right)q+{U}^{\prime}\left(q\right)-{c}^{\prime}\left(\frac{aq}{k}\right)}{{U}^{{\prime}{\prime}}\left(q\right)q}\right)}{\partial q}{U}^{{\prime}{\prime}}\left({q}^{l}\right){q}^{l}a\frac{\partial {q}^{l}\left(a\right)}{\partial a}$$

(26)

we can conclude from Eq. (24) that

$${U}^{{\prime}{\prime}}\left({q}^{l}\right){q}^{l}a\frac{\partial {q}^{l}\left(a\right)}{\partial a}=-\left(U\left(q\right)-{c}^{\prime}\left(\frac{aq}{k}\right)q-CS\left({q}^{l}\right)\right)\frac{{U}^{{\prime}{\prime}}\left(q\right)q}{{U}^{{\prime}{\prime}}\left(q\right)q+{U}^{\prime}\left(q\right)-{c}^{\prime}\left(\frac{aq}{k}\right)}<0$$

(27)

On the other hand

$$\begin{aligned} \frac{{\partial \left( {\frac{{U^{\prime\prime}\left( q \right)q + U^{\prime}\left( q \right) - c^{\prime}\left( \frac{aq}{k} \right)}}{{U^{\prime\prime}\left( q \right)q}}} \right)}}{\partial q} = & \frac{{\left( {U^{\prime\prime}\left( q \right) - c^{\prime\prime}\left( \frac{aq}{k} \right)\frac{a}{k}} \right)U^{\prime\prime}\left( q \right)q - \left( {U^{\prime}\left( q \right) - c^{\prime}\left( \frac{aq}{k} \right)} \right)\left( {U^{\prime\prime\prime}\left( q \right)q + U^{\prime\prime}\left( q \right)} \right)}}{{\left[ {U^{\prime\prime}\left( q \right)q} \right]^{2} }} \\ & \quad = \frac{{\left( {U^{\prime\prime}\left( q \right) - c^{\prime\prime}\left( \frac{aq}{k} \right)\frac{a}{k}} \right)\left( {U^{\prime\prime}\left( q \right)q + U^{\prime}\left( q \right) - c^{\prime}\left( \frac{aq}{k} \right)} \right) - \left( {U^{\prime}\left( q \right) - c^{\prime}\left( \frac{aq}{k} \right)} \right)\left( {U^{\prime\prime\prime}\left( q \right)q + 2U^{\prime\prime}\left( q \right) - c^{\prime\prime}\left( \frac{aq}{k} \right)\frac{a}{k}} \right)}}{{\left[ {U^{\prime\prime}\left( q \right)q} \right]^{2} }} \\ & \quad > \frac{{\left( {U^{\prime\prime}\left( q \right) - c^{\prime\prime}\left( \frac{aq}{k} \right)\frac{a}{k}} \right)\left( {U^{\prime\prime}\left( q \right)q + U^{\prime}\left( q \right) - c^{\prime}\left( \frac{aq}{k} \right)} \right)}}{{\left[ {U^{\prime\prime}\left( q \right)q} \right]^{2} }} \\ & \quad > \frac{{U^{\prime\prime}\left( q \right)q + U^{\prime}\left( q \right) - c^{\prime}\left( \frac{aq}{k} \right)}}{{\left[ {U^{\prime\prime}\left( q \right)q} \right]^{2} }} \\ \end{aligned}$$

(28)

where the first inequality,

$$\begin{aligned} & \frac{{\left( {U^{\prime\prime}\left( q \right) - c^{\prime\prime}\left( \frac{aq}{k} \right)\frac{a}{k}} \right)\left( {U^{\prime\prime}\left( q \right)q + U^{\prime}\left( q \right) - c^{\prime}\left( \frac{aq}{k} \right)} \right) - \left( {U^{\prime}\left( q \right) - c^{\prime}\left( \frac{aq}{k} \right)} \right)\left( {U^{\prime\prime\prime}\left( q \right)q + 2U^{\prime\prime}\left( q \right) - c^{\prime\prime}\left( \frac{aq}{k} \right)\frac{a}{k}} \right)}}{{\left[ {U^{\prime\prime}\left( q \right)q} \right]^{2} }} > \\ & \frac{{\left( {U^{\prime\prime}\left( q \right) - c^{\prime\prime}\left( \frac{aq}{k} \right)\frac{a}{k}} \right)\left( {U^{\prime\prime}\left( q \right)q + U^{\prime}\left( q \right) - c^{\prime}\left( \frac{aq}{k} \right)} \right)}}{{\left[ {U^{\prime\prime}\left( q \right)q} \right]^{2} }} \\ \end{aligned}$$

holds from the assumption

$${U}^{{\prime}{\prime}{\prime}}\left(q\right) q+2{ U}^{{\prime}{\prime}}\left(q\right)-{c}^{{\prime}{\prime}}\left(\frac{a q}{k}\right)\frac{a}{k}<0$$

(29)

and the second inequality, $\frac{\left({U}^{{\prime}{\prime}}\left(q\right)-{c}^{{\prime}{\prime}}\left(\frac{aq}{k}\right)\frac{a}{k}\right)\left({U}^{{\prime}{\prime}}\left(q\right)q+{U}^{\prime}\left(q\right)-{c}^{\prime}\left(\frac{aq}{k}\right)\right)}{{\left[{U}^{{\prime}{\prime}}\left(q\right)q\right]}^{2}}>\frac{{U}^{{\prime}{\prime}}\left(q\right)q+{U}^{\prime}\left(q\right)-{c}^{\prime}\left(\frac{aq}{k}\right)}{{\left[{U}^{{\prime}{\prime}}\left(q\right)q\right]}^{2}}$, holds from the fact that

$$\frac{{U}^{{\prime}{\prime}}\left(q\right)-{c}^{{\prime}{\prime}}\left(\frac{aq}{k}\right)\frac{a}{k}}{{U}^{{\prime}{\prime}}(q)}>1$$

(30)

Finally, the last expression in Eq. (28), $\frac{{U}^{{\prime}{\prime}}\left(q\right)q+{U}^{\prime}\left(q\right)-{c}^{\prime}\left(\frac{aq}{k}\right)}{{\left[{U}^{{\prime}{\prime}}\left(q\right)q\right]}^{2}}$, is positive since the pair $(a,q)$ satisfies ${U}^{{\prime}{\prime}}\left(q\right) q+{U}^{\prime}\left(q\right)-{c}^{\prime}\left(\frac{aq}{k}\right)<0$ (see above). Thus, Eqs. (27) and (28) together imply that

$$\begin{aligned} \frac{{\partial H\left( {a,q} \right)}}{\partial q} = & \, U^{\prime}\left( q \right) - c^{\prime}\left( \frac{aq}{k} \right) - c^{\prime\prime}\left( {\frac{a q}{k}} \right)\frac{a q}{k} + \frac{{\partial \left( {\frac{{U^{\prime\prime}\left( q \right) q + U^{\prime}\left( q \right) - c^{\prime}\left( {\frac{a q}{k}} \right)}}{{U^{\prime\prime}\left( q \right) q}}} \right)}}{\partial q}U^{\prime\prime}\left( {q^{l} } \right) q^{l} a\frac{{\partial q^{l} \left( a \right)}}{\partial a} \\ & \quad < \, U^{\prime} \left( q \right) - c^{\prime}\left( {\frac{a q}{k}} \right) - c^{\prime\prime}\left( {\frac{a q}{k}} \right)\frac{a q}{k} - \frac{{U\left( q \right) - c^{\prime}\left( {\frac{a q}{k}} \right) - CS\left( {q^{l} } \right)}}{q} \\ & \quad = - \frac{{CS\left( q \right) - CS\left( {q^{l} } \right)}}{q} - c^{\prime\prime}\left( {\frac{a q}{k}} \right)\frac{a q}{k} \\ \end{aligned}$$

(31)

and the last expression in Eq. (31), $-\frac{CS\left(q\right)-CS\left({q}^{l}\right)}{q}-{c}^{{\prime}{\prime}}\left(\frac{a q}{k}\right)\frac{a q}{k}$, is negative from the fact that consumer surplus is increasing in $q$, $q>{q}^{l}$ and ${c}^{{\prime}{\prime}}\left(\cdot \right)>0$. In sum, both $\mathrm{sign}\frac{\partial H\left(a,q\right)}{\partial q}<0$ and ${q}_{f}\left({a}^{l}\right)<q\left({a}^{nl}\right)$ imply that ${a}^{nl}<{a}^{l}$. Hence, Proposition 1 straightforwardly holds from Lemmas A1 and A2.

Proof of Proposition 2.

If the DF uses linear pricing contracts and decides to supply $a$ customers, customers that purchase from fringe firms pay the unit price $p\left(a,k\right)=\frac{1-a}{2-a-k}$ and consume the quantity ${q}_{f}\left(a,k\right)=\frac{1-k}{2-a-k}$. Therefore, the DF obtains profits ${\pi }^{l}\left(a,k\right)=\frac{a\left(2k+a\left(1+k\right)\right)(1-k)}{2k{\left(2-k-a\right)}^{2}}$, and decides to supply ${a}^{l}\left(k\right)=\frac{k\left(2-k\right)}{2-{k}^{2}}$ customers. These customers consume the quantity ${q}^{l}\left(k\right)=\frac{2-{k}^{2}}{2-{k}^{2}}$ and obtain the consumer surplus ${CS}^{l}\left(k\right)=\frac{1}{2}{\left(\frac{2-{k}^{2}}{2-{k}^{2}}\right)}^{2}$. Thus, aggregate welfare in equilibrium amounts to ${W}^{l}\left(k\right)=\frac{4+2k-{k}^{2}-{k}^{3}}{2\left(2-k\right)(2+{k)}^{2}}$. Contrariwise, if the DF uses non-linear pricing contracts with its customers, then it offers the marginal price ${p}^{nl}\left(a,k\right)=\frac{a}{k+a}$ in the 2PT contract, which leads to the consumption level ${q}^{nl}\left(a,k\right)=\frac{k}{k+a}$. As a result, the DF obtains profits ${\pi }^{nl}(a,k)=\frac{a\left(3-2k-\left(1+2k-{k}^{2}\right)a+k{a}^{2}\right)}{2\left(k+a\right){(2-k-a)}^{2}}$ and chooses the market share ${a}^{nl}(k)$ that satisfies the FOC

$$0=\frac{\partial {\pi }^{nl}(a,k)}{\partial a}=\frac{{k}^{2}\left(6-7k+2{k}^{2}\right)-k\left(4+3k-6{k}^{2}+2{k}^{3}\right)a-\left(2-3k-6{k}^{2}+4{k}^{3}\right){a}^{2}-\left(1-2k+2{k}^{2}\right){a}^{3}}{2{{\left(k+a\right)}^{2}\left(2-k-a\right)}^{3}}$$

(32)

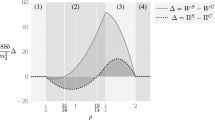
Figure 1 in the main text shows that ${a}^{nl}\left(k\right)<{a}^{l}\left(k\right)<k$. As a consequence, customers supplied by fringe firms consume lower quantities, ${q(a}^{nl},k)<{q(a}^{l},k)$, and pay higher prices, ${p(a}^{nl},k)>{p(a}^{l},k)$, when the DF sets non-linear pricing contracts than when it sets linear pricing contracts. This means that ${CS}^{nl}\left(k\right)<{CS}^{l}\left(k\right)$, i.e., the consumer surplus is lower when non-linear pricing is allowed, whereas the fringe firms’ profits are higher. Moreover, under both pricing strategies, as the DF’s size $k$ increases the consumer surplus decreases to converge to the consumer surplus achieved under monopoly, ${CS}^{nl}\left(1\right)=0<{CS}^{l}\left(1\right)$. Finally, the aggregate welfare obtained under non-linear pricing contracts, amounting to ${W(a}^{nl},k)=\frac{\left(1-k\right)k+\left(1-a\right)a}{2\left(2-k-a\right)(k+a)}$, is higher than that achieved under linear pricing contracts whenever the DF’s size is $k\in \left(0.7190, 1\right]$ and otherwise is lower. This completes the proof of Proposition 2.