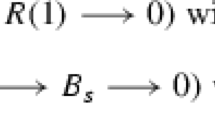Skip to main content
SpringerLink
Log in

Generalization of the Dehornoy–Lafont Order Complex to Categories: Application to Exceptional Braid Groups

  • Published:
Applied Categorical Structures Aims and scope Submit manuscript

Abstract

The homology of a Garside monoid, thus of a Garside group, can be computed efficiently through the use of the order complex defined by Dehornoy and Lafont. We construct a categorical generalization of this complex and we give some computational techniques which are useful for reducing computing time. We then use this construction to complete results of Salvetti, Callegaro and Marin regarding the homology of exceptional complex braid groups. We most notably study the case of the Borchardt braid group \(B(G_{31})\) through its associated Garside category.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

Data Availability

The first author should be contacted in order to request the data.

References

  1. Adjan, S.I.: Defining relations and algorithmic problems for groups and semigroups. Proceedings of the Steklov Institute of Mathematics. Translated from the Russian by M. Greendlinger, vol 85, pp. iii+152. American Mathematical Society, Providence (1966)

  2. Arnold, V.I.: Certain topological invariants of algebraic functions. Trudy Moskov. Mat. Obšč. 21, 27–46 (1970)

    MathSciNet  Google Scholar 

  3. Artin, E.: Theorie der Zöpfe. In: Abhandlungen aus dem Mathematischen Seminar der Universitat Hamburg 4.1, pp. 47–72 (1925). https://doi.org/10.1007/BF02950718

  4. Bannai, E.: Fundamental groups of the spaces of regular orbits of the finite unitary groups of rank 2. J. Math. Soc. Jpn. 28, 447–454 (1976). https://doi.org/10.2969/jmsj/02830447

    Article  Google Scholar 

  5. Bessis, D.: Finite complex reflection arrangements are K(p, 1). Ann. Math. (2) 181(3), 809–904 (2015). https://doi.org/10.4007/annals.2015.181.3.1

    Article  MathSciNet  Google Scholar 

  6. Bessis, D.: Garside categories, periodic loops and cyclic sets (2007). https://doi.org/10.48550/ARXIV.MATH/0610778

  7. Bestvina, M.: Non-positively curved aspects of Artin groups of finite type. Geom. Topol. 3, 269–302 (1999). https://doi.org/10.2140/gt.1999.3.269

    Article  MathSciNet  Google Scholar 

  8. Birman, J.S., Gebhardt, V., González-Meneses, J.: Conjugacy in Garside groups. I. Cyclings, powers and rigidity. Groups Geom. Dyn. 1(3), 221–279 (2007). https://doi.org/10.4171/GGD/12

    Article  MathSciNet  Google Scholar 

  9. Bosma, W., Cannon, J., Playoust, C.: The Magma algebra system. I. The user language. J. Symb. Comput. 24(3–4), 235–265 (1997)

    Article  MathSciNet  Google Scholar 

  10. Bouc, S., Thévenaz, J.: Correspondence functors and finiteness conditions. J. Algebra 495, 150–198 (2018). https://doi.org/10.1016/j.jalgebra.2017.11.010

    Article  MathSciNet  Google Scholar 

  11. Bourbaki, N.: Groupes et algèbres de Lie, chapitres 4, 5, 6. Masson (1981)

  12. Brieskorn, E.: Die Fundamentalgruppe des Raumes der regulären Orbits einer endlichen komplexen Spiegelungsgruppe. Invent. Math. 12, 57–61 (1971). https://doi.org/10.1007/BF01389827

    Article  ADS  MathSciNet  Google Scholar 

  13. Brieskorn, E., Saito, K.: Artin-Gruppen und Coxeter-Gruppen. Invent. Math. 17, 245–271 (1972). https://doi.org/10.1007/BF01406235

    Article  ADS  MathSciNet  Google Scholar 

  14. Broué, M., Malle, G., Rouquier, R.: Complex reflection groups, braid groups, Hecke algebras. J. Reine Angew. Math. 500, 127–190 (1998)

    MathSciNet  Google Scholar 

  15. Callegaro, F.: On the Cohomology of Artin groups in local systems and the associated Milnor fiber. J. Pure Appl. Algebra 197(1–3), 323–332 (2005). https://doi.org/10.1016/j.jpaa.2004.10.002

    Article  MathSciNet  Google Scholar 

  16. Callegaro, F., Marin, I.: Homology computations for complex braid groups. J. Eur. Math. Soc. (JEMS) 16(1), 103–164 (2014). https://doi.org/10.4171/JEMS/429

    Article  MathSciNet  Google Scholar 

  17. Charney, R., Meier, J., Whittlesey, K.: Bestvina’s Normal Form Complex and the Homology of Garside Groups. Geom. Dedicata 105, 171–188 (2004). https://doi.org/10.1023/B:GEOM.0000024696.69357.73

    Article  MathSciNet  Google Scholar 

  18. De Concini, C., Procesi, C., Salvetti, M.: Arithmetic properties of the cohomology of braid groups. Topology 40(4), 739–751 (2001). https://doi.org/10.1016/S0040-9383(99)00081-6

    Article  MathSciNet  Google Scholar 

  19. De Concini, C., Procesi, C., Salvetti, M., Stumbo, F.: Arithmetic properties of the cohomology of Artin groups. Ann. Scuola Norm. Sup. Pisa Cl. Sci. (4) 28(4), 695–717 (1999)

    MathSciNet  Google Scholar 

  20. Dehornoy, P., Digne, F., Godelle, E., Krammer, D., Michel, J.: Foundations of Garside Theory. EMS Tracts in Mathematics, vol. 22, p. xviii+691. European Mathematical Society (EMS), Zürich (2015). https://doi.org/10.4171/139

    Book  Google Scholar 

  21. Dehornoy, P., Lafont, Y.: Homology of Gaussian groups. Ann. Inst. Fourier (Grenoble) 53(2), 489–540 (2003)

    Article  MathSciNet  Google Scholar 

  22. Dehornoy, P., Paris, L.: Gaussian groups and Garside groups, two generalisations of Artin groups. Proc. Lond. Math. Soc. (3) 79(3), 569–604 (1999). https://doi.org/10.1112/S0024611599012071

    Article  MathSciNet  Google Scholar 

  23. Deligne, P.: Les immeubles des groupes de tresses généralisés. Invent. Math. 17, 273–302 (1972). https://doi.org/10.1007/BF01406236

    Article  ADS  MathSciNet  Google Scholar 

  24. Franco, N., González-Meneses, J.: Computation of centralizers in braid groups and Garside groups. In: Proceedings of the International Conference on Algebraic Geometry and Singularities (Spanish) (Sevilla, 2001), vol. 19(2), pp. 367–384 (2003). https://doi.org/10.4171/RMI/352

  25. Garnier, O.: Springer categories for regular centralizers in well-generated complex braid groups. arXiv:2306.13022 (2023)

  26. Garside, F.A.: The braid group and other groups. Quart. J. Math. Oxford Ser. (2) 20, 235–254 (1969). https://doi.org/10.1093/qmath/20.1.235

    Article  ADS  MathSciNet  Google Scholar 

  27. Godelle, E.: Parabolic subgroups of Garside groups. J. Algebra 317(1), 1–16 (2007). https://doi.org/10.1016/j.jalgebra.2007.05.024

    Article  MathSciNet  Google Scholar 

  28. Grayson, D.R., Stillman, M.E.: Macaulay2, a software system for research in algebraic geometry. Available at http://www.math.uiuc.edu/Macaulay2/

  29. Guerrier, W.J.: The Factorization of the Cyclotomic Polynomials mod p. Am. Math. Mon. 75, 46 (1968). https://doi.org/10.2307/2315109

    Article  MathSciNet  Google Scholar 

  30. Krammer, D.: A class of Garside groupoid structures on the pure braid group. Trans. Am. Math. Soc. 360(8), 4029–4061 (2008). https://doi.org/10.1090/S0002-9947-08-04313-4

    Article  MathSciNet  Google Scholar 

  31. Lehrer, G.I., Taylor, D.E.: Unitary Reflection Groups. Australian Mathematical Society Lecture Series, p. viii+294. Cambridge University Press, Cambridge (2009)

    Google Scholar 

  32. Marin, I.: Homology computations for complex braid groups II. J. Algebra 477, 540–547 (2017). https://doi.org/10.1016/j.jalgebra.2017.01.044

    Article  MathSciNet  Google Scholar 

  33. McDuff, D.: On the classifying spaces of discrete monoids. Topology 18(4), 313–320 (1979). https://doi.org/10.1016/0040-9383(79)90022-3

    Article  MathSciNet  Google Scholar 

  34. Michel, J.: The development version of the CHEVIE package of GAP3. J. Algebra 435, 308–336 (2015). https://doi.org/10.1016/j.jalgebra.2015.03.031

    Article  MathSciNet  Google Scholar 

  35. Mitchell, B.: Rings with several objects. Adv. Math. 8, 1–161 (1972). https://doi.org/10.1016/0001-8708(72)90002-3

    Article  MathSciNet  Google Scholar 

  36. Orlik, P., Solomon, L.: Discriminants in the invariant theory of reflection groups. Nagoya Math. J. 109, 23–45 (1988). https://doi.org/10.1017/S0027763000002749

    Article  MathSciNet  Google Scholar 

  37. Picantin, M.: Petits groupes gaussiens. PhD thesis. Université de Caen (2000)

  38. Picantin, M.: The center of thin Gaussian groups. J. Algebra 245(1), 92–122 (2001). https://doi.org/10.1006/jabr.2001.8894

    Article  MathSciNet  Google Scholar 

  39. El-Rifai, E.A., Morton, H.R.: Algorithms for positive braids. Quart. J. Math. Oxford Ser. (2) 45(180), 479–497 (1994). https://doi.org/10.1093/qmath/45.4.479

    Article  MathSciNet  Google Scholar 

  40. Salvetti, M.: The homotopy type of Artin groups. Math. Res. Lett. 1(5), 565–577 (1994). https://doi.org/10.4310/MRL.1994.v1.n5.a5

    Article  MathSciNet  Google Scholar 

  41. Shephard, G.C., Todd, J.A.: Finite unitary reflection groups. Can. J. Math. 6, 274–304 (1954). https://doi.org/10.4153/cjm-1954-028-3

    Article  MathSciNet  Google Scholar 

  42. Squier, C.: The homological algebra of Artin groups. Math. Scand. 75(1), 5–43 (1994). https://doi.org/10.7146/math.scand.a-12500

    Article  MathSciNet  Google Scholar 

  43. Vershinin, V.V.: Homology of braid groups and their generalizations. In: Knot theory (Warsaw, 1995). Vol. 42, pp. 421–446. Banach Center Publications, Polish Academy of Sciences Institute of Mathematics, Warsaw (1998)

  44. Webb, P.: An introduction to the representations and cohomology of categories. In: Group Representation Theory, pp. 149–173. EPFL Press, Lausanne (2007)

  45. Weibel, C.A.: An Introduction to Homological Algebra. Cambridge Studies in Advanced Mathematics, vol. 38, p. xiv+450. Cambridge University Press, Cambridge (1994)

    Book  Google Scholar 

Download references

Acknowledgements

The computational results for the braid groups of \(G_{24},G_{27},G_{29},G_{31},\) \(G_{33}\) and \(G_{34}\) were obtained using the MatriCS platform of the Université de Picardie Jules Verne in Amiens, France. I thank Étienne Piskorski, Laurent Renault and Jean-Baptiste Hoock for their help in using it. I also thank my PhD thesis advisor Ivan Marin for his precious help.

Funding

No funding was obtained for this study.

Author information

Authors and Affiliations

  1. LAMFA, Université de Picardie Jules Verne, CNRS UMR 7352, 33, rue Saint-Leu, 80000, Amiens, France

    Owen Garnier

Authors
  1. Owen Garnier
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

The author confirms sole responsibility for the following: study conception and design, analysis of results, and manuscript preparation.

Corresponding author

Correspondence to Owen Garnier.

Ethics declarations

Conflict of interest

The author declare that there are no competing interests.

Additional information

Communicated by Vladimir Dotsenko.

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Garnier, O. Generalization of the Dehornoy–Lafont Order Complex to Categories: Application to Exceptional Braid Groups. Appl Categor Struct 32, 1 (2024). https://doi.org/10.1007/s10485-023-09757-6

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10485-023-09757-6

Keywords

Mathematics Subject Classification

Search

Navigation