Abstract
In the current study, we explore stability of satellite motion around the natural moons of planets in solar system using the novel concept of ER3BP with variable eccentricity. This concept was introduced earlier when novel type of ER3BP (Sun–planet–satellite) was investigated with variable spin state of secondary planet correlated implicitly to the satellite motion (in the synodic co-rotating Cartesian coordinate system) for its trapped orbit near the secondary planet (which is involved in Kepler’s duet “Sun–planet”). However, it is of real interest to explore another kind of aforedescribed problem, ER3BP (planet–moon–satellite) with respect to investigation of satellite motion m around the natural moon mmoon of planet with variable eccentricity of the moon in its motion around the planet. Therefore, we consider here two primaries, Mplanet and mmoon, the latter orbiting around their common barycenter on quasi-elliptic orbit with slow-changing, not constant eccentricity (on a large-time scale) due to tidal phenomena. Our aim is to investigate the motion of a small dot satellite around the natural moon of planet on quasi-stable elliptic orbit. Both novel theoretical and numerical findings (for various cases of trio “planet–moon–satellite”) are presented in the current research.










Similar content being viewed by others
References
Cabral, F., Gil, P.: On the stability of quasi-satellite orbits in the elliptic restricted three-body problem. Master Thesis at the Universidade Técnica de Lisboa, Lisbon (2011)
Arnold, V.: Mathematical methods of classical mechanics. Springer, New York (1978)
Duboshin, G.N.: Nebesnaja mehanika. Osnovnye zadachi i metody. Nauka (handbook for Celestial Mechanics in Russian), Moscow (1968)
Szebehely, V.: Theory of orbits. The restricted problem of three bodies. Yale University, Academic Press, New Haven, Connecticut, New-York, London (1967)
Abouelmagd, E.I., Pal, A.K., Guirao, J.L.: Analysis of nominal halo orbits in the Sun–Earth system. Arch. Appl. Mech. 91(12), 4751–4763 (2021)
Ferrari, F., Lavagna, M.: Periodic motion around libration points in the elliptic restricted three-body problem. Nonlinear Dyn. 93(2), 453–462 (2018)
Llibre, J., Conxita, P.: On the elliptic restricted three-body problem. Celest. Mech. Dyn. Astron. 48(4), 319–345 (1990)
Ershkov, S., Abouelmagd, E., Rachinskaya,: A novel type of ER3BP introduced for hierarchical configuration with variable angular momentum of secondary planet. Arch. Appl. Mech. 91(11), 4599–4607 (2021)
Ershkov, S., Rachinskaya, A.: Semi-analytical solution for the trapped orbits of satellite near the planet in ER3BP. Arch. Appl. Mech. 91(4), 1407–1422 (2021)
Ershkov, S., Leshchenko, D., Rachinskaya, A.: Note on the trapped motion in ER3BP at the vicinity of barycenter. Arch. Appl. Mech. 91(3), 997–1005 (2021)
Abouelmagd, E.I., Ansari, A.A., Ullah, M.S., García Guirao, J.L.: A planar five-body problem in a framework of heterogeneous and mass variation effects. Astron. J. 160(5), 216 (2020)
Ershkov, S., Leshchenko, D., Abouelmagd, E.: About influence of differential rotation in convection zone of gaseous or fluid giant planet (Uranus) onto the parameters of orbits of satellites. Eur. Phys. J. Plus 136, 387 (2021)
Ershkov, S., Leshchenko, D.: On the stability of Laplace resonance for Galilean moons (Io, Europa, Ganymede). Anais da Academia Brasileira de Ciências (Ann. Braz. Acad. Sci.) 93(4), e20201016 (2021)
Ershkov, S.V.: About tidal evolution of quasi-periodic orbits of satellites. Earth Moon Planet. 120(1), 15–30 (2017)
Ershkov, S., Leshchenko, D., Rachinskaya, A.: Revisiting the dynamics of finite-sized satellite near the planet in ER3BP. Arch. Appl. Mech. 92(8), 2397–2407 (2022)
Ershkov, S., Leshchenko, D., Rachinskaya, A.: Capture in regime of a trapped motion with further inelastic collision for finite-sized asteroid in ER3BP. Symmetry 14(8), 1548 (2022)
Ershkov, S.V., Leshchenko, D.: Solving procedure for 3D motions near libration points in CR3BP. Astrophys. Space Sci. 364, 207 (2019)
Ershkov, S., Leshchenko, D., Prosviryakov, E.Yu.: A novel type of ER3BP introducing Milankovitch cycles or seasonal irradiation processes influencing onto orbit of planet. Arch. Appl. Mech. 93, 813–822 (2023)
Ferraz-Mello, S., Rodríguez, A., Hussmann, H.: Tidal friction in close-in satellites and exoplanets: the Darwin theory re-visited. Celest. Mech. Dyn. Astr. 101, 171–201 (2008). https://doi.org/10.1007/s10569-008-9133-x
Singh, J., Umar, A.: On motion around the collinear libration points in the elliptic R3BP with a bigger triaxial primary. New Astron. 29, 36–41 (2014)
Zotos, E.E.: Crash test for the Copenhagen problem with oblateness. Celest. Mech. Dyn. Astron. 122(1), 75–99 (2015)
Abouelmagd, E.I., Sharaf, M.A.: The motion around the libration points in the restricted three-body problem with the effect of radiation and oblateness. Astrophys. Space Sci. 344(2), 321–332 (2013)
Kushvah, B.S., Sharma, J.P., Ishwar, B.: Nonlinear stability in the generalised photogravitational restricted three body problem with Poynting-Robertson drag. Astrophys. Space Sci. 312(3–4), 279–293 (2007)
Nekhoroshev, N.N.: Exponential estimate on the stability time of near integrable Hamiltonian systems. Russ Math. Survey 32, N6 (1977)
Lidov, M.L., Vashkov’yak, M.A.: Theory of perturbations and analysis of the evolution of quasi-satellite orbits in the restricted three-body problem. Kosmicheskie Issledovaniia 31, 75–99 (1993)
Peale, S.J.: Orbital resonances in the solar system. Ann. Rev. Astron. Astro-Phys. 14, 215–246 (1976)
Wiegert, P., Innanen, K., Mikkola, S.: The stability of quasi satellites in the outer solar system. Astron. J. 119, 1978–1984 (2000). https://doi.org/10.1086/301291
Lhotka C.: Nekhoroshev stability in the elliptic restricted three body problem. Thesis for: Doktor reris naturalis (2008). https://doi.org/10.13140/RG.2.1.2101.3848
Abouelmagd, E.I., Mortari, D., Selim, H.H.: Analytical study of periodic solutions on perturbed equatorial two-body problem. Int. J. Bifurc. Chaos 25(14), 1540040 (2015)
Abouelmagd, E.I.: Periodic solution of the two-body problem by KB averaging method within frame of the modified newtonian potential. J. Astronaut. Sci. 65(3), 291–306 (2018)
Alshaery, A.A., Abouelmagd, E.I.: Analysis of the spatial quantized three-body problem. Results Phys. 17, 103067 (2020)
Abouelmagd, E.I., Llibre, J., Guirao, J.L.G.: Periodic orbits of the planar anisotropic Kepler problem. Int. J. Bifurc. Chaos 27(3), 1750039 (2017)
Abouelmagd, E.I., Guirao, J.L.G., Pal, A.K.: Periodic solution of the nonlinear Sitnikov restricted three-body problem. New Astron. 75, 101319 (2020)
Emelyanov, N.V.: Influence of tides in viscoelastic bodies of planet and satellite on the satellite’s orbital motion. Mon. Not. R. Astron. Soc. 479(1), 1278–1286 (2018)
Lu, T., et al.: Self-consistent spin, tidal, and dynamical equations of motion in the REBOUNDx framework. Astrophys. J. 948(1), 41 (2023). https://doi.org/10.3847/1538-4357/acc06d
Ershkov, S., Leshchenko, D., Prosviryakov, E.Y., Abouelmagd, E.I.: Finite-sized orbiter’s motion around the natural moons of planets with slow-variable eccentricity of their orbit in ER3BP. Mathematics 11, 3147 (2023). https://doi.org/10.3390/math11143147
Efroimsky, M., Lainey, V.: Physics of bodily tides in terrestrial planets and the appropriate scales of dynamical evolution. J. Geophys. Res. (2007). https://doi.org/10.1029/2007JE002908
Efroimsky, M., Makarov, V.V.: Tidal friction and tidal lagging. Applicability limitations of a popular formula for the tidal torque. Astrophys. J. 764, 26 (2013)
Peale, S.J., Cassen, P.: Contribution of tidal dissipation to lunar thermal history. Icarus 36, 245–269 (1978)
Reid, M.J.: The tidal loss of satellite-orbiting objects and implications for the lunar surface. Icarus 20, 240–248 (1973)
Gold, T.: Remarks on the paper “ The tidal loss of satellite-orbiting objects and implications for the lunar surface” by Mark. J. Reid. Icarus 24, 134–135 (1975)
Kollmeier, J.A., Raymond, S.N.: Can moons have moons? Mon. Not. R. Astron. Soc. Lett. 483(1), L80–L8 (2019). https://doi.org/10.1093/mnrasl/sly219
Rosario-Franco, M., et al.: Orbital stability of exomoons and submoons with applications to Kepler 1625b-I. Astron. J. 159, 260 (2020). https://doi.org/10.3847/1538-3881/ab89a7
Quarles, B., Li, G., Rosario-Franco, M.: Application of orbital stability and tidal migration constraints for exomoon candidates. Astrophys. J. Lett. 902(1), L20 (2020). https://doi.org/10.3847/2041-8213/abba36
Moraes, R.A., Vieira Neto, E.: Exploring formation scenarios for the exomoon candidate Kepler 1625b I. Mon. Not. R. Astron. Soc. 495(4), 3763–3776 (2020). https://doi.org/10.1093/mnras/staa1441
Ershkov, S., Prosviryakov, E., Leshchenko, D., Burmasheva, N.: Semianalytical findings for the dynamics of the charged particle in the Störmer problem. Math. Methods. Appl. Sci. 46(18), 19364–19376 (2023). https://doi.org/10.1002/mma.9631
Ershkov, S.V., Leshchenko, D.: On the dynamics OF NON-RIGID asteroid rotation. Acta Astronaut. 161, 40–43 (2019). https://doi.org/10.1016/j.actaastro.2019.05.011
Author information
Authors and Affiliations
Contributions
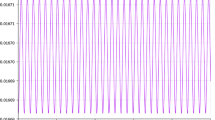
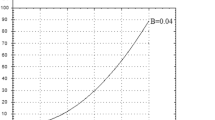
In this research, S.E. is responsible for the general ansatz and the solving procedure, simple algebra manipulations, calculations, results of the article and also is responsible for the search of analytical and semi-analytical solutions. D.L. is responsible for theoretical investigations as well as for the deep survey in the literature on the problem under consideration. E.P. is responsible for obtaining numerical solutions related to approximated ones (including their graphical plots). All authors agreed with results and conclusions of each other in Sections 1-4.
Corresponding author
Ethics declarations
Competing interests
The authors declare no competing interests.
Conflict of interest
On behalf of all authors, the corresponding author states that there is no conflict of interest.
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendix (mathematical procedure of derivation of Eq. (11)).
Appendix (mathematical procedure of derivation of Eq. (11)).
Let us present mathematical procedure of derivation of Eq. (11) as follows:
Since we consider that main contribution influencing on the orbit of moon in its motion around the host planet stems from the tides raised on the surface of planet by the moon orbiting in the 1:1 spin–orbit resonance around the planet, we can use formulae (3.2) obtained in [14], e.g., dynamical invariant which interrelates semimajor axis with respect to the eccentricity ({a1, e1} = {ap (0), e (0)} = const):
where the term: exp ((51/19)⋅(e2 − e12)) ≅ 1. For the reason that time t has not been involved to be presented in expressions in both parts of (14), we can change the independent variable t → f in (14) for {ap (t), e(t)} → {ap(f), e(f)} (and vice versa) without losing a generality for the dynamical invariant (14). Let us present Eq. (1) in other form as below
which can be transformed in case of low-eccentricity orbit e ≅ 0 (by neglecting of terms of second-order smallness in (15)) as follows
(let us remind that we have chosen e1 = e0, a1 = a0 in (11) just for simplicity of presentation of the final result). While in (16) eccentricity e is a very slowly varying function on a long-time scale period, it could be considered equal to constant in Eq. (16) for the sufficiently large period of changing of true anomaly f. Thus, in (16) we have obtained the equation solution of which approximately results to (9) as follows (see [8]):
Rights and permissions
Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.
About this article
Cite this article
Ershkov, S., Leshchenko, D. & Prosviryakov, E.Y. Illuminating dot-satellite motion around the natural moons of planets using the concept of ER3BP with variable eccentricity. Arch Appl Mech 94, 515–527 (2024). https://doi.org/10.1007/s00419-023-02533-x
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00419-023-02533-x