Skip to main content
SpringerLink
Log in

Numerical methods for the forward and backward problems of a time-space fractional diffusion equation

  • Published:
Calcolo Aims and scope Submit manuscript

Abstract

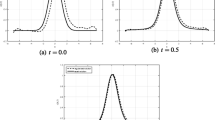
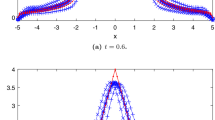
In this paper, we consider the numerical methods for both the forward and backward problems of a time-space fractional diffusion equation. For the two-dimensional forward problem, we propose a finite difference method. The stability of the scheme and the corresponding Fast Preconditioned Conjugated Gradient algorithm are given. For the backward problem, since it is ill-posed, we use a quasi-boundary-value method to deal with it. Based on the Fourier transform, we obtain two kinds of order optimal convergence rates by using an a-priori and an a-posteriori regularization parameter choice rules. Numerical examples for both forward and backward problems show that the proposed numerical methods work well.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11

Similar content being viewed by others

References

  1. Alikhonov, A.A.: A new difference scheme for the time fractional diffusion equation. J. Comput. Phys. 280, 424–438 (2015)

    MathSciNet  Google Scholar 

  2. Ames, K.A., Epperson, J.F.: A kernel-based method for the approximate solution of backward parabolic problems. SIAM J. Numer. Anal. 34(4), 1357–1390 (1997)

    MathSciNet  Google Scholar 

  3. Bessila, K.: Regularization by a modified quasi-boundary value method of the ill-posed problems for differential-operator equations of the first order. J. Math. Anal. Appl. 409, 315–302 (2014)

    MathSciNet  Google Scholar 

  4. Carasso, A.S.: The APEX method in image sharpening and the use of low exponent Lévy stable laws. SIAM J. Appl. Math. 63(2), 593–618 (2002)

    MathSciNet  Google Scholar 

  5. Celik, C., Duman, M.: Crank–Nicolson method for the fractional diffusion equation with the Riesz fractional derivative. J. Comput. Phys. 231, 1743–1750 (2012)

    MathSciNet  Google Scholar 

  6. Chan, R., Jin, X.: A family of block preconditioners for block systems. SIAM J. Sci. Stat. Comput. 13, 1218–1235 (1992)

    MathSciNet  Google Scholar 

  7. Chen, Z., Meerschaert, M.M., Nane, E.: Space-time fractional diffusion on bounded domains. J. Math. Anal. Appl. 393(2), 479–488 (2012)

    MathSciNet  Google Scholar 

  8. Chen, Z., Kim, K.H., Kim, P.: Fractional time stochastic partial differential equations. Stoch. Proc. Appl. 125(4), 1470–1499 (2015)

    MathSciNet  Google Scholar 

  9. Clark, G.W., Oppenheimer, S.F.: Quasi reversibility methods for non-well-posed problems. Electron. J. Differ. equ. 1994(8), 1–9 (1994)

    Google Scholar 

  10. Clément, P., Gripenberg, G., Londen, S.O.: Schauder estimates for equations with fractional derivatives. Trans. Am. Math. Soc. 352(5), 2239–2260 (2000)

    MathSciNet  Google Scholar 

  11. Clément, P., Londen, S.O., Simonett, G.: Quasilinear evolutionary equations and continuous interpolation spaces. J. Differ. Equ. 196(2), 418–447 (2004)

    MathSciNet  Google Scholar 

  12. Denche, M., Bessila, K.: A modified quasi-boundary value method for ill-posed problems. J. Math. Anal. 301, 419–426 (2005)

    MathSciNet  Google Scholar 

  13. Denche, M., Djezzar, S.: A modified quasi-boundary value method for a class of abstract parabolic ill-posed problems. Bound. Value. Probl. 2005, 1–8 (2006)

    MathSciNet  Google Scholar 

  14. Deng, W.: Finite element method for the space and time fractional Fokker–Planck equation. SIAM J. Numer. Anal. 47(1), 204–226 (2009)

    MathSciNet  Google Scholar 

  15. Deng, Z., Yang, X.: A discretized Tikhonov regularization method for a fractional backward heat conduction problem. Abstr. Appl. Anal. 2014, 964373 (2014)

    MathSciNet  Google Scholar 

  16. Dou, F., Hon, Y.C.: Numerical computation for backward time-fractional diffusion equation. Eng. Anal. Bound. Elem. 40, 138–146 (2014)

    MathSciNet  Google Scholar 

  17. Dou, F., Hon, Y.C.: Fundamental kernel-based method for backward space-time fractional diffusion problem. Comput. Math. Appl. 71(1), 356–367 (2016)

    MathSciNet  Google Scholar 

  18. Du, N., Wang, H.: A fast finite element method for space-fractional dispersion equations on bounded domains in \(\mathbb{R} ^2\). SIAM J. Sci. Comput. 37, A1614–A1635 (2015)

    Google Scholar 

  19. Duo, S., Zhang, Y.: Accurate numerical methods for two and three dimensional integral fractional Laplacian with applications. Comput. Methods Appl. Mech. Eng. 355, 639–662 (2019)

    MathSciNet  Google Scholar 

  20. Feng, X., Eldén, L., Fu, C.: A quasi-boundary-value method for the Cauchy problem for elliptic equations with nonhomogeneous Neumann data. J. Inverse Ill-Posed Probl. 18, 617–645 (2010)

    MathSciNet  Google Scholar 

  21. Feng, X., Ning, W., Qian, Z.: A quasi-boundary-value method for a Cauchy problem of an elliptic equation in multiple dimensions. Inverse Probl. Sci. Eng. 22(7), 1045–1061 (2014)

    MathSciNet  Google Scholar 

  22. Feng, X., Zhao, M., Qian, Z.: A Tikhonov regularization method for solving a backward time-space fractional diffusion problem. J. Comput. Appl. Math. 411, 114236 (2022)

    MathSciNet  Google Scholar 

  23. Fu, H., Wang, H.: A preconditioned fast parareal finite difference method for space-time fractional partial differential equation. J. Sci. Comput. 78, 1724–1743 (2019)

    MathSciNet  Google Scholar 

  24. Fu, C., Xiong, X., Qian, Z.: Fourier regularization for a backward heat equation. J. Math. Anal. Appl. 331, 472–480 (2007)

    MathSciNet  Google Scholar 

  25. Gorenflo, R., Mainardi, F., Moretti, D., Pagnini, G., Paradisi, P.: Discrete random walk models for space-time fractional diffusion. Chem. Phys. 284, 521–541 (2002)

    CAS  Google Scholar 

  26. Guo, S., Mei, L., Zhang, Z., Li, C., Li, M., Wang, Y.: A linearized finite difference/spectral-Galerkin scheme for three-dimensional distributed-order time-Cspace fractional nonlinear reaction-diffusion-wave equation: Numerical simulations of Gordon-type solitons. Comput. Phys. Commun. 252, 107144 (2020)

    MathSciNet  CAS  Google Scholar 

  27. Hào, D.N., Duc, N.V., Sahli, H.: A non-local boundary value problem method for parabolic equations backward in time. J. Math. Anal. Appl. 345, 805–815 (2008)

    MathSciNet  Google Scholar 

  28. Hào, D.N., Duc, N.V., Lesnic, D.: Regularization of parabolic equations backward in time by a non-local boundary value problem method, IMA. J. Appl. Math. 75, 291–315 (2010)

    MathSciNet  Google Scholar 

  29. Hào, D.N., Liu, J.J., Duc, N.V., Thang, N.V.: Stability results for backward time-fractional parabolic equations. Inverse Probl. 35(12), 125006 (2019)

    MathSciNet  Google Scholar 

  30. Hao, Z., Zhang, Z., Du, R.: Fractional centered difference scheme for high-dimensional integral fractional Laplacian. J. Comput. Phys. 424, 109851 (2021)

    MathSciNet  Google Scholar 

  31. Hendy, A.S., Zaky, M.A., Staelen, R.H.D.: A general framework for the numerical analysis of high-order finite difference solvers for nonlinear multi-term time-space fractional partial differential equations with time delay. Appl. Numer. Math. 169, 108–121 (2021)

    MathSciNet  Google Scholar 

  32. Jayakumar, K.: Modified quasi-boundary value method for the multidimensional nonhomogeneous backward time fractional diffusion equation. Math. Methods Appl. Sci. 44(10), 8363–8378 (2021)

    MathSciNet  Google Scholar 

  33. Jia, J., Peng, J., Gao, J., Li, Y.: Backward problem for a time-space fractional diffusion equation. Inverse Probl. Imaging 12(3), 773–799 (2018)

    MathSciNet  Google Scholar 

  34. Jin, B., Rundell, W.: A tutorial on inverse problems for anomalous diffusion processes. Inverse Probl. 31(3), 035003 (2015)

    MathSciNet  Google Scholar 

  35. Kaltenbacher, B., Rundell, W.: Regularization of a backwards parabolic equation by fractional operators. Inverse Probl. Imaging 13(2), 401–430 (2019)

    MathSciNet  Google Scholar 

  36. Kilbas, A., Srivastava, H.M., Trujillo, J.: Theory and Applications of Fractional Differential Equations. Elsevier, Amsterdam (2006)

    Google Scholar 

  37. Kim, K.H., Lim, S.: Asymptotic behaviors of fundamental solution and its derivatives related to space-time fractional differential equations. Mathematics 392(392), 31 (2015)

    Google Scholar 

  38. Kim, I., Kim, K.H., Lim, S.: An \(L_q(L_p)\)-theory for the time fractional evolution equations with variable coefficients. Adv. Math. 306, 123–176 (2017)

    MathSciNet  Google Scholar 

  39. Kirkup, S.M., Wadsworth, M.: Solution of inverse diffusion problems by operator-splitting methods. Appl. Math. Model. 26, 1003–1018 (2002)

    Google Scholar 

  40. Li, M., Xiong, X.: On a fractional backward heat conduction problem: Application to deblurring. Comput. Math. Appl. 64, 2594–2602 (2012)

    MathSciNet  Google Scholar 

  41. Liu, N.: Inverse initial value problem for a space-time fractional diffusion equation. In: Master Thesis (Lanzhou University, 2017)

  42. Liu, J., Xiao, M.: Quasi-boundary value methods for regularizing the backward parabolic equation under the optimal control framework. Inverse Probl. 35, 124003 (2019)

    MathSciNet  Google Scholar 

  43. Liu, J., Yamamoto, M.: A backward problem for the time-fractional diffusion equation. Appl. Anal. 89(11), 1769–1788 (2010)

    MathSciNet  Google Scholar 

  44. Mainardi, F., Luchko, Y., Pagnini, G.: The fundamental solution of the space-time fractional diffusion equation. Fract. Calc. Appl. Anal. 2, 153–192 (2001)

    MathSciNet  Google Scholar 

  45. Meerschaert, M.M., Schilling, R.L., Sikorskii, A.: Stochastic solutions for fractional wave equations. Nonlinear Dyn. 80(4), 1685–1695 (2015)

    MathSciNet  PubMed  Google Scholar 

  46. Metzler, R., Klafter, J.: Boundary value problems for fractional diffusion equations. Physica A 278(1), 107–125 (2000)

    MathSciNet  CAS  Google Scholar 

  47. Metzler, R., Klafter, J.: The random walks guide to anomalous diffusion: a fractional dynamics approach. Phys. Rep. 339(1), 1–77 (2000)

    MathSciNet  CAS  Google Scholar 

  48. Murio, D.A.: Implicit finite difference approximation for time fractional diffusion equations. Comput. Math. Appl. 56, 1138–1145 (2008)

    MathSciNet  Google Scholar 

  49. Natterer, F.: Error bounds for Tikhonov regularization in Hilbert scales. Appl. Anal. 18, 29–37 (1984)

    MathSciNet  Google Scholar 

  50. Ortigueira, M.D.: Riesz potential operators and inverse via fractional centred derivatives. Int. J. Math. Sci. 2006, 1–12 (2006)

    Google Scholar 

  51. Podlubny, I.: Fractional Differential Equations: An Introduction to Fractional Derivatives, Fractional Differential Equations, to Methods of their Solution and some of their Applications, in: Mathematics in Science and Engineering, vol. 198, Academic Press Inc., San Diego, CA, (1999)

  52. Podlubny, I.: Mittag–Leffler function. https://www.mathworks.com/matlabcentral/fileexchange/8738-mittagleffler-function. Accessed 07 Sep 2012 (2012)

  53. Qian, Z., Feng, X.: A fractional Tikhonov method for solving a Cauchy problem of Helmholtz equation. Appl. Anal. 96(10), 1656–1668 (2017)

    MathSciNet  Google Scholar 

  54. Qian, A., Yang, X., Wu, Y.: Optimal error bound and a quasi-boundary value regularization method for a Cauchy problem of the modified Helmholtz equation. Int. J. Comput. Math. 93(12), 2028–2041 (2016)

    MathSciNet  Google Scholar 

  55. Quan, P.H., Trong, D.D., Tuan, N.H.: A new version of quasi-boundary value method for a 1-D nonlinear ill-posed heat problem. J. Inverse Ill-Posed Probl. 17, 913–932 (2009)

    MathSciNet  Google Scholar 

  56. Quan, P.H., Trong, D.D., Triet, L.M., Tuan, N.H.: A modified quasi-boundary value method for regularizing of a backward problem with time-dependent coefficient. Inverse Probl. Sci. Eng. 19(3), 409–423 (2011)

    MathSciNet  Google Scholar 

  57. Ruan, Z., Wang, Z., Zhang, W.: A directly numerical algorithm for a backward time-fractional diffusion equation based on the finite element method. Math. Probl. Eng. 2015, 8 (2015)

    MathSciNet  Google Scholar 

  58. Ruan, Z., Zhang, S., Xiong, S.: Solving an inverse source problem for a time fractional diffusion equation by a modified quasi-boundary value method. Evol. Equ. Control 7(4), 669–682 (2018)

    MathSciNet  Google Scholar 

  59. Saichev, A.I., Zaslavsky, G.M.: Fractional kinetic equations: solutions and applications. Chaos 7(4), 753–764 (1997)

    MathSciNet  CAS  PubMed  Google Scholar 

  60. Sakamoto, K., Yamamoto, M.: Initial value/boundary value problems for fractional diffusion-wave equations and applications to some inverse problems. J. Math. Anal. Appl. 382, 426–447 (2011)

    MathSciNet  Google Scholar 

  61. Showalter, R.E.: The final value problem for evolution equations. J. Math. Anal. Appl. 47, 563–572 (1974)

    MathSciNet  Google Scholar 

  62. Sun, H., Sun, Z., Gao, G.: Some high order difference schemes for the space and time fractional Bloch–Torrey equations. Appl. Math. Comput. 281, 356–380 (2016)

    MathSciNet  Google Scholar 

  63. Tang, T., Wang, L., Yuan, H., Zhou, T.: Rational spectral methods for PDEs involving fractional Laplacian in unbounded domains. SIAM J. Sci. Comput. 42(2), A585–A611 (2020)

    MathSciNet  Google Scholar 

  64. Trong, D.D., Quan, P.H., Tuan, N.H.: A quasi-boundary value methods for regularizing nonlinear ill-posed problems, Electron. J. Differ. Equ. 109, 1–16 (2009)

    Google Scholar 

  65. Trong, D.D., Hai, D.N.D., Dien, N.M.: On a time-space fractional backward diffusion problem with inexact orders. Comput. Math. Appl. 78, 1572–1593 (2019)

    MathSciNet  Google Scholar 

  66. Tuan, N.H., Long, L.D., Tatar, S.: Tikhonov regularization method for a backward problem for the inhomogeneous time-fractional diffusion equation. Appl. Anal. 97(5), 842–863 (2018)

    MathSciNet  Google Scholar 

  67. Wang, L., Liu, J.: Total variation regularization for a backward time-fractional diffusion problem. Inverse Probl. 29, 115013 (2013)

    MathSciNet  Google Scholar 

  68. Wang, J., Wei, T., Zhou, Y.: Tikhonov regularization method for a backward problem for the time-fractional diffusion equation. Appl. Math. Model. 37, 8518–8532 (2013)

    MathSciNet  Google Scholar 

  69. Wang, J., Zhou, Y., Wei, T.: A posteriori regularization parameter choice rule for the quasi-boundary value method for the backward time-fractional diffusion problem. Appl. Math. Lett. 26, 741–747 (2013)

    MathSciNet  Google Scholar 

  70. Wang, J., Xiong, X., Cao, X.: Fractional Tikhonov regularization method for a time-fractional backward heat equation with a fractional Laplacian. J. Partial Differ. Equ. 31(4), 332–342 (2018)

    MathSciNet  Google Scholar 

  71. Wei, T., Wang, J.: A modified quasi-boundary value method for the backward time-fractional diffusion problem. ESAIM-Math. Model. Numer. 48, 603–621 (2014)

    MathSciNet  Google Scholar 

  72. Wei, T., Wang, J.: A modified quasi-boundary value method for an inverse source problem of the time-fractional diffusion equation. Appl. Numer. Math. 78, 95–111 (2014)

    MathSciNet  Google Scholar 

  73. Xie, C., Fang, S.: Finite difference scheme for time-space fractional diffusion equation with fractional boundary conditions. Math. Methods Appl. Sci. 43, 3473–3487 (2020)

    MathSciNet  Google Scholar 

  74. Xiong, X., Wang, J., Li, M.: An optimal method for fractional heat conduction problem backward in time. Appl. Anal. 91(4), 823–840 (2012)

    MathSciNet  Google Scholar 

  75. Xu, T., Liu, F., Lü, S., Anh, V.V.: Finite difference/finite element method for two-dimensional time-space fractional Bloch–Torrey equations with variable coefficients on irregular convex domains. Comput. Math. Appl. 80, 3173–3192 (2020)

    MathSciNet  Google Scholar 

  76. Yang, M., Liu, J.: Solving a final value fractional diffusion problem by boundary condition regularization. Appl. Numer. Math. 66, 45–58 (2013)

    MathSciNet  Google Scholar 

  77. Yang, M., Liu, J.: Fourier regularization for a final value time-fractional diffusion problem. Appl. Anal. 94(7), 1508–1526 (2015)

    MathSciNet  Google Scholar 

  78. Yang, Q., Turner, I., Liu, F., Ilić, M.: Novel numerical methods for solving the time-space fractional diffusion equation in two dimensions. SIAM J. Sci. Comput. 33(3), 1159–1180 (2011)

    MathSciNet  Google Scholar 

  79. Yang, F., Fu, C., Li, X.: A quasi-boundary value regularization method for determining the heat source. Math. Methods Appl. Sci. 37, 3026–3035 (2014)

    MathSciNet  Google Scholar 

  80. Yang, F., Zhang, M., Li, X.: A quasi-boundary value regularization method for identifying an unknown source in the Poisson equation. J. Inequal. Appl. 2014, 1–11 (2014)

    MathSciNet  Google Scholar 

  81. Yang, F., Zhang, Y., Li, X., Huang, C.: The quasi-boundary value regularization method for identifying the initial value with discrete random noise. Bound. Value. Probl. 2018, 1–12 (2018)

    MathSciNet  Google Scholar 

  82. Yang, Z., Liu, F., Nie, Y., Turner, I.: An unstructured mesh finite difference/finite element method for the three-dimensional time-space fractional Bloch-Torrey equations on irregular domains. J. Comput. Phys. 408, 109284 (2020)

    MathSciNet  Google Scholar 

  83. Yang, F., Zhang, Y., Liu, X., Li, X.: The quasi-boundary value method for identifying the initial value of the space-time fractional diffusion equation. Acta. Math. Sci. 40B(3), 641–658 (2020)

    MathSciNet  Google Scholar 

  84. Zacher, R.: Maximal regularity of type \(L_p\) for abstract parabolic Volterra equations. J. Evol. Equ. 5(1), 79–103 (2005)

    MathSciNet  Google Scholar 

  85. Zhang, H.: Modified quasi-boundary value method for Cauchy problems of elliptic equations with variable coefficients. Electron. J. Differ. Equ. 106, 1–10 (2011)

    MathSciNet  CAS  Google Scholar 

  86. Zhang, Z., Zhou, Z.: Numerical analysis of backward subdiffusion problems. Inverse Probl. 36, 105006 (2020)

    MathSciNet  Google Scholar 

  87. Zheng, G., Wei, T.: Two regularization methods for solving a Riesz–Feller space-fractional backward diffusion problem. Inverse Probl. 26, 115017 (2010)

    MathSciNet  Google Scholar 

  88. Zheng, G., Zhang, Q.: Solving the backward problem for space-fractional diffusion equation by a fractional Tikhonov regularization method. Math. Comput. Simul. 148, 37–47 (2018)

    MathSciNet  Google Scholar 

Download references

Acknowledgements

The authors would like to offer their cordial thanks to the editorial board and the reviewers of this paper for their valuable comments and suggestions, without these suggestions there would be no present form of this paper.

Author information

Authors and Affiliations

  1. School of Mathematics and Statistics, Xidian University, Xi’an, 713200, People’s Republic of China

    Xiaoli Feng, Xiaoyu Yuan & Meixia Zhao

  2. Department of Mathematics, Nanjing University, Nanjing, 210093, People’s Republic of China

    Zhi Qian

Authors
  1. Xiaoli Feng
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Xiaoyu Yuan
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Meixia Zhao
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Zhi Qian
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Xiaoli Feng.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

The work is supported by the Natural Science Basic Research Program of Shaanxi (Program Nos. 2024JC-YBMS-034, 2024JC-YBQN-0050, 2023-JC-YB-054) and the National Natural Science Foundation of China (Nos. 61877046, 12071214).

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Feng, X., Yuan, X., Zhao, M. et al. Numerical methods for the forward and backward problems of a time-space fractional diffusion equation. Calcolo 61, 16 (2024). https://doi.org/10.1007/s10092-024-00567-3

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10092-024-00567-3

Keywords

Mathematics Subject Classification

Search

Navigation