1 Introduction

Over the past 80 years, the correlation between the density of states (DOS) and physical properties such as electrical resistivity [1], Seebeck coefficient [2,3,4], and thermal conductivity (κ) [5] have been investigated. In 1936, Mott [6,7,8] reported that the electrical resistivity for transition metals is dominated by the transition of the s-electron to the DOS of the d-state at the Fermi level that now constitutes “Mott’s s–d scattering model”. Aisaka and Shimizu [5] reported that the electrical resistivity and the Seebeck coefficient of the transition metals in a solid state can be explained using the extended Mott’s s–d scattering model. In 1986, Zinov’yev et al. [9] measured the thermal diffusivity (α) of transition metals in a liquid state using a plane temperature wave method and investigated the relationship between the thermophysical properties in a liquid state and the DOS for transition metals. However, Zinov’yev et al. did not find a linear relationship between α and the reciprocal DOS for two reasons:

  1. 1

    The plane temperature wave method may not be valid for the thermal diffusivity measurement because of circulation flows arising from temperature gradients in the sample as pointed out by Assael et al. [10].

  2. 2

    Zionv’yev used the DOS at 300 K to investigate the relationship between the DOS and α at the liquid state.

Our group developed a laboratory facility called PROSPECT for high accuracy measurement of thermophysical properties of matter in the liquid state, [11,12,13,14,15,16,17,18,19,20,21,22]. PROSPECT exploits an electromagnetic levitation technique (EML) and employs a static magnetic field that can suppress convection, translational motion, and surface vibrations in levitated melts. We investigated the relationship between α and reciprocal DOS for six different transition metals in 2021 [23]. We concluded that α is proportional to the reciprocal DOS at Fermi level ((N(EF))−1) for these six transition metals in the liquid state that can be explained by Mott’s s–d scattering model.

For vanadium melts, Zinov’yev et al. [9] measured α using the plane-temperature method, and Pottlacher et al. [24] evaluated α from the measured electric resistivity using the Wiedemann–Franz law. This law may underestimate the thermal conductivity of vanadium melts because it does not consider contributions to heat transfer from the thermal vibration of atoms. To discuss α of the V melt using the s–d scattering model, an accurate α of the V melt is required. The purpose of this study is to first measure the density (ρ), normal spectral emissivity (ε), heat capacity at constant pressure (CP), and electrical resistivity (κ) to determine a of the V melt and then discuss the mechanism underlying thermal transport of transition metals in the liquid state based on Mott’s s–d scattering model.

2 Experimental

The purity of the vanadium sample was 99.9 mass%, the supplier being Rare Metallic Co. Ltd, Japan. After experiments, the oxygen contamination in the sample ranged from 0.02 mass% to 0.18 mass%, which was measured using a LECO elemental gas analyzer (ON-836, LECO, St. Joseph, USA).

The experimental details have been reported elsewhere [11, 14, 23], and therefore the procedure is only outlined here. The experimental apparatus (PROSPECT; see Fig. 1) involves both a turbomolecular and a rotary pump to evacuate the chamber up to 10−3 Pa. After evacuation, the chamber is filled with Ar-5 vol% H2 gas up to 0.1 MPa to avoid sample oxidation. Then, an EML coil carrying an AC current ranging from 310 A to 350 A levitates the sample while a radio-frequency generator (Easy Heat 8310, Ameritherm Inc., New York, USA) heats it. Employing a superconducting magnet (JMTD-10T 120SSFX, Japan Superconductor Technology, Kobe, Japan), a static magnetic field is applied to suppress the surface vibrations, translational motion, and convection of the levitated melt.

Fig. 1
figure 1

Schematic of the experimental apparatus (PROSPECT)

Full size image

A single-color pyrometer (detection wavelength range: 1.45–1.8 µm, IGA140/MB25, IMPAC Pyrometer, LumaSense Technologies, Germany), which was calibrated using the sample’s emissivity at melting point (2183 K), records the sample temperature [25]. In the determination of this temperature, the emissivity of the sample in the liquid state was assumed the same as that at the melting point. Helium gas is supplied to the chamber to control the sample temperature.

2.1 Density Measurement

Shadow images of the droplet, levitated electromagnetically under a static magnetic field of 4 T, were obtained from the horizontal direction using a shadowgraph optical system, consisting of a YAG laser (532 nm), a beam expander, and a high-speed camera. The frame rate of the high-speed camera was set at 150 fps and sample images were recorded for 20 s for each temperature setting. Three stainless-steel balls of different diameters (4.998 mm, 6.348 mm, and 7.000 mm) were imaged with the same optical system in converting pixels spanned into lengths.

The sample density (ρ) is defined as

$$\rho =\frac{m}{V},$$
(1)

where m and V denote the sample mass and volume, respectively. The sample mass was measured before and after each density measurement to evaluate the uncertainty through sample evaporation. The sample volume was determined from average values of the calculated volumes, obtained from the shadowgraph images assuming the levitated samples were rotationally symmetric around the vertical axis.

2.2 Normal Spectral Emissivity Measurement

The normal spectral emissivity (ε) of samples, levitated under a field of 3 T, is expressed as

$$\varepsilon \left(\lambda , T\right)=\frac{{E}_{{\text{s}}}(\lambda , T)}{{E}_{{\text{B}}}\left(\lambda , T\right)},$$
(2)
$${E}_{{\text{B}}}\left(\lambda , T\right)=\frac{2\pi h{{c}_{0}}^{2}}{{\lambda }^{5}\left({\text{exp}}\left(\frac{hc}{\lambda {k}_{{\text{B}}}T}\right)-1\right)},$$
(3)

where Es(λ, T) and EB(λ, T) denote the normal spectral emissive power of the sample and a blackbody, respectively, λ denotes wavelength, T temperature, h Planck’s constant, kB Boltzmann’s constant, and c the speed of light. EB(λ, T) is Planck’s radiation law. Es(λ, T) is measured using a multichannel spectrometer (wavelength range (530–1100) nm, USB2000, Ocean Optics Inc., FL, USA) and is obtained from

$${E}_{{\text{s}}}\left(\lambda , T\right)={{\text{C}}}_{0}\left(\lambda \right)\bullet X\left(\lambda , T\right),$$
(4)

where X(λ, T) denotes the output count of the multichannel spectrometer, measured from the top of the sample droplet, and C0(λ) is a coefficient of proportionality determined from the calibration of the spectrometer using a quasi-blackbody component [14].

2.3 Laser Modulation Calorimetry

In the laser modulation calorimetry method, the top of the levitated sample was heated in a periodic manner by a semiconductor laser (laser wavelength: (940 ± 20) nm, maximum power: 67.5 W, SPOLD L13920-511, Hamamatsu Photonics, Japan) with an output power [P0(1 + cosωt)] and angular frequency (ω); the temperature response was then monitored at the bottom of the sample by the pyrometer.

2.3.1 Heat Capacity Measurements

The heat capacity of the V melts at constant pressures (CP) were measured through laser modulation calorimetry. The measurement requires the temperature amplitude (ΔTac) and the phase shift (Δϕ) between the irradiated laser power and temperature response. The three quantities are expressed as

$${C}_{P}=\frac{{\alpha }_{{\text{N}}}{S}_{h}A{P}_{0}}{\omega {\Delta T}_{{\text{ac}}}}f,$$
(5)
$$\Delta \phi ={\text{arccos}}\left\{\frac{{\tau }_{{\text{c}}}}{\omega }\left(\frac{1}{{\tau }_{{\text{c}}}{\tau }_{{\text{r}}}}-{\omega }^{2}\right)f\right\},$$
(6)
$$f={\left(1+\frac{1}{{\omega }^{2}{{\tau }_{{\text{r}}}}^{2}}+{\omega }^{2}{{\tau }_{{\text{c}}}}^{2}\right)}^{-\frac{1}{2}},$$
(7)

where αN denotes the laser absorptivity, Sh the area ratio of the laser-irradiated part of the levitated sample, A the surface area of the levitated sample, and f a correction function; τr and τc denote the external and internal thermal relaxation times. In this study, αN was assumed the same as ε based on Kirchhoff’s law. Because τc decreases with increasing convective flow in the melt, the quasi-adiabatic condition (i.e., τc/τr ≪ 1) is satisfied by a proper choice of laser-modulation frequency and static magnetic field. In CP measurements, a relatively low magnetic field intensity (2.2–2.5 T) was applied to levitate the sample and maintain convective flow. The relaxation times, τc and τr, are obtained by fitting Eq. 6 to the relation between Δϕ and the modulation frequency. After fitting, CP is determined using Eq. 6 with the values of τc, τr, and ΔTac.

2.3.2 Thermal Conductivity Measurements

Thermal conductivity (κ) was also measured through laser modulation calorimetry. The unsteady state heat conduction equation in spherical coordinates is expressed as

$$\rho {C}_{P}\frac{\partial T}{\partial t}=\kappa \left\{\frac{1}{{r}^{2}}\frac{\partial }{\partial r}\left({r}^{2}\frac{\partial T}{\partial r}\right)+\frac{1}{{r}^{2}{\text{sin}}\theta }\frac{\partial }{\partial \theta }\left({\text{sin}}\theta \frac{\partial T}{\partial \theta }\right)\right\}+Q\left(r, \theta \right),$$
(8)

where Q(r, θ) denotes the heat generated by induction current, t time, and r and θ denote the radial distance and polar angle, respectively, with the center of the sample as the origin. No azimuthal dependence for T and Q is assumed.

When the upper part of the droplet is irradiated by the modulated laser beam, the temperature at each point in the droplet (T(r, θ, t)) increases in average temperature and the modulation amplitude from the initial temperature and then reaches a stationary modulation state with a certain constant average temperature and amplitude. In this stationary modulation state, T(r, θ, t) is expressed as

$$T\left(r, \theta , t\right)={T}_{0}+\Delta {T}_{{\text{av}}}\left(r, \theta \right)+\Delta {T}_{{\text{ac}}}\left(r, \theta , t\right),$$
(9)

where T0 denotes the initial temperature of the droplet, ΔTav(r, θ) the increase in average temperature, and ΔTac takes the form

$$\Delta {T}_{{\text{ac}}}\left(r, \theta , t\right)=\Delta {{T}^{{\text{in}}}}_{{\text{ac}}}\left(r, \theta \right){\text{cos}}\omega t+\Delta {{T}^{{\text{out}}}}_{{\text{ac}}}\left(r, \theta \right){\text{sin}}\omega t,$$
(10)

where ΔTinac(r, θ) and ΔToutac(r, θ) denote the in-phase and out-of-phase components of ΔTac(r, θ, t). On substituting Eqs. 9 and 10 into Eq. 8, a steady-state system of linear equations for ΔTinac(r, θ) and ΔToutac(r, θ) are obtained

$$\rho {C}_{P}\omega \Delta {{T}^{{\text{out}}}}_{{\text{ac}}}=\kappa \left\{\frac{1}{{r}^{2}}\frac{\partial }{\partial r}\left({r}^{2}\frac{\partial (\Delta {{T}^{{\text{in}}}}_{{\text{ac}}})}{\partial r}\right)+\frac{1}{{r}^{2}{\text{sin}}\theta }\frac{\partial }{\partial \theta }\left({\text{sin}}\theta \frac{\partial (\Delta {{T}^{{\text{in}}}}_{{\text{ac}}})}{\partial \theta }\right)\right\},$$
(11)
$$\rho {C}_{P}\omega \left(-\Delta {{T}^{{\text{in}}}}_{{\text{ac}}}\right)=\kappa \left\{\frac{1}{{r}^{2}}\frac{\partial }{\partial r}\left({r}^{2}\frac{\partial \left(\Delta {{T}^{{\text{out}}}}_{{\text{ac}}}\right)}{\partial r}\right)+\frac{1}{{r}^{2}{\text{sin}}\theta }\frac{\partial }{\partial \theta }\left({\text{sin}}\theta \frac{\partial \left(\Delta {{T}^{{\text{out}}}}_{{\text{ac}}}\right)}{\partial \theta }\right)\right\}.$$
(12)

Equations 11 and 12 were solved using the finite element method with boundary conditions at the laser-irradiated and non-laser-irradiated droplet surfaces to obtain the ΔTinac(r, θ) and ΔToutac(r, θ) distributions in the droplet. Using the values obtained, the phase shift ∆ϕ is given by

$$\Delta \phi ={{\text{tan}}}^{-1}\left(\frac{\Delta {{T}^{{\text{out}}}}_{{\text{ac}}}}{\Delta {{T}^{{\text{in}}}}_{{\text{ac}}}}\right).$$
(13)

The unknown parameter, κ, is obtained by fitting the modulation frequency dependence of the phase shift obtained from the above analysis to the experimentally obtained phase shift using the least-squares method.

3 Results

3.1 Density

Figure 2 and Table 1 present results of the temperature dependence of the ρ of V melt with values obtained from the literature [26,27,28,29,30,31]. Our results show clear linearity over a range that includes the supercooled temperature region and are in good agreement with literature data reported by Saito et al. [26], Paradis et al. [27], Ivaschenko and Marcgenuk [29], Reiplinger and Brillo [31], and Zhang et al. [32]. The density ρ can be expressed as

$$\rho ={\rho }_{{\text{c}}}\left(T-{T}_{{\text{m}}}\right)+{\rho }_{{\text{m}}},$$
(14)

where ρc denotes the coefficient of proportionality between density and temperature, Tm the melting point, and ρm the density at the melting point. The error bar shows the expanded uncertainty (k = 2), which is described in Sect. 4.1.1.

Fig. 2
figure 2

Temperature dependence of density of vanadium melt. Black-dashed line marks the melting point (M.P.). Error bars show the expanded uncertainty (k = 2). PW:Present work (EML with a static magnetic field), [26]: Saito et al. (EML), [27]: Paradis et al. (ESL), [28]: Elyutin and Kostikov (Pendant drop), [29]: Ivaschenko and Marcgenuk (Pendant drop), [30]: Maurakh (Maximum bubble pressure), [31]: Reiplinger and Brillo (EML), [32]: Zhang et al. (ESL)

Full size image
Table 1 Densities of vanadium melts as functions of temperature
Full size table

In determining the volume from the side-view image taken in the pendant drop method, Elyutin et al. [28] assumed that the melt has a symmetrical shape, and gave an uncertainty in calculating the volume ranging from 3 % to 5 %. This uncertainty mainly occurs in the deformation of drops from the axial symmetry in the vertical direction. Because of the large uncertainty, the present work differs from the values reported by Elyutin et al. [28].

Maurakh [30] used a beryllium oxide crucible and capillary for density measurements, and there is concern that the vanadium melt sample was contaminated with oxygen or beryllium during the experiment.

3.2 Normal Spectral Emissivity

Figure 3 shows the temperature dependence of the normal spectral emissivity at 807 nm and 940 nm of V melt. The error bars show the expanded uncertainty, which is discussed in Sect. 4.1.2. The normal spectral emissivity of the V melt exhibits a negligible temperature dependence. Figure 4 and Table 2 show the wavelength dependence of the normal spectral emissivity at the melting point along with reported values [24, 33,34,35,36,37,38]. The calculated value obtained by the Drude model is also presented in Fig. 4 and is described in Sect. 4.2. The present results are in good agreement with the results reported by McClure and Cezairliyan [35]. The emissivity of V melts decreases with increasing wavelength.

Fig. 3
figure 3

Temperature dependence of normal spectral emissivity of vanadium melts at 807 and 940 nm. Black dashed line marks the melting point (M.P.). Error bars show the expanded uncertainty (k = 2)

Full size image
Fig. 4
figure 4

Wavelength dependence of normal spectral emissivity of vanadium at melting point. The dotted line shows the values obtained from the Drude model. Error bars show the expanded uncertainty (k = 2). PW: Present work, [24]: Pottlacher et al. (Pulse-heating with polarimeter), [33]: Lin and Frohberg. (Electrostatic levitation with spectrometer), [34]: Treverton and Margrave (EML with pyrometer), [35]: McClure and Cezairliyan (Pulse-heating with pyrometer), [36]: Cezairliyan et al. (Pulse-heating with pyrometer), [37]: Ronchi et al. (Pulsed laser with pyrometer), [38]: Berezin et al. (EML with pyrometer)

Full size image
Table 2 Normal spectral emissivity of vanadium melts
Full size table

3.3 Heat Capacity at Constant Pressure

As an example, the temperature response of the V melt to laser irradiation during the modulation calorimetry at a static magnetic field of 2.5 T and a modulation frequency of 0.08 Hz is shown in Fig. 5. Figure 6 plots Δϕ and ωΔTac of the melt as a function of the modulation frequency in a static magnetic field of 2.5 T at 2016 K. The τr and τc values were obtained by curve fitting using Eq. 6 to the phase shift to be 0.32 s and 5.59 s, respectively. The value for f is 0.95 at 0.12 Hz, where the quasi-adiabatic condition (ω2τr2 ≫ 1  ≫ ω2τc2, i.e., f≈1) is satisfied. The CP was determined using Eq. 6 employing the maximum value of ωΔTac. Figure 7 and Table 3 present the temperature dependence of CP for the V melt along with reported values [24, 27, 33, 34, 38, 39, 41,42,43,44,45,46,47,48,49]. The error bars indicate the expanded uncertainty (k = 2), which is described at Sect. 4.1.3. The temperature dependence of CP obtained in the present work was negligible with the range in temperature including the undercooled region. Within the expanded uncertainty, this result is in good agreement with almost all reported values except that of Hultgren et al. [46, 47], which did not describe details of the determination of recommended value of CP. In consequence, a discussion on differences between their work and ours is not possible.

Fig. 5
figure 5

Laser power (right-hand ordinate) and temperature response (left-hand ordinate) of vanadium melt during laser-modulated calorimetry with a modulation frequency at 0.08 Hz under static magnetic field of 2.5 T

Full size image
Fig. 6
figure 6

Phase shift (right-hand ordinate) and ωΔTac (left-hand ordinate) of vanadium melt as a function of modulation frequency at 2016 K under 2.5 T

Full size image
Fig. 7
figure 7

Temperature dependence of heat capacity at constant pressure of vanadium melt. Dashed black line marks the melting point (M.P.). Error bars show expanded uncertainty (k = 2). PW: Present work, [24]: Pottlacher et al. (Pulse-heating), [27]: Paradis et al. (Electrostatic levitation), [33]: Lin and Frohberg (EML with drop calorimetry), [34]: Treverton and Margrave (EML with drop calorimetry), [38] Berezin et al. (EML with drop calorimetry), [39]: Ishikawa et al. (Electrostatic levitation), [41]: Schaefers et al. (EML with drop calorimetry), [42]: Arblaster (Recommended value), [43]: NIST-JANAF (Recommended value), [44]: Berezin (EML with drop calorimetry), [45]:Desai (Recommended value), [46]:Hultgren et al. (Recommended value), [47]:Hultgren et al. (Recommended value), [48]: Dinsdale (Calculation), [49]: Sun et al. (Aerodynamic levitation)

Full size image
Table 3 Heat capacity at constant pressure of vanadium melts
Full size table

3.4 Thermal Conductivity

3.4.1 Effect of Static Magnetic Field on Apparent Thermal Conductivity

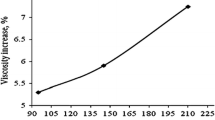
Because heat is carried by convective flows, the effect of applying a static magnetic field to suppress the convection in the κ measurement was investigated. Figure 8 shows the relationship between the phase shift (Δϕ) and the modulation frequency at 2167 K under a 10 T field. The value of κ was determined by reproducing the experimentally obtained relationships using Eq. 13. Figure 9 shows the static magnetic field dependence of the apparent κ of the V melt at 2183 K. The apparent κ of V melts decreased with increasing static magnetic field and converged above 7 T. This result indicates that convective flow in sample melts was sufficiently suppressed to enable thermal conductivity measurements above 7 T. From this result, thermal conductivity measurements were conducted under static magnetic fields of 10 T.

Fig. 8
figure 8

Phase shift of vanadium melt as a function of modulation frequency at 2167 K and 10 T

Full size image
Fig. 9
figure 9

Static magnetic field dependence of thermal conductivity of vanadium melts at 2183 K. The error bars show expanded uncertainty (k = 2)

Full size image

3.4.2 Thermal Conductivity and Thermal Diffusivity

Figure 10 and Table 4 show the temperature dependence of the thermal conductivity (κ) of the V melt measured in this study along with reported values [9, 24, 50, 51]. The present work shows κ increasing linearly with increasing temperature, even in the undercooled region. The error bars in Fig. 10 indicate the expanded uncertainty (k = 2), as described in Sect. 4.1.4. Within the experimental uncertainty, the present data of κ agrees with the values reported by Mills et al. [51].

Fig. 10
figure 10

Temperature dependence of thermal conductivity of vanadium melt. The dashed black line marks the melting point (M.P.). The error bars show expanded uncertainty (k = 2). PW: Present work, [9, 50]: Zinov’yev et al. (Plane temperature wave method), [24]: Pottlacher et al. (Wiedemann–Franz law), [51] Mills et al. (Recommended value)

Full size image
Table 4 Thermal conductivity of vanadium melt
Full size table

Figure 11 shows the temperature dependence of the thermal diffusivity (α), which was obtained using

Fig. 11
figure 11

Temperature dependence of thermal diffusivity of vanadium melt. The dashed black line marks the melting point (M.P.). The error bars show expanded uncertainty (k = 2). PW: Present work, [9, 50]: Zinov’yev et al. (Plane temperature wave method), [24]: Pottlacher et al. (Wiedemann–Franz law)

Full size image
$$\alpha =\frac{\kappa }{{C}_{P}\bullet \rho }.$$
(15)

Zinov’yev et al. [9, 50] measured α employing the plane temperature wave method; their value of α was higher than the present data. As described in Sect. 1, Assael et al. [10] reported a higher value of α than the true values obtained using the plane temperature wave method because of the circulational flow. In contrast, the value of κ reported by Zinov’yev et al. was lower than the present data but they had used the low CP value of Hultgren et al. [47].

The present work on κ and α gave higher values than the data reported in Pottlacher et al. [24], in which α and κ were determined using the Wiedemann–Franz law and the electric conductivity to be discussed in Sect. 4.3.

4 Discussion

4.1 Uncertainty Analysis

The uncertainty associated with measurements was evaluated based on the Guide to the Expression of Uncertainty in Measurement (GUM) [52]. In addition, the regression analysis was conducted to evaluate the experimental variance of the slope and intercept in the equations.

4.1.1 Uncertainty Analysis of the Density Measurement

The uncertainty in the density measurement was evaluated using

$${u}^{2}\left(\rho \right)={\left(\frac{\partial \rho }{\partial V}\right)}^{2}{u}^{2}\left(V\right)+{\left(\frac{\partial \rho }{\partial M}\right)}^{2}{u}^{2}\left(M\right),$$
(16)

where u(ρ) denotes the combined standard uncertainty in the density measurement, u(V) the uncertainty from the standard deviation of values of volume evaluated from 3000 droplet images, and u(M) the uncertainty of mass due to sample evaporation. By way of example, the uncertainty of density measurement at 2153 K is outlined in Table 5. The dominant component of the uncertainty is u(V), and the value of U = 2 u(ρ) was 105 kg·m−3, which corresponds to 1.9 % of the density value (5490 kg·m−3). For all density measurements, U is between 1.4 % and 1.9 %.

Table 5 Uncertainty evaluation in density measurements at 2153 K
Full size table

4.1.2 Uncertainty Analysis of Normal Spectral Emissivity Measurement

Similarly, the uncertainty of the normal spectral emissivity is expressed as

$${u}^{2}\left(\varepsilon \right)={\left(\frac{\partial \varepsilon }{\partial {{\text{C}}}_{0}}\right)}^{2}{u}^{2}\left({{\text{C}}}_{0}\right)+{\left(\frac{\partial \varepsilon }{\partial T}\right)}^{2}{u}^{2}\left({T}_{{\text{Pyro}}}\right)+{\left(\frac{\partial \varepsilon }{\partial T}\right)}^{2}{u}^{2}\left({T}_{{\text{Cal}}}\right),$$
(17)

where u(ε) denotes the combined standard uncertainty of the normal spectral emissivity; u(C0), u(TPyro), and u(TCal) denote respectively the standard uncertainty of the coefficient of C0, the accuracy of the pyrometer, and the uncertainty of the pyrometer calibration using the temperature profile at the melting point. Based on the pyrometer specifications, u(TPyro) was evaluated as 0.3 % of the measured value plus 1 K up to 1773 K and 0.5 % of the measured value above 1773 K.

As an example, the uncertainty evaluation of the emissivity at 940 nm for V melts at 2177 K is outlined in Table 6. The dominant contribution to u(ε) is u(TCal). The expanded uncertainty U = 2u(ε) is 0.020, which corresponds to 6.6 % of the emissivity. For the V melts overall, the expanded uncertainty varies from 7.6 % to 7.7 % at 807 nm, and from 6.5 % to 6.6 % at 940 nm, respectively.

Table 6 Uncertainty evaluation in emissivity measurement at 940 nm at 2177 K
Full size table

4.1.3 Uncertainty Analysis of Heat Capacity Measurement

The uncertainty of the heat capacity is given by

$${u}^{2}\left({C}_{P}\right)={\left(\frac{\partial {C}_{P}}{\partial \varepsilon }\right)}^{2}{u}^{2}\left(\varepsilon \right)+{\left(\frac{\partial \varepsilon }{\partial \Delta {T}_{{\text{ac}}}}\right)}^{2}{u}^{2}\left(\Delta {T}_{{\text{ac}}}\right)+{\left(\frac{\partial {C}_{P}}{\partial m}\right)}^{2}{u}^{2}\left(m\right),$$
(18)

where u(CP) denotes the combined standard uncertainty of the heat capacity; u(ε), u(ΔTac), and u(m) denote respectively the uncertainties of the normal spectral emissivity, temperature amplitude, and sample mass. As an example, Table 7 outlines the evaluation of the uncertainty in heat capacity measurements of the V melt at 2137 K. The factor u(ε) contributes strongly to u(CP) with an expanded uncertainty U = 2u(CP) of 3.18 J·mol−1·K−1, which corresponds to 6.8 % of the heat capacity. For the V melts overall, the expanded uncertainty for all measurements ranged from 6.6 % to 6.8 %, respectively.

Table 7 Uncertainty evaluation in heat capacity at constant pressure at 2137 K
Full size table

4.1.4 Uncertainty Analysis of Thermal Conductivity Measurement

The uncertainty of the thermal conductivity was evaluated by the following equation:

$${u(\kappa )}^{2}={\left(\frac{\partial \kappa }{\partial \Delta \phi }\right)}^{2}{u}^{2}\left(\Delta \phi \right)+{\left(\frac{\partial \kappa }{\partial \rho }\right)}^{2}{u}^{2}\left(\rho \right)+{\left(\frac{\partial \kappa }{\partial {C}_{P}}\right)}^{2}{u}^{2}\left({C}_{P}\right),$$
(19)

where u(κ) denotes the combined standard uncertainty of the thermal conductivity, and u(Δϕ) the uncertainty of the phase shift, which was evaluated as the standard deviation of the phase shift at 0.5 Hz corresponding to the highest frequency. As an example, Table 8 lists the uncertainty contributions in the thermal conductivity measurement u(κ) of the V melt at 2215 K. The dominant contribution is u(CP) with an expanded uncertainty U = 2u(κ) of 3.30 W·m−1·K−1, which corresponds to 7.1 % of the thermal conductivity. The expanded uncertainty for all measurements of the V melts ranged from 6.9 % to 7.1 %, respectively.

Table 8 Uncertainty evaluation in thermal conductivity measurement at 2215 K
Full size table

4.1.5 Uncertainty analysis of Thermal Diffusivity

$${u(\alpha )}^{2}={\left(\frac{\partial \alpha }{\partial \kappa }\right)}^{2}{u}^{2}\left(\kappa \right)+{\left(\frac{\partial \alpha }{\partial \rho }\right)}^{2}{u}^{2}\left(\rho \right)+{\left(\frac{\partial \alpha }{\partial {C}_{P}}\right)}^{2}{u}^{2}\left({C}_{P}\right),$$
(20)

where u(α) denotes the combined standard uncertainty of the thermal diffusivity. As an example, Table 9 lists the uncertainty contributions in the thermal conductivity u(α) of the V melt at 2215 K. The dominant contribution is u(κ) with an expanded uncertainty U = 2u(α) of 9.08 × 10−7 m2·s−1, which corresponds to 9.9 % of the thermal diffusivity. The expanded uncertainty for all measurements of the V melts ranged from 9.7 % to 9.9 %, respectively.

Table 9 Uncertainty evaluation in thermal diffusivity at 2215 K
Full size table

4.2 Drude Model

As indicated by the dashed line in Fig. 4, ε was calculated using the Drude model. The Drude model had been previously described in Ref. [22] and is only outlined here. The values used in this study are listed in Table 10. Given the number of valence electrons, the number of free electrons per atom reported by Itami and Shimoji [53] was used. Susa’s group [54, 55] described the spectral radiation mechanism, which involves of the excitation-relaxation of the free electrons and the inner shell electrons; the former being called an interband transition and the latter called an intraband transition. For transition metals and noble metals, the interband transition mainly occurs through the excitation of electrons from the d band to the conduction band above Fermi level, affecting ε in the visible region. In the Drude model, the intraband transition can be interpreted but the influence on the interband transition is not considered. As the wavelength decreases, the difference between the calculated and experimental emissivity increases. This indicates the effect of interband transition of electrons.

Table 10 Values used to calculate normal spectral emissivity of V melts by the Drude model at melting point
Full size table

4.3 Wiedemann–Franz Law

The thermal conductivity (κ) is expressed as

$$\kappa ={\kappa }_{{\text{vib}}}+{\kappa }_{{\text{el}}},$$
(21)

where κvib and κel denote the atomic thermal vibration part and the free electron contribution part in the thermal conductivity, respectively. According to the Wiedemann–Franz law, κel depends on the electrical resistivity (ρel) and Lorenz number (L = 2.45 × 10−8/W·Ω·K−2),

$${\kappa }_{{\text{el}}}=LT{{\rho }_{{\text{el}}}}^{-1}.$$
(22)

From Figs. 10 and 11, the value obtained was larger than that reported by Pottlacher et al. [24] which were calculated using the Wiedemann–Franz law. The difference may have risen from the contribution of the thermal vibration of the atoms to the thermal conductivity of the V melt.

4.4 Correlation Between Density of States and Thermal Conductivity

In 1936, Mott [6, 7] reported the relationship between electrical current and the DOS at the Fermi level (N(EF)) of the transition metals; specifically.

  • 1. The electrical current is carried by almost all the s electrons, each having an effective mass of approximately that of a free electron.

  • 2. The electrical resistivity is mainly attributed to the s electron transition to the d state (i.e., s–d transition), and the conduction of the electrons in the d state is relatively smaller because of the heavier effective mass.

  • 3. The probability of an s–d transition is proportional to the DOS in the d state.

These observations constitute Mott’s s–d scattering model.

Based on the scattering model, our group found a linear correlation between the reciprocal DOS at the Fermi level (N(EF))−1 and α for six transition metals in liquid states [23].

With the additional V melt data, we were able to discuss further the correlation between (N(EF))−1 and thermophysical properties. Figure 12a plots (N(EF))−1 for liquid states of seven transition metals [23, 56]. Similarly, Fig. 12b plots the thermal conductivity (left vertical axis) and thermal diffusivity (right vertical axis) for these same transition metals.

Fig. 12
figure 12

(a) Reciprocal density of states at the Fermi level of transition metals in the liquid state [23, 56], (b) Thermal conductivity (left vertical axis) and thermal diffusivity (right vertical axis) of transition metals in the liquid state. (c) Correlation between the reciprocal density of states at the Fermi level and thermal diffusivity of transition metals. All error bars indicate expanded uncertainty (k = 2)

Full size image

For ease of comparison, their values of (N(EF))−1, α, and κ are listed in Table 11. The value in Table 11 represent data at a temperature ranging from – 25 K to 50 K from the melting point of respective metals. Figure 12a and b shows the same trend in elemental dependence for (N(EF))−1 and α. Figure 12c shows for these transition metals near linearity in the dependence of (N(EF))−1 on α with one exception the Pd melt, which deviates from the trend because of a large contribution from thermal vibration of the atoms [23]. This result from V melts indicates that heat conduction in transition metals can be roughly explained with Mott’s s–d scattering model, in which the s-electrons mainly carry the heat.

Table 11 Reciprocal density of states at the Fermi level, thermal diffusivity, and thermal conductivity of transition metals in the liquid state
Full size table

5 Conclusion

In this study, density, normal spectral emissivity between 807 nm and 940 nm, heat capacity at constant pressure, and thermal conductivity of the V melt were measured using the PROSPECT facility. The value obtained for the normal spectral emissivity of the V melt was larger than that calculated by the Drude model. This indicates that the effect of interband transitions of d-state electrons increases with decreasing wavelength. Likewise, the value obtained for the thermal conductivity was larger than that obtained from the Wiedemann–Franz law. The difference between the two reflects the contribution to thermal conduction from the thermal vibration of atoms in the melt. The thermal diffusivity of the V melt was determined from the density, heat capacity, and thermal conductivity. The value obtained is approximately proportional to the reciprocal DOS at the Fermi level, and likewise for other transition metals. Except for the Pd melt, the thermal diffusivities of the transition elements in the liquid state can be explained by Mott’s s–d scattering model and the heat of the transition elements is transported by s-electrons.