Skip to main content
SpringerLink
Log in

A structure-preserving parametric finite element method for geometric flows with anisotropic surface energy

  • Published:
Numerische Mathematik Aims and scope Submit manuscript

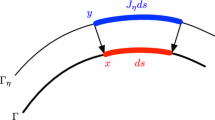
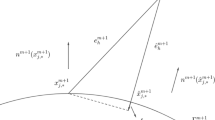
Abstract

We propose and analyze a structure-preserving parametric finite element method (SP-PFEM) for the evolution of a closed curve under different geometric flows with arbitrary anisotropic surface energy density \(\gamma (\varvec{n})\), where \(\varvec{n}\in \mathbb {S}^1\) represents the outward unit normal vector. We begin with the anisotropic surface diffusion which possesses two well-known geometric structures—area conservation and energy dissipation—during the evolution of the closed curve. By introducing a novel surface energy matrix \(\varvec{G}_k(\varvec{n})\) depending on \(\gamma (\varvec{n})\) and the Cahn-Hoffman \(\varvec{\xi }\)-vector as well as a nonnegative stabilizing function \(k(\varvec{n})\), we obtain a new conservative geometric partial differential equation and its corresponding variational formulation for the anisotropic surface diffusion. Based on the new weak formulation, we propose a full discretization by adopting the parametric finite element method for spatial discretization and a semi-implicit temporal discretization with a proper and clever approximation for the outward normal vector. Under a mild and natural condition on \(\gamma (\varvec{n})\), we can prove that the proposed full discretization is structure-preserving, i.e. it preserves the area conservation and energy dissipation at the discretized level, and thus it is unconditionally energy stable. The proposed SP-PFEM is then extended to simulate the evolution of a close curve under other anisotropic geometric flows including anisotropic curvature flow and area-conserved anisotropic curvature flow. Extensive numerical results are reported to demonstrate the efficiency and unconditional energy stability as well as good mesh quality (and thus no need to re-mesh during the evolution) of the proposed SP-PFEM for simulating anisotropic geometric flows.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9

Similar content being viewed by others

References

  1. Andrews, B.: Volume-preserving anisotropic mean curvature flow. Indiana Univ. Math. J., 783-827 (2001)

  2. Bao, W., Garcke, H., Nürnberg, R., Zhao, Q.: Volume-preserving parametric finite element methods for axisymmetric geometric evolution equations. J. Comput. Phys. 460, 111180 (2022)

    Article  MathSciNet  Google Scholar 

  3. Bao, W., Garcke, H., Nürnberg, R., Zhao, Q.: A structure-preserving finite element approximation of surface diffusion for curve networks and surface clusters. Numer. Methods Partial Differ. Equ. 39, 759–794 (2023)

    Article  MathSciNet  Google Scholar 

  4. Bao, W., Jiang, W., Li, Y.: A symmetrized parametric finite element method for anisotropic surface diffusion of closed curves. SIAM J. Numer. Anal. 61(2), 617–641 (2023)

    Article  MathSciNet  Google Scholar 

  5. Bao, W., Jiang, W., Wang, Y., Zhao, Q.: A parametric finite element method for solid-state dewetting problems with anisotropic surface energies. J. Comput. Phys. 330, 380–400 (2017)

    Article  MathSciNet  Google Scholar 

  6. Bao, W., Li, Y.: A symmetrized parametric finite element method for anisotropic surface diffusion in three dimensions. SIAM J. Sci. Comput. 45(4), A1438–A1461 (2023)

    Article  MathSciNet  Google Scholar 

  7. Bao, W., Zhao, Q.: An energy-stable parametric finite element method for simulating solid-state dewetting problems in three dimensions. J. Comput. Math. 41, 771–796 (2023)

    Article  MathSciNet  Google Scholar 

  8. Bao, W., Zhao, Q.: A structure-preserving parametric finite element method for surface diffusion. SIAM J. Numer. Anal. 59(5), 2775–2799 (2021)

    Article  MathSciNet  Google Scholar 

  9. Barrett, J.W., Garcke, H., Nürnberg, R.: A parametric finite element method for fourth order geometric evolution equations. J. Comput. Phys. 222(1), 441–467 (2007)

    Article  MathSciNet  Google Scholar 

  10. Barrett, J.W., Garcke, H., Nürnberg, R.: Numerical approximation of anisotropic geometric evolution equations in the plane. IMA J. Numer. Anal. 28(2), 292–330 (2008)

    Article  MathSciNet  Google Scholar 

  11. Barrett, J.W., Garcke, H., Nürnberg, R.: On the parametric finite element approximation of evolving hypersurfaces in \(\mathbb{R} ^3\). J. Comput. Phys. 227(9), 4281–4307 (2008)

    Article  MathSciNet  Google Scholar 

  12. Barrett, J.W., Garcke, H., Nürnberg, R.: A variational formulation of anisotropic geometric evolution equations in higher dimensions. Numer. Math. 109(1), 1–44 (2008)

    Article  MathSciNet  Google Scholar 

  13. Barrett, J.W., Garcke, H., Nürnberg, R.: Finite-element approximation of coupled surface and grain boundary motion with applications to thermal grooving and sintering. Eur. J. Appl. Math. 21(6), 519–556 (2010)

    Article  MathSciNet  Google Scholar 

  14. Barrett, J.W., Garcke, H., Nürnberg, R.: Parametric finite element approximations of curvature-driven interface evolutions. Handb. Numer. Anal. 21, 275–423 (2020)

    MathSciNet  Google Scholar 

  15. Cahn, J.: Stability, microstructural evolution, grain growth, and coarsening in a two-dimensional two-phase microstructure. Acta Metall. Mater. 39(10), 2189–2199 (1991)

    Article  Google Scholar 

  16. Cahn, J.W., Taylor, J.E.: Overview no. 113 surface motion by surface diffusion. Acta Metall. Mater. 42(4), 1045–1063 (1994)

    Article  Google Scholar 

  17. Carter, W.C., Roosen, A., Cahn, J.W., Taylor, J.E.: Shape evolution by surface diffusion and surface attachment limited kinetics on completely faceted surfaces. Acta Metall. Mater. 43(12), 4309–4323 (1995)

    Article  Google Scholar 

  18. Chen, Y., Giga, Y., Goto, S.: Uniqueness and existence of viscosity solutions of generalized mean curvature flow equations. In: Fundamental Contributions to the Continuum Theory of Evolving Phase Interfaces in Solids, pp. 375–412. Springer, Berlin (1999)

    Chapter  Google Scholar 

  19. Clarenz, U., Diewald, U.,Rumpf, M.: Anisotropic geometric diffusion in surface processing. IEEE Vis. (2000)

  20. Deckelnick, K., Dziuk, G., Elliott, C.M.: Computation of geometric partial differential equations and mean curvature flow. Acta Numer. 14, 139–232 (2005)

    Article  MathSciNet  Google Scholar 

  21. Deckelnick, K., Dziuk, G., Elliott, C.M.: Fully discrete finite element approximation for anisotropic surface diffusion of graphs. SIAM J. Numer. Anal. 43(3), 1112–1138 (2005)

    Article  MathSciNet  Google Scholar 

  22. Dolcetta, I.C., Vita, S.F., March, R.: Area-preserving curve-shortening flows: from phase separation to image processing. Interfaces Free Bound. 4(4), 325–343 (2002)

    Article  MathSciNet  Google Scholar 

  23. Du, P., Khenner, M., Wong, H.: A tangent-plane marker-particle method for the computation of three-dimensional solid surfaces evolving by surface diffusion on a substrate. J. Comput. Phys. 229(3), 813–827 (2010)

    Article  MathSciNet  Google Scholar 

  24. Du, Q., Feng, X.: The phase field method for geometric moving interfaces and their numerical approximations. Handb. Numer. Anal 21, 425–508 (2020)

    MathSciNet  Google Scholar 

  25. Dziuk, G.: An algorithm for evolutionary surfaces. Numer. Math. 58(1), 603–611 (1990)

    Article  MathSciNet  Google Scholar 

  26. Dziuk, G.: Convergence of a semi-discrete scheme for the curve shortening flow. Math. Models Methods Appl. Sci. 04(4), 589–606 (1994)

    Article  MathSciNet  Google Scholar 

  27. Fonseca, I., Pratelli, A., Zwicknagl, B.: Shapes of epitaxially grown quantum dots. Arch. Ration. Mech. Anal. 214, 359–401 (2014)

    Article  MathSciNet  Google Scholar 

  28. Girao, P.M., Kohn, R.V.: The crystalline algorithm for computing motion by curvature. In: Variational Methods for Discontinuous Structures, pp. 7–18. Springer, Berlin (1996)

    Chapter  Google Scholar 

  29. Gurtin, M.E., Jabbour, M.E.: Interface evolution in three dimensions with curvature-dependent energy and surface diffusion: interface-controlled evolution, phase transitions, epitaxial growth of elastic films. Arch. Ration. Mech. Anal. 163, 171–208 (2002)

    Article  MathSciNet  Google Scholar 

  30. Hoffman, D.W., Cahn, J.W.: A vector thermodynamics for anisotropic surfaces: I. Fundamentals and application to plane surface junctions. Surf. Sci. 31, 368–388 (1972)

    Article  Google Scholar 

  31. Jiang, W., Bao, W., Thompson, C.V., Srolovitz, D.J.: Phase field approach for simulating solid-state dewetting problems. Acta Mater. 60(15), 5578–5592 (2012)

    Article  Google Scholar 

  32. Jiang, W., Wang, Y., Zhao, Q., Srolovitz, D.J., Bao, W.: Solid-state dewetting and island morphologies in strongly anisotropic materials. Scr. Mater. 115, 123–127 (2016)

    Article  Google Scholar 

  33. Jiang, W., Zhao, Q.: Sharp-interface approach for simulating solid-state dewetting in two dimensions: A Cahn-Hoffman \(\varvec {\xi }\)-vector formulation. Phys. D 390, 69–83 (2019)

    Article  MathSciNet  Google Scholar 

  34. Kovács, B., Li, B., Lubich, C.: A convergent evolving finite element algorithm for mean curvature flow of closed surfaces. Numer. Math. 143, 797–853 (2019)

    Article  MathSciNet  Google Scholar 

  35. Li, Y., Bao, W.: An energy-stable parametric finite element method for anisotropic surface diffusion. J. Comput. Phys. 446, 110658 (2021)

    Article  MathSciNet  Google Scholar 

  36. Mercier, G., Novaga, M., Pozzi, P.: Anisotropic curvature flow of immersed curves. Commun. Anal. Geom. 27(4), 937–964 (2019)

    Article  MathSciNet  Google Scholar 

  37. Niessen, W.J., Romeny, B.M., Florack, L.M., Viergever, M.A.: A general framework for geometry-driven evolution equations. Int. J. Comput. Vis. 21(3), 187–205 (1997)

    Article  Google Scholar 

  38. Perl, R., Pozzi, P., Rumpf, M.: A Nested Variational Time Discretization for Parametric Anisotropic Willmore Flow, pp. 221–241. Springer International Publishing, Berlin (2014)

    Google Scholar 

  39. Randolph, S., Fowlkes, J., Melechko, A., Klein, K., Meyer, I., Simpson, M., Rack, P.: Controlling thin film structure for the dewetting of catalyst nanoparticle arrays for subsequent carbon nanofiber growth. Nanotech. 18(46), 465354 (2007)

    Article  Google Scholar 

  40. Shen, H., Nutt, S., Hull, D.: Direct observation and measurement of fiber architecture in short fiber-polymer composite foam through micro-CT imaging. Compos. Sci. Technol. 64(13–14), 2113–2120 (2004)

    Article  Google Scholar 

  41. Sutton, A.P., Balluffi, R.W.: Interfaces in Crystalline Materials. Clarendon Press, Oxford (1995)

    Google Scholar 

  42. Taylor, J.E.: Mean curvature and weighted mean curvature. Acta Metall. Mater. 40, 1475–1485 (1992)

    Article  Google Scholar 

  43. Taylor, J.E., Cahn, J.W., Handwerker, C.A.: Overview no. 98 i–geometric models of crystal growth. Acta Metall. Mater. 40(7), 1443–1474 (1992)

    Article  Google Scholar 

  44. Thompson, C.V.: Solid-state dewetting of thin films. Annu. Rev. Mater. Res. 42, 399–434 (2012)

    Article  Google Scholar 

  45. Wang, Y., Jiang, W., Bao, W., Srolovitz, D.J.: Sharp interface model for solid-state dewetting problems with weakly anisotropic surface energies. Phys. Rev. B 91(4), 045303 (2015)

    Article  Google Scholar 

  46. Wheeler, A.: Cahn-Hoffman \(\varvec {\xi }\)-vector and its relation to diffuse interface models of phase transitions. J. Stat. Phys. 95, 1245–1280 (1999)

    Article  MathSciNet  Google Scholar 

  47. Wong, H., Voorhees, P., Miksis, M., Davis, S.: Periodic mass shedding of a retracting solid film step. Acta Mater. 48(8), 1719–1728 (2000)

    Article  Google Scholar 

  48. Xu, Y., Shu, C.: Local discontinuous Galerkin method for surface diffusion and Willmore flow of graphs. J. Sci. Comput. 40(1–3), 375–390 (2009)

    Article  MathSciNet  Google Scholar 

  49. Ye, J., Thompson, C.V.: Mechanisms of complex morphological evolution during solid-state dewetting of single-crystal nickel thin films. Appl. Phys. Lett. 97(7), 071904 (2010)

    Article  Google Scholar 

  50. Zhao, Q., Jiang, W., Bao, W.: An energy-stable parametric finite element method for simulating solid-state dewetting. IMA J. Numer. Anal. 41(3), 2026–2055 (2021)

    Article  MathSciNet  Google Scholar 

Download references

Acknowledgements

This work was partially supported by the Ministry of Education of Singapore under its AcRF Tier 2 funding MOE-T2EP20122-0002 (A-8000962-00-00). Part of the work was done when the authors were visiting the Institute of Mathematical Science at the National University of Singapore in 2023.

Author information

Authors and Affiliations

  1. Department of Mathematics, National University of Singapore, Singapore, 119076, Singapore

    Weizhu Bao & Yifei Li

Authors
  1. Weizhu Bao
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Yifei Li
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Yifei Li.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Bao, W., Li, Y. A structure-preserving parametric finite element method for geometric flows with anisotropic surface energy. Numer. Math. 156, 609–639 (2024). https://doi.org/10.1007/s00211-024-01398-8

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00211-024-01398-8

Mathematics Subject Classification

Search

Navigation