Dephasing effects on nonclassical correlations in two-qubit Heisenberg spin chain model with anisotropic spin–orbit interactions

Abstract

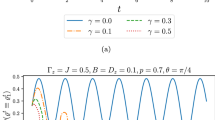
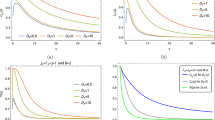
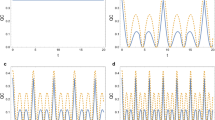
The advancement of quantum information hinges on the scalability of quantum systems to maintain non-local characteristics and improve the efficiency of quantum protocols. Strategies to enhance nonclassical correlations within quantum systems while mitigating decoherence phenomena are searing topics nowadays. Our study delves into the dynamics of nonclassical correlations within a two-qubit Heisenberg spin XXX model, utilizing Milburn’s dynamical master equation. We consider the impacts of anisotropic spin–orbit interactions, such as the Dzyaloshinskii–Moriya (DM) interaction and Kaplan–Shekhtman–Entin–Wohlman–Aharony (KSEWA) interaction, and employ three metrics, namely Bell’s inequality violation, concurrence, and local quantum uncertainty, to examine the dynamical features of nonclassical correlations under intrinsic decoherence. Our findings showcase the significance of the initial state of the two-qubit system in determining the parameters of spin–orbit interactions that influence the nonclassicality of the system. This underscores the critical role that DM and KSEWA interactions play in nonclassical correlations in solid-state quantum systems.

Appendices

Appendices

1.1 Appendix A: Bell non-locality

Consider a two-qubit X-shape density matrix:

$$\begin{aligned} \hat{X}^{AB}=\left( \begin{array}{cccc} \rho _{11} &{} 0 &{} 0 &{} \rho _{14} \\ 0 &{} \rho _{22} &{} \rho _{23} &{} 0 \\ 0 &{} \rho _{23}^* &{} \rho _{33} &{} 0 \\ \rho _{14}^* &{} 0 &{} 0 &{} \rho _{44} \\ \end{array} \right) , \end{aligned}$$

(21)

where the elements $\rho _{ij} \ge 0 (i,j=1,2,3,4)$ fulfill the unit trace (i.e., $\sum _{i=1}^4 \rho _{ii}=1$) and positivity conditions ($\rho _{22}\rho _{33} \ge \vert \rho _{23}\vert ^2$ and $\rho _{11}\rho _{44} \ge \vert \rho _{14}\vert ^2$). The nonzero elements $a_{ij}$ of the correlation matrix A (Eq. (14)) are given by:

$$\begin{aligned} \begin{aligned}&{a}_{11}=2\mathcal {R}(\varrho _{14}+\varrho _{23}),\\&{a}_{12}=-\mathcal {J}(\varrho _{14}-\varrho _{23}),\\&{a}_{21}=-\mathcal {J}(\varrho _{14}+\varrho _{23}),\\&{a}_{22}=-\mathcal {R}(\varrho _{14}-\varrho _{23}),\\&{a}_{33}=\varrho _{11}-\varrho _{22}-\varrho _{33}+\varrho _{44}. \end{aligned} \end{aligned}$$

(22)

where $\mathcal {R}(\Omega )$ and $\mathcal {J}(\Omega )$ denote, respectively, the real and imaginary parts of a given complex number $\Omega$. So, by making use of the above nonzero elements $a_{ij}$, we straightforwardly obtain the eigenvalues of the matrix $\mathcal {A}$ as:

$$\begin{aligned} \begin{aligned}&{\xi }_1=\left( \varrho _{11}-\varrho _{22}-\varrho _{33}+\varrho _{44}\right) {}^2,\\&{\xi }_2=4 \left( |\varrho _{23}|+|\varrho _{14}|\right) {}^2,\\&{\xi }_3=4 \left( |\varrho _{23}|-|\varrho _{14}|\right) {}^2. \end{aligned} \end{aligned}$$

(23)

So, the explicit formula of Bell non-locality is:

$$\begin{aligned} BL=2\sqrt{4 \left( |\varrho _{23}|+|\varrho _{14}|\right) {}^2+\max \left[ 4 \left( |\varrho _{23}|-|\varrho _{14}|\right) {}^2,\left( \varrho _{11}-\varrho _{22}-\varrho _{33}+\varrho _{44}\right) {}^2\right] }-1. \end{aligned}$$

(24)

1.2 Appendix B: Concurrence

The analytical expression of the concurrence for a two-qubit X-shape state (21) is obtained by making use of the following matrix:

$$\begin{aligned} \begin{aligned} M=&\hat{{X}}^{AB} (\hat{\sigma }_y^A\otimes \hat{\sigma }_y^B) \hat{ {X}}^{*AB} (\hat{\sigma }_y^A\otimes \hat{\sigma }_y^B)\\ =&\left( \begin{array}{cccc} |\varrho _{14}|^2+\varrho _{11} \varrho _{44} &{} 0 &{} 0 &{} 2 \varrho _{11} \varrho _{14} \\ 0 &{} |\varrho _{23}|^2+\varrho _{22} \varrho _{33} &{} 2 \varrho _{22} \varrho _{23} &{} 0 \\ 0 &{} 2 \varrho _{33} \varrho _{23}^* &{} |\varrho _{23}|^2+\varrho _{22} \varrho _{33} &{} 0 \\ 2 \varrho _{44} \varrho _{14}^*&{} 0 &{} 0 &{} |\varrho _{14}|^2+\varrho _{11} \varrho _{44} \\ \end{array} \right) . \end{aligned} \end{aligned}$$

(25)

Hence, the expression of the concurrence is given as:

$$\begin{aligned} C=2 \max [0,|\varrho _{23}|-\sqrt{\varrho _{11}\varrho _{44}},|\varrho _{14}|-\sqrt{\varrho _{22}\varrho _{33}}]. \end{aligned}$$

(26)

1.3 Appendix C: Local quantum uncertainty

The square root of a given two-qubit X-shape density matrix (Eq. (21)) is given by:

$$\begin{aligned} \sqrt{\hat{X}^{AB}}=\left( \begin{array}{cccc} b_{11} &{} 0 &{} 0 &{} b_{14} \\ 0 &{} b_{22} &{} b _{23} &{} 0 \\ 0 &{} b_{32} &{} b_{33} &{} 0 \\ b_{41} &{} 0 &{} 0 &{} b_{44} \\ \end{array} \right) , \end{aligned}$$

(27)

where

$$\begin{aligned} \begin{aligned}&b_{11}=\\&\frac{1}{2\sqrt{2} \sqrt{\left( \varrho _{11}-\varrho _{44}\right) ^2+4 |\varrho _{14}|^2}}\\&\quad \bigg [\left( \varrho _{11}-\varrho _{44}+\sqrt{\left( \varrho _{11}-\varrho _{44}\right) ^2+4 |\varrho _{14}|^2}\right) \sqrt{\varrho _{11}+\varrho _{44}+\sqrt{\left( \varrho _{11}-\varrho _{44}\right) ^2+4 |\varrho _{14}|^2}}\\&- \left( \varrho _{11}-\varrho _{44}-\sqrt{\left( \varrho _{11}-\varrho _{44}\right) ^2+4 |\varrho _{14}|^2}\right) \sqrt{\varrho _{11}+\varrho _{44}-\sqrt{\left( \varrho _{11}-\varrho _{44}\right) ^2+4 |\varrho _{14}|^2}}\bigg ]\\&b_{14}=b_{41}^{*}=\frac{\varrho _{14} \left( \sqrt{\varrho _{11}+\varrho _{44}+\sqrt{\left( \varrho _{11}-\varrho _{44}\right) ^2+4|\varrho _{14}|^2}}-\sqrt{\varrho _{11}+\varrho _{44}-\sqrt{\left( \varrho _{11}-\varrho _{44}\right) ^2+4 |\varrho _{14}|^2}}\right) }{\sqrt{2} \sqrt{\left( \varrho _{11}-\varrho _{44}\right) ^2+4 |\varrho _{14}|^2}}\\&b_{22}=\\&\frac{1}{2 \sqrt{2} \sqrt{\left( \varrho _{22}-\varrho _{33}\right) ^2+4 |\varrho _{23}|^2}}\\&\quad \bigg [\left( \varrho _{22}-\varrho _{33}+\sqrt{\left( \varrho _{22}-\varrho _{33}\right) ^2+4 |\varrho _{23}|^2}\right) \sqrt{\varrho _{22}+\varrho _{33}+\sqrt{\left( \varrho _{22}-\varrho _{33}\right) ^2+4 |\varrho _{23}|^2}}\\&-\left( \varrho _{22}-\varrho _{33}-\sqrt{\left( \varrho _{22}-\varrho _{33}\right) ^2+4 |\varrho _{23}|^2}\right) \sqrt{\varrho _{22}+\varrho _{33}-\sqrt{\left( \varrho _{22}-\varrho _{33}\right) ^2+4|\varrho _{23}|^2}}\bigg ]\\&b_{23}=b_{32}^{*}=\frac{\varrho _{23} \left( \sqrt{\varrho _{22}+\varrho _{33}+\sqrt{\left( \varrho _{22}-\varrho _{33}\right) ^2+4|\varrho _{23}|^2}}-\sqrt{\varrho _{22}+\varrho _{33}-\sqrt{\left( \varrho _{22}-\varrho _{33}\right) ^2+4 |\varrho _{23}|^2}}\right) }{\sqrt{2} \sqrt{\left( \varrho _{22}-\varrho _{33}\right) ^2+4 |\varrho _{23}|^2}}\\&b_{33}=\\&\frac{1}{2 \sqrt{2} \sqrt{\left( \varrho _{22}-\varrho _{33}\right) ^2+4 |\varrho _{23}|^2}}\\&\quad \bigg [\left( \varrho _{22}-\varrho _{33}+\sqrt{\left( \varrho _{22}-\varrho _{33}\right) ^2+4 |\varrho _{23}|^2}\right) \sqrt{\varrho _{22}+\varrho _{33}-\sqrt{\left( \varrho _{22}-\varrho _{33}\right) ^2+4 |\varrho _{23}|^2}}\\&-\left( \varrho _{22}-\varrho _{33}-\sqrt{\left( \varrho _{22}-\varrho _{33}\right) ^2+4 |\varrho _{23}|^2}\right) \sqrt{\varrho _{22}+\varrho _{33}+\sqrt{\left( \varrho _{22}-\varrho _{33}\right) ^2+4|\varrho _{23}|^2}}\bigg ]\\&b_{44}=\frac{1}{2\sqrt{2}\sqrt{\left( \varrho _{11}-\varrho _{44}\right) ^2 +4 |\varrho _{14}|^2}} \\&\quad \bigg [\left( \varrho _{11}-\varrho _{44}-\sqrt{\left( \varrho _{11}-\varrho _{44}\right) ^2+4 |\varrho _{14}|^2}\right) \sqrt{\varrho _{11}+\varrho _{44}+\sqrt{\left( \varrho _{11}-\varrho _{44}\right) ^2+4 |\varrho _{14}|^2}}\\&- \left( \varrho _{11}-\varrho _{44}-\sqrt{\left( \varrho _{11} -\varrho _{44}\right) ^2+4 |\varrho _{14}|^2}\right) \sqrt{\varrho _{11} +\varrho _{44}+\sqrt{\left( \varrho _{11}-\varrho _{44}\right) ^2+4 |\varrho _{14} |^2}}\bigg ].\\ \end{aligned} \end{aligned}$$

Then, by working out Eq. (20), we can get the correlation matrix W (3 $\times$ 3) as the following:

$$\begin{aligned} \begin{aligned}&W=\\&\left( \begin{array}{ccc} 2 \left( b_{11} b_{33}+b_{22} b_{44}+2\mathcal {R}(b_{14} b_{23})\right) &{} 4 i b_{14} \mathcal {I}(b_{23}) &{} 0 \\ 4 i b_{14}^* \mathcal {I}(b_{23}) &{} 2 \left( b_{11} b_{33}+b_{22} b_{44}-2\mathcal {R}(b_{14} b_{23})\right) &{} 0 \\ 0 &{} 0 &{} b_{11}^2+b_{22}^2+b_{33}^2+b_{44}^2-2 (|b_{23}|^2+|b_{14}|^2) \\ \end{array} \right) , \end{aligned} \end{aligned}$$

(28)

where its eigenvalues are given by:

$$\begin{aligned} \begin{aligned}&\mu _1=2 \left( b_{11} b_{33}+b_{22} b_{44}-\sqrt{(b_{14} b_{23})^2+(b_{23}^* b_{14}^*)^2-|b_{14}|^2\left( b_{23}^2-4 |b_{23}|^2+b_{23}^{*^2}\right) }\right) ,\\&\mu _2=2 \left( b_{11} b_{33}+b_{22} b_{44}+\sqrt{(b_{14} b_{23})^2+(b_{23}^* b_{14}^*)^2-|b_{14}|^2\left( b_{23}^2-4 |b_{23}|^2+b_{23}^{*^2}\right) }\right) ,\\&\mu _3=b_{11}^2+b_{22}^2+b_{33}^2+b_{44}^2-2 (|b_{23}|^2+|a_{14}|^2). \end{aligned} \end{aligned}$$

(29)